这是一份YORK约克大学MAT00001C作业代写的成功案例
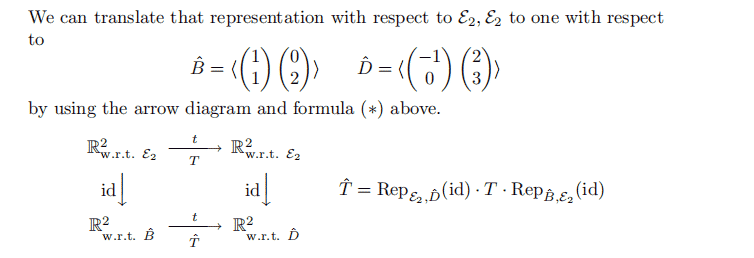
first box. Since the third box is formed from $x_{1}\left(\begin{array}{l}1 \ 3\end{array}\right)+x_{2}\left(\begin{array}{l}2 \ 1\end{array}\right)=\left(\begin{array}{l}6 \ 8\end{array}\right)$ and $\left(\begin{array}{l}2 \ 1\end{array}\right)$, and the determinant is unchanged by adding $x_{2}$ times the second column to the first column, the size of the third box equals that of the second. We have this.
$$
\left|\begin{array}{ll}
6 & 2 \
8 & 1
\end{array}\right|=\left|\begin{array}{ll}
x_{1} \cdot 1 & 2 \
x_{1} \cdot 3 & 1
\end{array}\right|=x_{1} \cdot\left|\begin{array}{ll}
1 & 2 \
3 & 1
\end{array}\right|
$$
Solving gives the value of one of the variables.
$$
x_{1}=\frac{\left|\begin{array}{ll}
6 & 2 \
8 & 1
\end{array}\right|}{\left|\begin{array}{ll}
1 & 2 \
3 & 1
\end{array}\right|}=\frac{-10}{-5}=2
$$
The theorem that generalizes this example, Cramer’s Rule, is: if $|A| \neq 0$ then the system $A \vec{x}=\vec{b}$ has the unique solution $x_{i}=\left|B_{i}\right| /|A|$ where the matrix $B_{i}$ is formed from $A$ by replacing column $i$ with the vector $\vec{b}$. Exercise 3 asks for a proof.
For instance, to solve this system for $x_{2}$
$$
\left(\begin{array}{ccc}
1 & 0 & 4 \
2 & 1 & -1 \
1 & 0 & 1
\end{array}\right)\left(\begin{array}{l}
x_{1} \
x_{2} \
x_{3}
\end{array}\right)=\left(\begin{array}{c}
2 \
1 \
-1
\end{array}\right)
$$

MAT00010C COURSE NOTES :
Recall the definitions of the complex number addition
$$
(a+b i)+(c+d i)=(a+c)+(b+d) i
$$
and multiplication.
$$
\begin{aligned}
(a+b i)(c+d i) &=a c+a d i+b c i+b d(-1) \
&=(a c-b d)+(a d+b c) i
\end{aligned}
$$