这是一份imperial帝国理工大学 MATH M2PM5作业代写的成功案例
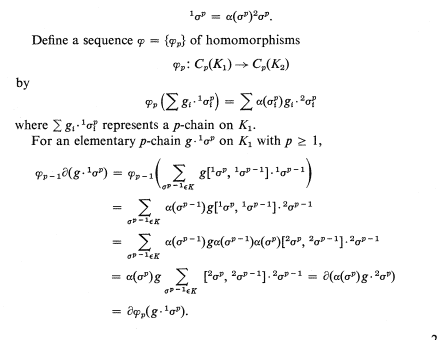
Observe that $B_{p}$ is spanned by the boundaries of elementary chains
$$
\partial\left(1 \cdot \sigma_{i}^{p+1}\right)=\sum \eta_{i j}(p) \cdot \sigma_{j}^{p}
$$
where $\left(\eta_{t j}(p)\right)=\eta(p)$ is the $p$ th incidence matrix. Thus $\operatorname{dim} B_{p}=\operatorname{rank} \eta(p)$.
Since the number of $d_{p+1}^{i}$ is the same as the number of $b_{p}^{i}$, then
$$
\operatorname{dim} D_{p+1}=\operatorname{dim} B_{p}=\operatorname{rank} \eta(p), \quad 0 \leq p \leq n-1 .
$$
Then
$$
\begin{aligned}
R_{p} &=\alpha_{p}-\operatorname{dim} D_{p}-\operatorname{dim} B_{p} \
&=\alpha_{p}-\operatorname{rank} \eta(p-1)-\operatorname{rank} \eta(p), \quad 1 \leq p \leq n-1 .
\end{aligned}
$$
Note also that
$$
\begin{aligned}
&R_{0}=\operatorname{dim} Z_{0}-\operatorname{dim} B_{0}=\alpha_{0}-\operatorname{rank} \eta(0) \
&R_{n}=\operatorname{dim} Z_{n}=\alpha_{n}-\operatorname{dim} D_{n}=\alpha_{n}-\operatorname{rank} \eta(n-1)
\end{aligned}
$$
In the alternating sum $\sum_{p=0}^{n}(-1)^{p} R_{p}$, all the terms rank $\eta(p)$ cancel, and we have
$$
\sum_{p=0}^{n}(-1)^{p} R_{p}=\sum_{p=0}^{n}(-1)^{p} \alpha_{p} .
$$

MATH M2PM5 COURSE NOTES :
It is left as an exercise for the reader to show that this orientation for the $n$-simplexes and $(n-1)$-simplexes gives
$$
\left[\sigma_{i}, \sigma_{i j}\right]=-\left[\sigma_{j}, \sigma_{i j}\right]
$$
in each case. It follows that any $n$-chain of the form $\sum_{\sigma_{i} \in R} g \cdot \sigma_{i}, g$ an integer, is an $n$-cycle. Furthermore, if
$$
z=\sum_{\sigma_{i} \in \mathbb{K}} g_{i} \cdot \sigma_{i}
$$
is an $n$-cycle, then
$$
0=\partial(z)=\sum_{\sigma_{i j} \in \mathcal{R}} h_{i f} \cdot \sigma_{i j}
$$