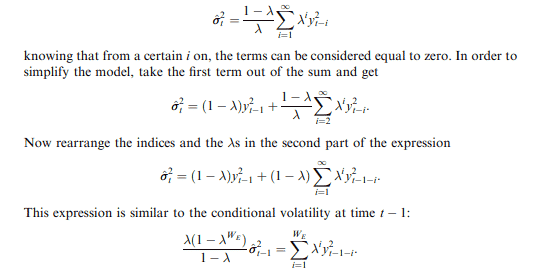

The first model designed to capture volatility clusters was ARCH
$$
\sigma_{l}^{2}=\omega+\sum_{i=1}^{L_{1}} \alpha_{i} Y_{l-i}^{2}
$$
where $L_{1}$ is the number of lags. ${ }^{2}$ Setting the lag to one in (2.3) will result in the ARCH(1) model which states that the conditional variance of today’s return is equal to a constant, plus yesterday’s return squared; that is:
$$
\sigma_{t}^{2}=\omega+\alpha Y_{l-1}^{2} .
$$
The moments of any order $m$ are given by:
$$
\mathrm{E}\left(Y^{m}\right)=\mathrm{E}\left(\mathrm{E}{t}\left(Y^{m}\right)\right)=\mathrm{E}\left(Y{t}^{m}\right)
$$
for all $t$. Therefore:
$$
\mathrm{E}\left(Y^{2}\right)=\sigma^{2}=\mathrm{E}\left(Y_{t}^{2}\right)=\mathrm{E}\left(\sigma_{t}^{2} Z_{t}^{2}\right)=\mathrm{E}\left(\sigma_{t}^{2}\right)
$$
Then
$$
\sigma^{2}=\mathrm{E}\left(\omega+\alpha Y_{t-1}^{2}\right)=\omega+\alpha \sigma^{2} .
$$
So, the unconditional volatility of the $\mathrm{ARCH}(1)$ model is given by:
$$
\sigma^{2}=\frac{\omega}{1-\alpha}
$$
PHAS00038 COURSE NOTES :
We assume initially that we hold one unit of the asset (i.e., the current portfolio value is $P_{t}$ ). We then derive the VaR for simple returns from Definition 1.2:
$$
R_{t}=\frac{P_{t}-P_{t-1}}{P_{t-1}}
$$
where-following the discussion in Section $5.4$-we assume mean return is zero. Volatility is indicated by $\sigma$. Let us start with the definition of VaR from (4.1):
$$
\operatorname{Pr}\left[Q_{t} \leq-\operatorname{VaR}(p)\right]=p .
$$
VaR is then obtained from:
$$
\begin{aligned}
p &=\operatorname{Pr}\left(P_{t}-P_{t-1} \leq-\operatorname{VaR}(p)\right) \
&=\operatorname{Pr}\left(P_{t-1} R_{t} \leq-\operatorname{VaR}(p)\right) \
&=\operatorname{Pr}\left(\frac{R_{t}}{\sigma} \leq-\frac{\operatorname{VaR}(p)}{P_{t-1} \sigma}\right) .
\end{aligned}
$$
Let us denote the distribution of standardized returns $\left(R_{t} / \sigma\right)$ by $F_{R}(\cdot)$ and the inverse distribution by $F_{R}^{-1}(p)$. Then it follows that the VaR for holding one unit of the asset is:
$$
\operatorname{VaR}(p)=-\sigma F_{R}^{-1}(p) P_{t-1} .
$$