Assignment-daixieTM为您提供伦敦大学学院 London’s Global University MATH0051 Analysis 4: Real Analysis实分析代写代考和辅导服务!
Instructions:
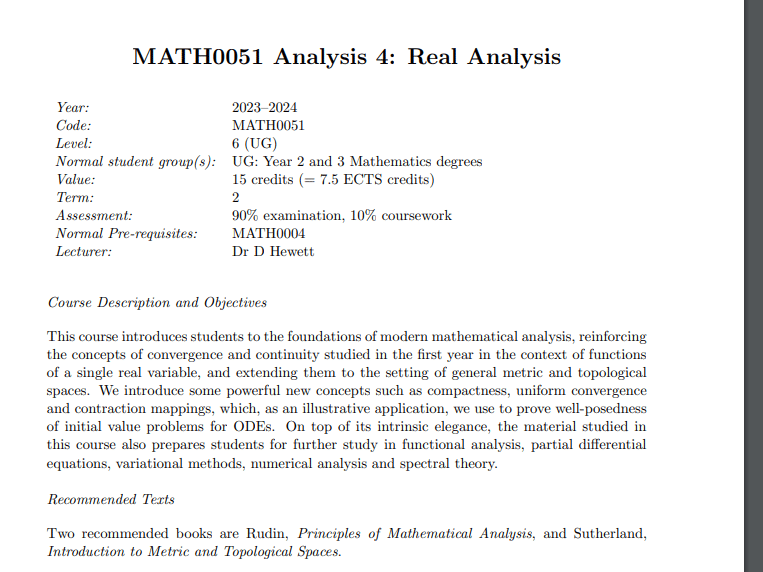
Let $R \in \mathbb{R}$, and let $f:[R, \infty) \rightarrow \mathbb{R}$. We say that $f(x)$ converges to $L$ as $x \rightarrow \infty$ if for every $\epsilon>0$ there exists $M \geq R$ such that for all $x \geq M$ we have $|f(x)-L|<\epsilon$. We write $f(x) \rightarrow L$ as $x \rightarrow \infty$ or $$ \lim _{x \rightarrow \infty} f(x)=L $$ [A similar definition can be formulated for limits as $x \rightarrow-\infty$ but we will not do so here.]
(a) Prove that $$ \lim _{x \rightarrow \infty} \frac{x^2}{x^2+1}=1 $$
(a) To prove that $\lim_{x \rightarrow \infty} \frac{x^2}{x^2+1} = 1$, we need to show that for every $\epsilon > 0$, there exists $M \geq R$ such that $|f(x)-1| < \epsilon$ for all $x \geq M$.
Let $\epsilon > 0$ be given. We want to find $M \geq R$ such that $\left| \frac{x^2}{x^2+1} – 1 \right| = \frac{1}{x^2+1} < \epsilon$ for all $x \geq M$. This is equivalent to $\frac{1}{\epsilon} – 1 < x^2+1$, or $x^2 > \frac{1-\epsilon}{\epsilon}$. So we can choose $M$ to be any real number greater than $\sqrt{\frac{1-\epsilon}{\epsilon}}$. Then for all $x \geq M$, we have $\left| \frac{x^2}{x^2+1} – 1 \right| < \epsilon$, which proves that $\lim_{x \rightarrow \infty} \frac{x^2}{x^2+1} = 1$.
(b) Prove that $$ \lim _{x \rightarrow \infty} \sin x $$ does not exist.
(b) To prove that $\lim_{x \rightarrow \infty} \sin x$ does not exist, we need to show that there exist two different values $L_1$ and $L_2$ such that for every $M \geq R$, there exist $x_1, x_2 \geq M$ such that $|\sin x_1 – L_1| \geq \epsilon$ and $|\sin x_2 – L_2| \geq \epsilon$ for some $\epsilon > 0$.
Let $L_1 = 1$ and $L_2 = -1$. Let $\epsilon = \frac{1}{2}$ be given. For any $M \geq R$, we can choose $x_1 = \left(2k+\frac{1}{4}\right)\pi$ and $x_2 = \left(2k+\frac{3}{4}\right)\pi$ for some integer $k$ such that $x_1, x_2 \geq M$. Then we have $\sin x_1 = 1$ and $\sin x_2 = -1$, so $|\sin x_1 – L_1| = |\sin x_2 – L_2| = 0.5 \geq \epsilon$. Therefore, $\lim_{x \rightarrow \infty} \sin x$ does not exist.
Let $S \subset \mathbb{R}$. We say that $f: S \rightarrow \mathbb{R}$ is Lipschitz continuous on $S$ if there exists $L \geq 0$ such that for all $x, y \in S$
$$
|f(x)-f(y)| \leq L|x-y|
$$
Prove that if $f: S \rightarrow \mathbb{R}$ is Lipschitz continuous on $S$ then $f$ is uniformly continuous on $S$.
To prove that $f$ is uniformly continuous on $S$, we need to show that for any $\epsilon>0$, there exists a $\delta>0$ such that for all $x,y \in S$ with $|x-y|<\delta$, we have $|f(x)-f(y)|<\epsilon$.
Since $f$ is Lipschitz continuous on $S$, we know that there exists $L\geq 0$ such that $|f(x)-f(y)|\leq L|x-y|$ for all $x,y\in S$.
Now, let $\epsilon>0$ be given. Choose $\delta=\frac{\epsilon}{L}$. Then, for any $x,y\in S$ with $|x-y|<\delta$, we have
$|f(x)-f(y)| \leq L|x-y|<L \cdot \frac{\epsilon}{L}=\epsilon$.
Thus, we have shown that for any $\epsilon>0$, there exists a $\delta>0$ such that for all $x,y\in S$ with $|x-y|<\delta$, we have $|f(x)-f(y)|<\epsilon$. Therefore, $f$ is uniformly continuous on $S$.
