Tag Archives: KCL代写

时间序列分析 Time Series Analysis  6CCM344A

0
Posted on by

这是一份UCL伦敦大学 6CCM344A作业代写的成功案例

时间序列分析 Time Series Analysis  6CCM344A
问题 1.

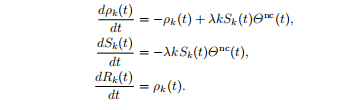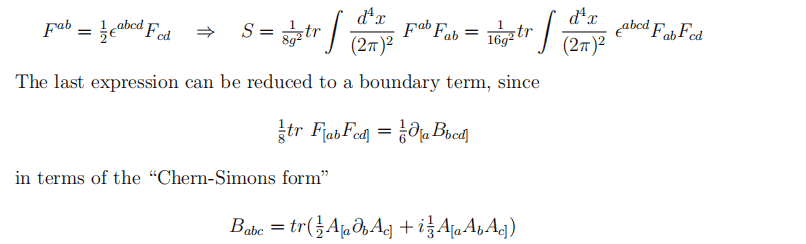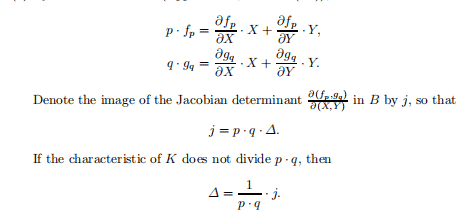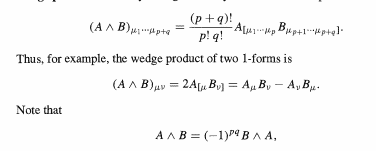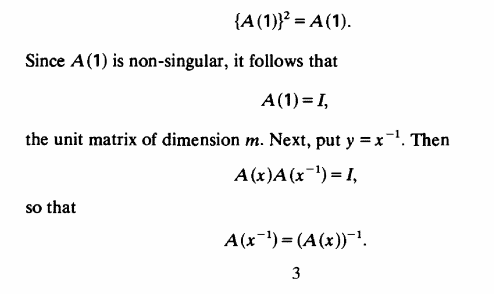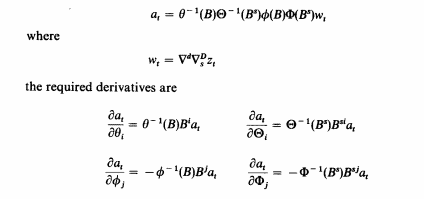
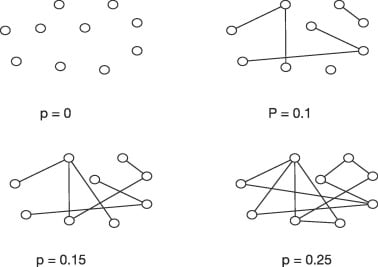
$$
\beta_{t}=\phi_{x}(B) \theta_{x}^{-1}(B) y_{t}
$$
then the model may be written
$$
\beta_{t}=v(B) \alpha_{t}+\varepsilon_{t}
$$
where $\varepsilon_{t}$ is the transformed noise series defined by
$$
\varepsilon_{t}=\phi_{x}(B) \theta_{x}^{-1}(B) n_{t}
$$


证明 .

$$
\gamma_{\alpha \beta}(k)=v_{k} \sigma_{\alpha}^{2}
$$
where $\gamma_{\alpha \beta}(k)=E\left[\alpha_{t-k} \beta_{t}\right]$ is the cross covariance at lag $+k$ between $\alpha$ and $\beta$. Thus
$$
v_{k}=\frac{\gamma_{\alpha \phi}(k)}{\sigma_{\alpha}^{2}}
$$

英国论文代写Viking Essay为您提供作业代写代考服务

 6CCM344A COURSE NOTES ：

$$
f_{1, t}=\delta_{1}^{-1}(B) \omega_{1}(B) x_{1, t-b_{1}}
$$
and for specified values of $b_{2}, \boldsymbol{\delta}{2}, \boldsymbol{\omega}{2}$,
$$
y_{2, t}=\delta_{2}^{-1}(B) \omega_{2}(B) x_{2, t-b_{2}}
$$
Then the noise $n_{t}$ can be calculated from
$$
n_{t}=y_{t}-y_{1, t}-y_{2, t}
$$
and finally, $a_{t}$ from
$$
a_{t}=\theta^{-1}(B) \phi(B) n_{t}
$$







复杂网络理论 Theory Of Complex Networks 6CCMCS02

0
Posted on by

这是一份UCL伦敦大学  6CCMCS02作业代写的成功案例

复杂网络理论 Theory Of Complex Networks 6CCMCS02
问题 1.

$$
S_{k}(t)=e^{-\lambda k \phi(t)}, \quad R_{k}(t)=\int_{0}^{\infty} \rho_{k}(\tau) d t
$$
where we have defined the auxiliary function
$$
\phi(t)=\int_{0}^{t} \Theta^{\mathrm{nc}}(\tau) d \tau=\frac{1}{\langle k\rangle} \sum_{k}(k-1) P(k) R_{k}(t)
$$


证明 .

In order to get a closed relation for the total density of infected individuals, it results more convenient to focus on the time evolution of the averaged magnitude $\phi(t)$. To this purpose, let us compute its time derivative:
$$
\frac{d \phi(t)}{d t}=1-\frac{1}{\langle k\rangle}-\phi(t)-\frac{1}{\langle k\rangle} \sum_{k}(k-1) P(k) e^{-\lambda k \phi(t)}
$$
where we have introduced the time dependence of $S_{k}(t)$ obtained in ( $[8.37)$. Once solved (В.39]), we can obtain the total epidemic prevalence $R_{\infty}$ as a function of $\phi_{\infty}=\lim {t \rightarrow \infty} \phi(t)$. Since $R{k}(\infty)=1-S_{k}(\infty)$, we have
$$
R_{\infty}=\sum_{k} P(k)\left(1-e^{-\lambda k \phi_{\infty}}\right) .
$$

英国论文代写Viking Essay为您提供作业代写代考服务

  6CCMCS02 COURSE NOTES ：

$$
P_{i j}^{k}(n)=\sum_{l_{1}, l_{2}, \ldots, l_{n-1}} p_{i l_{1}}^{k} p_{l_{1} l_{2}}^{k} \cdots p_{l_{n-1} j^{*}}^{k}
$$
This definition allows us to compute the average number of times, $b_{i j}^{k}$, that a packet generated at $i$ and with destination at $k$ passes through $j$.
$$
b^{k}=\sum_{n=1}^{\infty} P^{k}(n)=\sum_{n=1}^{\infty}\left(p^{k}\right)^{n}=\left(I-p^{k}\right)^{-1} p^{k}
$$
and the effective betweenness of node $j, B_{j}$, is then defined as the sum over all possible origins and destinations of the packets,
$$
B_{j}=\sum_{i, k} b_{i j}^{k}
$$







超对称性和共形场理论 Supersymmetry and Conformal Field Theory 7CCMMS40

0
Posted on by

这是一份UCL伦敦大学 7CCMMS40作业代写的成功案例

超对称性和共形场理论 Supersymmetry and Conformal Field Theory 7CCMMS40
问题 1.

Self-duality then follows from requiring a certain coordinate dependence of the $U$ ‘s: This is fixed by giving the explicit dependence of the $v$ ‘s as
$$
v_{I i \alpha}=b_{I i \mathcal{A}} z_{\alpha}^{\mathcal{A}}, \quad v_{i^{\prime} \alpha}^{I}=b_{i^{\prime} \mathcal{A}}^{I} z^{\mathcal{A}}{ }{\alpha} $$ where the $b$ ‘s are constants. The orthonormality conditions on the $U$ ‘s then implies the constraint on the $b$ ‘s $$ b^{I}{ }{i^{\prime}(\mathcal{A}} b_{I i \mathcal{B})}=0
$$


证明 .

as well as determining the $u$ ‘s in terms of the $b$ ‘s (with much messier dependence than the $v$ ‘s), and thus $A$. Note that the $z$ dependence of $u$ can be written in terms of just $x$, as follows from rewriting the $u v$ orthogonality as (after multiplying by $z$ )
$$
u^{I}{ }{\iota} b{I i, \mathcal{A}} y^{\mathcal{A B}}=u_{I}{ }^{\iota} b^{I}{ }{i^{\prime} \mathcal{A}} y^{\mathcal{A B}}=0 $$ and noting scale invariance. Then the $x$ components of $A$ can also be written in terms of just $x$. We then can check the self-duality condition by calculating $f$ : The orthonormality condition on the $U$ ‘s can be written as $$ \delta{I}^{J}=u_{I}{ }^{\iota} u_{{ }^{J}}{ }^{J}+v_{I i}{ }^{\alpha} g^{i i^{\prime}} v_{i^{\prime} \alpha}^{J}
$$

英国论文代写Viking Essay为您提供作业代写代考服务

 7CCMMS40 COURSE NOTES ：

$$
-\varphi-r^{2} \mathcal{A} \varphi=2 \mathcal{A}+r \mathcal{A}^{\prime}, \quad-r \varphi^{\prime}+r^{2} \mathcal{A} \varphi=-r \mathcal{A}^{\prime}+r^{2} \mathcal{A}^{2}
$$
After some massaging, we find the change of variables
$$
\tilde{\varphi}=\frac{1}{r}+r \varphi, \quad \tilde{\mathcal{A}}=\frac{1}{r}+r \mathcal{A}
$$
leads to the simplification
$$
\tilde{\varphi}^{\prime}=-\tilde{\mathcal{A}}^{2}, \quad \tilde{\mathcal{A}}^{\prime}=-\tilde{\mathcal{A}} \tilde{\varphi}
$$
$\tilde{\varphi}$ then can be eliminated, giving an equation for $\tilde{\mathcal{A}}$. Making a final change of variables,
$$
\psi=\tilde{\mathcal{A}}^{-1} \quad \Rightarrow \quad \psi \psi^{\prime \prime}-\left(\psi^{\prime}\right)^{2}=-1
$$







代数几何学 Algebraic Geometry MATH0076

0
Posted on by

这是一份UCL伦敦大学 MATH0076作业代写的成功案例

代数几何学 Algebraic Geometry MATH0076
问题 1.


$$
\operatorname{dim}{K} \frac{\overline{\mathcal{O}}{F, P}}{\mathcal{O}{F, P}}+\operatorname{dim}{K} \frac{\mathcal{O}{F, P}}{(\gamma)}=\operatorname{dim}{K} \frac{\overline{\mathcal{O}}{F, P}}{(\gamma)}+\operatorname{dim}{K} \frac{\gamma \overline{\mathcal{O}}{F, P}}{\gamma \mathcal{O}{F, P}} .
$$
Since $\gamma$ is not a zero divisor of $\overline{\mathcal{O}}{F, P}$, the $K$-vector spaces $\overline{\mathcal{O}}{F, P} / \mathcal{O}{F, P}$ and $\gamma \overline{\mathcal{O}}{F, P} / \gamma \mathcal{O}_{F, P}$ are isomorphic, and so


证明 .

$$
\mu_{P}(F, G)=\operatorname{dim}{K} \overline{\mathcal{O}}{F, P} /(\gamma) .
$$
As in the proof of we have
$$
\overline{\mathcal{O}}{F, P} /(\gamma) \cong R{1} /(\gamma) \times \cdots \times R_{h} /(\gamma)
$$
and then from E.13,
$$
\operatorname{dim}{K} \overline{\mathcal{O}}{F, P} /(\gamma)=\sum_{i=1}^{h} \operatorname{dim}{K}\left(R{i} /(\gamma)\right)=\sum_{i=1}^{h} \nu_{R_{i}}(\gamma)
$$

英国论文代写Viking Essay为您提供作业代写代考服务

MATH0076 COURSE NOTES ：



$$
\varepsilon: S\left[X_{1}, X_{2}\right] \rightarrow A[X, Y] \quad\left(X_{1} \mapsto X, X_{2} \mapsto Y\right)
$$
and
$$
\delta: S\left[X_{1}, X_{2}\right] \rightarrow B[X, Y] \quad\left(X_{1} \mapsto X, X_{2} \mapsto Y\right) .
$$
Here $\varepsilon(F)=f, \varepsilon(G)=g, \delta(F)=G f, \delta(G)=G g$, so that by $\varepsilon$ the induced epimorphism
$$
S\left[X_{1}, X_{2}\right] /(F, G) \rightarrow A[X, Y] /(f, g)
$$
can be identified with the canonical epimorphism $S^{e} \rightarrow A^{e}$ and
$$
S\left[X_{1}, X_{2}\right] /(F, G) \rightarrow B[X, Y] /(G f, G g)
$$
with $S^{e} \rightarrow B^{e}$.






李群和李代尔矩阵 Lie Groups and Lie Algebras MATH0075

0
Posted on by

这是一份UCL伦敦大学 MATH0075作业代写的成功案例

李群和李代尔矩阵 Lie Groups and Lie Algebras MATH0075
问题 1.


$$
H=\left(\begin{array}{cc}
1 & 0 \
0 & -1
\end{array}\right)
$$
in the Lie algebra sl $(2 ; \mathbb{C})$. Applying formula gives
$$
\left(\pi_{m}(H) f\right)(z)=-\frac{\partial f}{\partial z_{1}} z_{1}+\frac{\partial f}{\partial z_{2}} z_{2} .
$$


证明 .

Thus, we see that
$$
\pi_{m}(H)=-z_{1} \frac{\partial}{\partial z_{1}}+z_{2} \frac{\partial}{\partial z_{2}} .
$$
Applying $\pi_{m}(H)$ to a basis element $z_{1}^{k} z_{2}^{m-k}$, we get

英国论文代写Viking Essay为您提供作业代写代考服务

MATH0075 COURSE NOTES ：



We will use the following basis for $\operatorname{sl}(2 ; \mathbb{C})$ :
$$
H=\left(\begin{array}{cc}
1 & 0 \
0 & -1
\end{array}\right) ; X=\left(\begin{array}{ll}
0 & 1 \
0 & 0
\end{array}\right) ; Y=\left(\begin{array}{ll}
0 & 0 \
1 & 0
\end{array}\right)
$$
which have the commutation relations
$$
\begin{aligned}
&{[H, X]=2 X} \
&{[H, Y]=-2 Y} \
&{[X, Y]=H}
\end{aligned}
$$






黑洞和宇宙的时空结构Space-time Structure of Black Holes and the Universe 7CCMMS38

0
Posted on by

这是一份KCL伦敦大学 7CCMMS38作业代写的成功案例

黑洞和宇宙的时空结构Space-time Structure of Black Holes and the Universe 7CCMMS38
问题 1.


$$
d s^{2}=-(1+2 \Phi) d t^{2}+(1-2 \Phi)\left(d x^{2}+d y^{2}+d z^{2}\right)
$$
where $\Phi$ is the Newtonian potential $(-1 \ll \Phi<0)$. Consider a nearly Newtonian perfect fluid [stress-energy tensor
$$
T^{\alpha \beta}=(\rho+p) u^{\alpha} u^{\beta}+p g^{\alpha \beta}, \quad p \ll \rho ;
$$


证明 .

$$
v^{j} \equiv d x^{j} / d t \ll 1
$$
Show that the equations $T_{; \nu}^{\mu \nu}=0$ for this system reduce to the familiar Newtonian law of mass conservation, and the Newtonian equation of motion for a fluid in a gravitational field:
$$
\frac{d \rho}{d t}=-\rho \frac{\partial v^{j}}{\partial x^{j}}, \quad \rho \frac{d v^{j}}{d t}=-\rho \frac{\partial \Phi}{\partial x^{j}}-\frac{\partial p}{\partial x^{j}},
$$

英国论文代写Viking Essay为您提供作业代写代考服务

 7CCMMS38 COURSE NOTES ：



Demand that the intervals $a \mathscr{B}$ and $\mathscr{P} \mathscr{Q}$ be sufficiently small compared to the scale of curvature of spacetime; or specifically,
$$
R^{(A B)}(\mathscr{G B})^{2} \ll 1 / N
$$
and
$$
R^{(P Q)}(\mathscr{P} \mathcal{Q})^{2} \ll 1 / N,
$$
where $R^{(A B)}$ and $R^{(P Q)}$ are the largest relevant components of the curvature tensor in the two regions in question.
Demand that the time scale, $\tau_{0}$, of the geodesic clocks employed be small compared to $\mathscr{B} B$ and $\mathscr{P} \mathcal{Q}$ individually; thus,
$$
\begin{aligned}
&\tau_{0} \ll \mathscr{Q} \mathscr{B} / N, \
&\tau_{0} \ll \mathscr{P} \mathscr{Q} / N
\end{aligned}
$$






时空几何和广义相对论|Space Time Geometry and General Relativity  6CCM334A

0
Posted on by

这是一份KCL伦敦大学  6CCM334A作业代写的成功案例

空几何和广义相对论|Space Time Geometry and General Relativity  6CCM334A
问题 1.


$$
T(X, Y)=\nabla_{X} Y-\nabla_{Y} X-[X, Y],
$$
and thinking of the Riemann tensor as a map from three vector fields to a fourth one, we have (in funny-looking but standard notation)
$$
R(X, Y) Z=\nabla_{X} \nabla_{Y} Z-\nabla_{Y} \nabla_{X} Z-\nabla_{[X, Y]} Z .
$$


证明 .

In these expressions, the notation $\nabla_{X}$ refers to the covariant derivative along the vector field $X$; in components, $\nabla_{X}=X^{\mu} \nabla_{\mu}$. So, for example, (3.116) is equivalent to
$$
\begin{aligned}
R_{\sigma_{\mu \nu}} X^{\mu} Y^{v} Z^{\sigma}=& X^{\lambda} \nabla_{\lambda}\left(Y^{n} \nabla_{\eta} Z^{\rho}\right)-Y^{\lambda} \nabla_{\lambda}\left(X^{\eta} \nabla_{\eta} Z^{\rho}\right) \
&-\left(X^{\lambda} \partial_{\lambda} Y^{\eta}-Y^{\lambda} \partial_{\lambda} X^{\eta}\right) \nabla_{\eta} Z^{\rho}
\end{aligned}
$$

英国论文代写Viking Essay为您提供作业代写代考服务

 6CCM334A COURSE NOTES ：



$$
p^{\lambda} \nabla_{\lambda} p^{\mu}=0
$$
By metric compatibility we are free to lower the index $\mu$, and then we may expand the covariant derivative to obtain
$$
p^{\lambda} \partial_{\lambda} p_{\mu}-\Gamma_{\lambda \mu}^{\sigma} p^{\lambda} p_{\sigma}=0 .
$$
The first term tells us how the momentum components change along the path,
$$
p^{\lambda} \partial_{\lambda} p_{\mu}=m \frac{d x^{\lambda}}{d \tau} \partial_{\lambda} p_{\mu}=m \frac{d p_{\mu}}{d \tau}
$$






序列和系列|Sequences And Series 4CCM115A

0
Posted on by

这是一份KCL伦敦大学 4CCM115A作业代写的成功案例

圆环与模块|Rings And Modules  6CCM350A
问题 1.


We shall use the notation $\equiv$ for equivalence, as in $\S 1.6$.
(i) Reflexivity. For all $a, b \in \mathbf{Z}, a b=b a$ and so $\frac{a}{b} \equiv \frac{a}{b}$.
(ii) Symmetry. Suppose $\frac{a}{b} \equiv \frac{c}{d}$. Then $a d=b c$, and so, by commutativity for the integers, $c b=d a$, showing that $\frac{c}{d} \equiv \frac{a}{b}$.


证明 .

(iii) Transitivity. Suppose $\frac{a}{b} \equiv \frac{c}{d}$ and $\frac{c}{d} \equiv \frac{e}{f}$. Then $a d=b c$ and $c f=$ de. Multiplying both left-hand sides and both right-hand sides together gives adcf $=b c d e$. This factorizes to give $(a f-b e) c d=0$. Now $b, d, f$ are non-zero, being denominators. Since $a d=b c$ and $c f=$ de we conclude that if $c=0$ then $a=0$ and $e=0$, giving $a f=b e$. If $c \neq 0$ then since $d \neq 0,(a f-b e) c d=0$ implies that $a f-b e=0$, i.e. $a f=b e$. This shows that $\frac{a}{b} \equiv \frac{e}{f^{\prime}}$, establishing transitivity.

英国论文代写Viking Essay为您提供作业代写代考服务

4CCM115A COURSE NOTES ：



(i) From the calculation of the first four convergents earlier in this section the result can be verified for small values of $n$. (The algebra is fairly involved for $n=3$ and $n=4$.) For $n=1$ the conventions introduced in Proposition 3 tell us that
$$
p_{1} q_{0}-q_{1} p_{0}=a_{1} \times 0-1 \times 1=-1=(-1)^{1},
$$
so the equation is satisfied for $n=1$.
Suppose that the equation is valid for $n-1$. Using the recurrence relations of proposition 3 gives
$$
\begin{aligned}
p_{n} q_{n-1}-q_{n} p_{n-1} &=\left(a_{n} p_{n-1}+p_{n-2}\right) q_{n-1}-\left(a_{n} q_{n-1}+q_{n-2}\right) p_{n-1} \
&=-\left(p_{n-1} q_{n-2}-q_{n-1} p_{n-2}\right)=(-1)^{n}
\end{aligned}
$$






圆环与模块|Rings And Modules  6CCM350A

0
Posted on by

这是一份KCL伦敦大学  6CCM350A作业代写的成功案例

圆环与模块|Rings And Modules  6CCM350A
问题 1.


Proof. (i) It is most convenient here to think in module terms. We have $V=V_{1} \oplus \cdots \oplus V_{s}$ as $\mathbf{k}[x]$-module, where $V_{1}$ is cyclic of order $d_{i}$. As $d_{i} \mid d_{s}$, we have $d_{s} V_{i}={0}$ for $1 \leqslant i \leqslant s$, and so $d_{s} V={0}$. Therefore $d_{s}(\alpha)=0$ and $g \mid d_{s}$, where $g=\min \alpha$. On the other hand, $g(\alpha)=0$, and so ${0}=g(\alpha) V_{s}=g V_{s^{*}}$. Hence $d_{s} \mid g$. Therefore $d_{s} \sim g$, and since $d_{s}$ and $g$ are both monic, this gives $d_{s}=g$.


证明 .

We prove by induction on $r$ that $\operatorname{ch} C(d)=d$. If $r=1$, then the above determinant is equal to $x+a_{0}$ as stated. If $r>1$, we expand by the top row and obtain
$$
\begin{aligned}
\operatorname{ch} C\left(a_{0}+a_{1} x+\cdots+a_{r-1} x^{r-1}+x^{r}\right) \
&=x \operatorname{ch} C\left(a_{1}+a_{2} x+\cdots+a_{r-1} x^{r-2}+x^{r-1}\right)+a_{0}
\end{aligned}
$$
whence the result follows by induction.
We therefore obtain $\operatorname{ch} \alpha=\operatorname{ch} A=\operatorname{ch} C\left(d_{1}\right) \ldots \operatorname{ch} C\left(d_{s}\right)=d_{1} \ldots d_{s}$, as stated.

英国论文代写Viking Essay为您提供作业代写代考服务

6CCM350A COURSE NOTES ：


Proof. We notice first that a gencral element $f \in F$ has the form $f=\sum_{i=1}^{t} g_{i}(x) f_{i}$ with $g_{i}(x) \in \mathbf{k}[x]$, and that the effect of $\epsilon$ on such an element is given by
$$
\epsilon\left(\sum g_{i}(x) f_{i}\right)=\sum g_{i}(x) v_{l}=\sum g_{i}(\alpha)\left(v_{l}\right) .
$$
Therefore $\epsilon\left(n_{j}\right)=\alpha\left(v_{l}\right)-\sum a_{j i} v_{j}=0$, since $A=M(\alpha, v)$. Hence each $n_{i}$ belongs to $N$.

We now show that n generates $N$. To see this, let $N^{}$ be the submodule $\sum_{i=1}^{t} \mathbf{k}[x] n_{i}$ generated by $\mathbf{n}$. Then $$ N^{} \subseteq N \text {. }
$$






有限群的代表理论|Representation Theory Of Finite Groups  6CCM351A

0
Posted on by

这是一份KCL伦敦大学 6CCM351A作业代写的成功案例

有限群的代表理论|Representation Theory Of Finite Groups  6CCM351A
问题 1.


Show that $U$ and $W$ are subspaces of $V$ which are invariant under $\varepsilon$, that is $U \varepsilon \subset U, W \varepsilon \subset W$.
Prove that
$$
V=U \oplus W
$$


证明 .

Deduce that if $E$ is an $m \times m$ matrix such that
$$
E^{2}=E, \quad E \neq 0, \quad E \neq I,
$$
there exists an integer $r$ satisf ying $1 \leqslant r<m$ and a non-singular matrix $T$ such that
$$
T^{-1} E T=\left(\begin{array}{ll}
I_{r} & 0 \
0 & 0
\end{array}\right)=J
$$

英国论文代写Viking Essay为您提供作业代写代考服务

6CCM351A COURSE NOTES ：


Next we construct the matrix
$$
C=C(\Xi)=\sum_{y \in G} B\left(y^{-1}\right) \Xi A(y) .
$$
Let $x$ be a fixed element of $G$; then $z=y x$ ranges over $G$ when $y$ does. Thus we may equally well write
$$
C=\sum_{z} B\left(z^{-1}\right) \Xi A(z)=\sum_{y} B\left(x^{-1} y^{-1}\right) \Xi A(y x) .
$$
Since $A$ and $B$ are representations, we obtain that
$$
C=B\left(x^{-1}\right)\left(\sum_{y} B\left(y^{-1}\right) \Xi A(y)\right) A(x),
$$
or
$$
B(x) C=C A(x) \quad(x \in G),
$$