这是一份BATH巴斯大学MA10210作业代写的成功案例
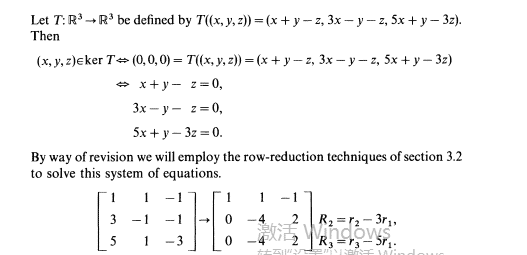

An equivalent system is, therefore,
$$
\begin{aligned}
&x+y-z=0 \
&-4 y+2 z=0
\end{aligned}
$$
This has infinitely many solutions: $2 y=z, x=z-y=2 y-y=y$. Hence, $\operatorname{ker} T={(y, y, 2 y): y \in \mathbb{R}}$
$$
={y(1,1,2): y \in \mathbb{R}}=\langle(1,1,2)\rangle,
$$
and nullity $T=1$. Now $T((1,0,0))=(1,3,5), T((0,1,0))=(1,-1,1)$, $T((0,0,1))=(-1,-1,-3)$. But $(1,0,0),(0,1,0),(0,0,1)$ is a basis for $\mathbb{R}^{3}$, so $(1,3,5),(1,-1,1),(-1,-1,-3)$ is a spanning sequence for im $T$, by Theorem 6.4.3. These vectors must be linearly dependent, since, by the dimension theorem,c

MA10210 COURSE NOTES :
to above is $T: \mathbb{R}^{3} \rightarrow \mathbb{R}^{3}$ given by
$$
T((x, y, z))=(x-y+2 z, 2 x+y-z, x-4 y+7 z)
$$
Now $T((1,0,0))=(1,2,1), \quad T((0,1,0))=(-1,1,-4), \quad T((0,0,1))=(2,-1,7)$ span im $T$, by Theorem $6.4 .3$. However,
$$
(1,2,1)=5(-1,1,-4)+3(2,-1,7),
$$
so these three vectors are linearly dependent. Since $(1,2,1),(-1,1,-4)$ are linearly independent, these two vectors form a basis for im $T$. Hence $\operatorname{rank} A=\operatorname{rank} T=2$.