Assignment-daixieTM为您提供芝加哥大学University of ChicagoMATH 23900Topics in Analysis拓扑学代写代考和辅导服务!
Instructions:
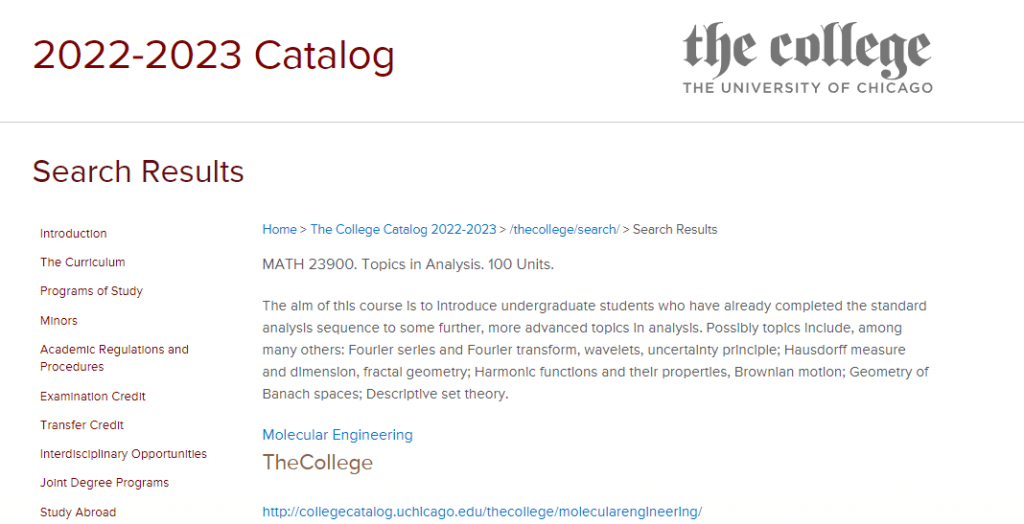
Each of these topics is a rich area of study in its own right, and together they give a sense of the breadth and depth of modern analysis.
Fourier analysis is a cornerstone of many fields in mathematics and science, and the Fourier series and transform are powerful tools for understanding periodic functions and signals. Wavelets are a more recent development that allow for the analysis of non-periodic functions with local properties.
The concept of dimension and measures of sets is a central theme in geometric measure theory, which aims to understand the structure of sets in Euclidean space and beyond. Fractal geometry is a fascinating subject that seeks to understand the structure of irregular or fragmented shapes.
Harmonic functions arise in many areas of analysis, including potential theory, partial differential equations, and probability theory. Brownian motion is a fundamental process in probability theory that describes the random motion of particles.
Banach spaces are important in functional analysis, which is the study of spaces of functions and operators between them. They have applications in many areas of mathematics and physics, including quantum mechanics.
Finally, descriptive set theory is concerned with the study of sets and functions on topological spaces, and has applications in many areas of mathematics, including logic, topology, and analysis.
Overall, this course seems like an excellent opportunity for students to deepen their understanding of analysis and to explore some fascinating topics in more depth.
An order of a number field $K$ is a subring (with 1 ) of $\mathcal{O}_K$ which is free of rank $[K: \mathbb{Q}]$ as a $\mathbb{Z}$-module. Describe (with proof) all the orders of a quadratic field $\mathbb{Q}(\sqrt{d})$.
Let $K=\mathbb{Q}(\sqrt{d})$ be a quadratic field with discriminant $D=4d$ if $d\equiv 2$ or $3$ mod $4$, and $D=d$ if $d\equiv 1$ mod $4$. The ring of integers of $K$ is given by
\mathcal{O}_K= \begin{cases}\mathbb{Z}\left[\frac{1+\sqrt{d}}{2}\right], & \text { if } d \equiv 2,3(\bmod 4) \ \mathbb{Z}[\sqrt{d}], & \text { if } d \equiv 1(\bmod 4)\end{cases}
An order $\mathcal{O}$ of $K$ is a subring of $\mathcal{O}_K$ that is free of rank $[K:\mathbb{Q}]$ as a $\mathbb{Z}$-module. Thus, if $\mathcal{O}$ is an order of $K$, then $\mathcal{O}=\mathbb{Z}a_1+\mathbb{Z}a_2$ for some $a_1,a_2\in\mathcal{O}_K$.
We first consider the case when $d\equiv 2,3\pmod{4}$, so that $\mathcal{O}_K=\mathbb{Z}\left[\frac{1+\sqrt{d}}{2}\right]$. Let $\alpha=\frac{1+\sqrt{d}}{2}$, so that $\mathcal{O}_K=\mathbb{Z}[\alpha]$. Then any element of $\mathcal{O}$ can be written as $r\alpha+s$ for some $r,s\in\mathbb{Z}$. We note that $r$ and $s$ must be both even or both odd, since $\mathcal{O}$ is closed under addition and multiplication. Thus, $\mathcal{O}=\mathbb{Z}[2\alpha]+\mathbb{Z}[2]$ or $\mathcal{O}=\mathbb{Z}[\alpha]+\mathbb{Z}[2]$.
Next, we consider the case when $d\equiv 1\pmod{4}$, so that $\mathcal{O}_K=\mathbb{Z}[\sqrt{d}]$. Let $\alpha=\sqrt{d}$, so that $\mathcal{O}_K=\mathbb{Z}[\alpha]$. Then any element of $\mathcal{O}$ can be written as $r\alpha+s$ for some $r,s\in\mathbb{Z}$. We note that $r$ and $s$ must have the same parity, since $\mathcal{O}$ is closed under addition and multiplication. Thus, $\mathcal{O}=\mathbb{Z}[2\alpha]+\mathbb{Z}[2]$ or $\mathcal{O}=\mathbb{Z}[\alpha]+\mathbb{Z}[2\alpha]$.
In summary, the orders of $K=\mathbb{Q}(\sqrt{d})$ are as follows: \begin{align*} &\mathcal{O}=\mathbb{Z}[2\alpha]+\mathbb{Z}[2],\quad d\equiv 2,3\pmod{4},\ &\mathcal{O}=\mathbb{Z}[\alpha]+\mathbb{Z}[2],\quad d\equiv 2,3\
Let $m>1$ be a squarefree composite integer. Show that $\mathbb{Z}[\sqrt{-m}]$ is not a PID.
Assume, for the sake of contradiction, that $\mathbb{Z}[\sqrt{-m}]$ is a principal ideal domain (PID).
Let $a+b\sqrt{-m}\in\mathbb{Z}[\sqrt{-m}]$ be a non-zero element, and let $d$ be a generator of the ideal $(a+b\sqrt{-m})$. Then, we have $(a+b\sqrt{-m})=(d)$, which implies $a+b\sqrt{-m}=d\cdot(c+d\sqrt{-m})$ for some $c+d\sqrt{-m}\in\mathbb{Z}[\sqrt{-m}]$.
Taking norms on both sides, we get $N(a+b\sqrt{-m})=N(d)\cdot N(c+d\sqrt{-m})$, where $N(x)=x\cdot\overline{x}=a^2+mb^2$ is the norm of $x=a+b\sqrt{-m}$.
Since $m$ is squarefree and greater than $1$, it follows that $m$ is odd and $a^2\equiv b^2\pmod{m}$. Therefore, $N(a+b\sqrt{-m})=a^2+mb^2\equiv a^2-b^2\pmod{m}$.
If $d$ is a unit, then $(a+b\sqrt{-m})=\mathbb{Z}[\sqrt{-m}]$, which implies $N(a+b\sqrt{-m})=1$, a contradiction. Thus, $d$ must be a non-unit. Since $d$ generates the ideal $(a+b\sqrt{-m})$, it follows that $N(d)$ divides $N(a+b\sqrt{-m})$.
However, since $a^2\equiv b^2\pmod{m}$, we have $a^2-b^2=(a-b)(a+b)\equiv 0\pmod{m}$, and since $m$ is squarefree, it follows that $a+b\sqrt{-m}$ and $a-b\sqrt{-m}$ are relatively prime.
Therefore, we have $N(d)\mid N(a+b\sqrt{-m})$ and $N(d)\mid N(a-b\sqrt{-m})$, which implies $N(d)\mid N((a+b\sqrt{-m})(a-b\sqrt{-m}))=N(m)$. However, since $m$ is composite and greater than $1$, its norm $N(m)=m^2$ is not a prime power, and hence $N(d)$ must be one of the divisors of $m$.
Since $d$ generates the ideal $(a+b\sqrt{-m})$, it follows that $(a+b\sqrt{-m})=(d)$ is one of the prime ideals in the factorization of $(m)$. However, since $m$ is composite and $m\mid N(a+b\sqrt{-m})$, it follows that $(a+b\sqrt{-m})$ is not a prime ideal, a contradiction.
Therefore, $\mathbb{Z}[\sqrt{-m}]$ is not a PID.
Let $A$ be a Dedekind domain which has a unique nonzero maximal ideal. Show that $A$ is a PID.
Let $M$ be the unique nonzero maximal ideal of $A$. We will show that any nonzero ideal $I$ of $A$ is principal.
Since $A$ is a Dedekind domain, any nonzero ideal $I$ of $A$ can be written uniquely as a product of prime ideals: $I = \mathfrak{p}_1^{e_1} \cdots \mathfrak{p}_k^{e_k}$, where $\mathfrak{p}_1, \ldots, \mathfrak{p}_k$ are distinct prime ideals of $A$ and $e_i \geq 1$ for all $i$. Since $M$ is the unique nonzero maximal ideal of $A$, it follows that $M$ is a prime ideal.
If $I = M^r$ for some $r \geq 1$, then $I$ is a principal ideal generated by $m^r$ for any nonzero element $m \in M$. So suppose that $I \neq M^r$ for all $r \geq 1$. Then $I$ contains a prime ideal $\mathfrak{p}$ which is not equal to $M$. Since $\mathfrak{p}$ is a prime ideal, it follows that $M\mathfrak{p}$ is contained in $I$. Moreover, $M\mathfrak{p}$ is a proper ideal of $A$, since $A/\mathfrak{p}$ is a domain and $A/M$ is not.
By unique factorization of ideals, we have $M\mathfrak{p} = M^{e}\mathfrak{q}_1 \cdots \mathfrak{q}_l$ for some $e \geq 1$, where $\mathfrak{q}_1, \ldots, \mathfrak{q}_l$ are distinct prime ideals of $A$ that are not equal to $M$. Since $M$ is the unique maximal ideal of $A$, it follows that $e = 1$, and hence $M\mathfrak{p} = \mathfrak{q}_1 \cdots \mathfrak{q}_l$.
Since $\mathfrak{p}$ is a prime ideal and $\mathfrak{p} \neq M$, it follows that $\mathfrak{p}$ is contained in a maximal ideal $N$ of $A$ that is not equal to $M$. Since $A$ has a unique nonzero maximal ideal, it follows that $N = \mathfrak{q}$ for some prime ideal $\mathfrak{q}$ of $A$ that is not equal to $M$. Since $\mathfrak{q}$ is a prime ideal and $M\mathfrak{p} = \mathfrak{q}_1 \cdots \mathfrak{q}_l$, it follows that $\mathfrak{q}$ is equal to one of the prime ideals $\mathfrak{q}_1, \ldots, \mathfrak{q}_l$. Without loss of generality, we may assume that $\mathfrak{q} = \mathfrak{q}_1$.
We claim that $\mathfrak{p}$ is not contained in $\mathfrak{q}_2 \cdots \mathfrak{q}_l$. Suppose to the contrary that $\mathfrak{p}$ is contained in $\mathfrak{q}_2 \cdots \mathfrak{q}_l$. Then $M\mathfrak{p}$
