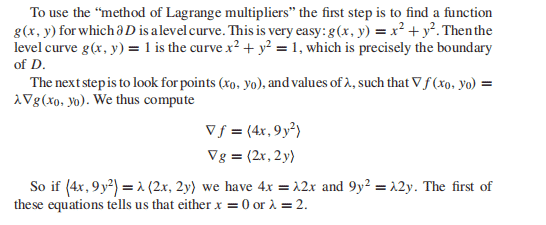
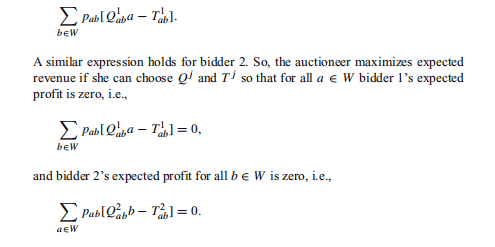
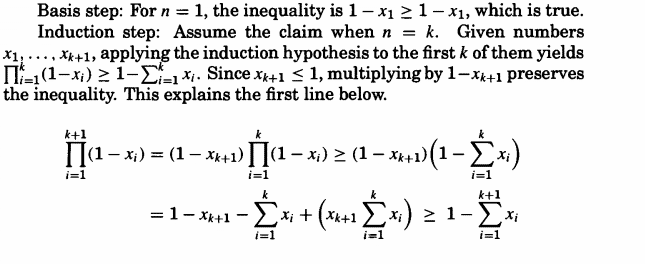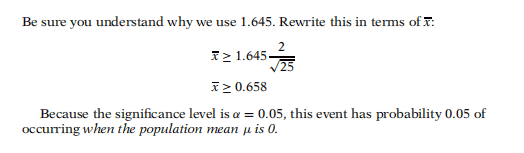这是一份ed.ac.uk爱丁堡大学MATH08064作业代写的成功案例
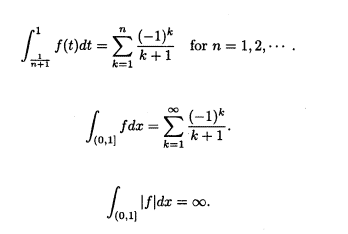
Since $\sigma$ is integrable over $J_{n}$ and continuous at the endpoints of $J_{n}, \int_{J_{n}} \sigma=\int_{K} 1_{J_{n}} \sigma$ by (i). So we can define $G_{n}$ on $K=[a, b]$ by
$$
G_{n}(t)=\int_{a}^{t} 1_{J_{n}} \sigma .
$$
The function $G_{n}$ is continuous since $\sigma$ is a continuous differential. By Theorem $1(\S 2.5),(17)$ implies
$$
d G_{n}=1_{J_{n}} \sigma \text { for all } n .
$$
$\sum_{n=1}^{\infty}\left|\Delta G_{n}\left(I_{n}\right)\right|<c$ for all $I_{n} \subseteq J_{n}$. Hence,
$$
\sum_{n=1}^{\infty} \operatorname{diam} G_{n}(K) \leq c<\infty
$$
Now for the sup norm in (5) of Theorem 2,
$$
\left|G_{n}\right| \leq \operatorname{diam} G_{n}(K)
$$

MATH08064 COURSE NOTES :
Let $A_{n}^{}=A_{n} \cup A_{n+1} \cup \cdots$. Then $A_{n}^{} \searrow A$ where $A=\varlimsup \lim A_{n}$. Clearly $A_{n}, A_{n}^{}, A$ are all measurable. By (3) $$ \int_{K} \sigma^{+}<\int_{K} 1_{A_{n}^{}} \sigma^{+}+\frac{1}{2^{n}}
$$
since $1_{A_{n}} \sigma \leq 1_{A_{n}} \sigma^{+} \leq 1_{A_{n}^{*}} \sigma^{+}$. (3) also implies
$$
\int_{K} 1_{A_{n}} \sigma^{-}<\frac{1}{2^{n}}
$$
since $1_{A_{n}} \sigma^{-} \leq 1_{A_{n}} \sigma^{-}+\left(1-1_{A_{n}}\right) \sigma^{+}=\sigma^{+}-1_{A_{n}} \sigma$.