
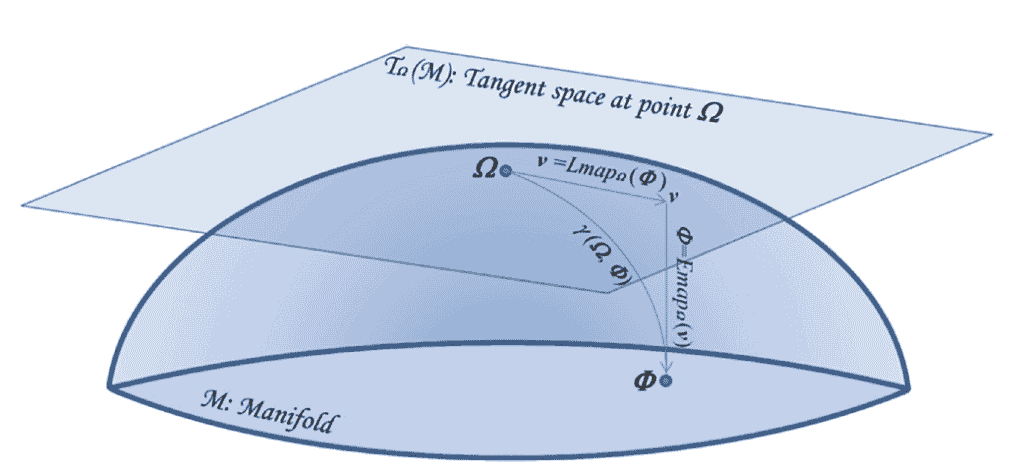
Let $M$ be a Riemannian manifold. For any $\xi \in T M$, define the length of $\xi$, $|\xi|$, by
$$
|\xi|=\langle\xi, \xi\rangle^{1 / 2} .
$$
For any path $\omega:[\alpha, \beta] \rightarrow M \in D^{1}$ define the length of $\omega, \ell(\omega)$ by
$$
\ell(\omega)=\int^{\beta}\left|\omega^{\prime}(t)\right| d t .
$$
For $M$ connected (our usual assumption), $p, q \in M$, define the distance between $p$ and $q, d(p, q)$, by
$$
d(p, q)=\inf _{\omega} \ell(\omega),
$$
where $\omega$ ranges over all $\omega:[\alpha, \beta] \rightarrow M \in D^{1}$ satisf ying $\omega(\alpha)=p, \omega(\beta)=q$.
One immediately verifies that
$$
\begin{aligned}
&d(p, q)=d(q, p) \
&d(p, q) \geq 0 \
&d(p, q) \leq d(p, r)+d(r, q)
\end{aligned}
$$

MATH0072 COURSE NOTES :
$$
\omega_{t}(0)=t \xi, \quad \omega_{t}^{\prime}(0)=t \Im_{t \xi} \eta \in(\mathrm{S}(p ; t)){t \xi} . $$ Thus, the map $$ \eta \mapsto t \Im{t \xi} \eta
$$
is an isomorphism of $\xi^{\perp}$ onto $(\mathrm{S}(p ; t)){t \xi}$. In particular, if $\zeta \in(\mathrm{S}(p ; t)){t \xi}$, let $\eta \in \xi^{\perp}$ be given by
$$
\eta=t^{-1} \Im_{t \xi}^{-1} \zeta,
$$
and consider
$$
v(t, \theta)=\exp \omega_{t}(\theta) ;
$$