这是一份drps爱丁堡大学MATH10028作业代写的成功案
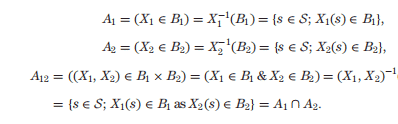

\begin{aligned}
&Y_{1}=\frac{1}{\sqrt{k}} Z_{1}+\frac{1}{\sqrt{k}} Z_{2}+\cdots+\frac{1}{\sqrt{k}} Z_{k} \
&Y_{2}=\frac{1}{\sqrt{2 \times 1}} Z_{1}-\frac{1}{\sqrt{2 \times 1}} Z_{2}
\end{aligned}
The variance of a r.v. $X$ is denoted by $\operatorname{Var}(X)$ and is defined by:
$$
\operatorname{Var}(X)=E(X-E X)^{2} \text {. }
$$
The explicit expression of the right-hand side in (8) is taken from (6) for $g(x)=(x-E X)^{2}$. The alternative notations $\sigma^{2}(X)$ and $\sigma_{X}^{2}$ are also often used for the $\operatorname{Var}(X)$.
MATH10028 COURSE NOTES :
F_{n}(x)=\left{\begin{array}{ll}
0, & \text { if } x<1-\frac{1}{n} \
\frac{1}{2}, & \text { if } 1-\frac{1}{n} \leq x<1+\frac{1}{n}, \
1, & \text { if } x \geq 1+\frac{1}{n}
\end{array} \quad G(x)= \begin{cases}0, & \text { if } x<1 \
1, & \text { if } x \geq 1\end{cases}\right.