这是一份GLA格拉斯哥大学MATHS5049_1作业代写的成功案例
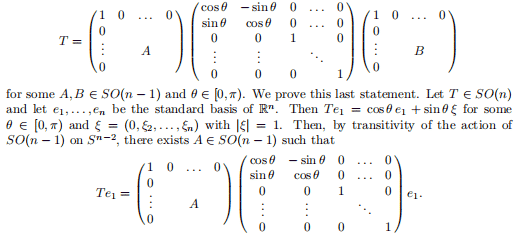
A chart of dimension $d$ for $X$ is a pair $(U, \psi)$ with $U$ an open subset of $X$ en $\psi: U \rightarrow \mathbb{R}^{d}$ such that $\psi(U)$ is open in $\mathbb{R}^{d}$ and $\psi: U \rightarrow \psi(U)$ is a homeomorphism.
Twee charts $\left(U_{1}, \psi_{1}\right)$ and $\left(U_{2}, \psi_{2}\right)$ for $X$ are called $C^{\infty}$-compatible if they have the same dimension $d$ and if the $\operatorname{map} \psi_{2} \circ \psi_{1}^{-1}: \psi_{1}\left(U_{1} \cap U_{2}\right) \rightarrow \psi_{2}\left(U_{1} \cap U_{2}\right)$ is a $C^{\infty}{ }{-}$ diffeomorphism. (Observe that $\psi{1}\left(U_{1} \cap U_{2}\right)$ is open in $\psi_{1}\left(U_{1}\right)$, hence it is also open in $\mathbb{R}^{d}$.)
Let $\left{\left(U_{\alpha}, \psi_{\alpha}\right)\right}_{\alpha \in A}$ be a $C^{\infty}$-atlas for $X$. A chart $(U, \psi)$ for $X$ is called $C^{\infty}$-compatible with the atlas if $(U, \psi)$ is $C^{\infty}$-compatible with each chart $\left(U_{\alpha}, \psi_{\alpha}\right)$ in the atlas. Two $C^{\infty}$-atlases for $X$ are called equivalent if each chart in the first atlas is $C^{\infty}$-compatible with each chart in the second atlas. Two $C^{\infty}$-manifolds built on the same topological space $X$ and having equivalent atlases will not be distinguished from each other as $C^{\infty}$-manifolds.

MATHS5049_1 COURSE NOTES :
$$
\psi_{S}^{-1}(y)=\widetilde{x}, \quad \text { where } \quad x:=\alpha_{S}\left(\begin{array}{l}
1 \
y
\end{array}\right)
$$
Here $\left(\begin{array}{l}1 \ y\end{array}\right)$ is a $(k+(n-k)) \times k$ block matrix. Now let $\xi \in U_{S} \cap U_{T}$ and put $y:=\psi_{S}(\xi)$, $z:=\psi_{T}(\xi), x:=\alpha_{S}\left(\begin{array}{l}1 \ y\end{array}\right)$. Then $\xi=\widetilde{x}$ and $x=\alpha_{T}\left(\begin{array}{l}1 \ z\end{array}\right) \lambda$ for some $\lambda \in G L(k, \mathbb{R})$. Hence
$$
\left(\begin{array}{l}
1 \
z
\end{array}\right) \lambda=\alpha_{T}^{-1} \alpha_{S}\left(\begin{array}{l}
1 \
y
\end{array}\right) .
$$
Write $\alpha_{T}^{-1} \alpha_{S}$ as a $(k+(n-k)) \times(k+(n-k))$ block matrix $\alpha_{T}^{-1} \alpha_{S}=\left(\begin{array}{ll}a & b \ c & d\end{array}\right)$. Then $\lambda=a+b y, z \lambda=c+d y$. Hence $z=(c+d y)(a+b y)^{-1}$, by which we have found the explicit expression for the transition map
$$
\psi_{T}^{-1} \circ \psi_{S}: \quad y \mapsto(c+d y)(a+b y)^{-1}: \quad \psi_{S}\left(U_{S} \cap U_{T}\right) \rightarrow \psi_{T}\left(U_{S} \cap U_{T}\right),
$$
and see that it is $C^{\infty}$.