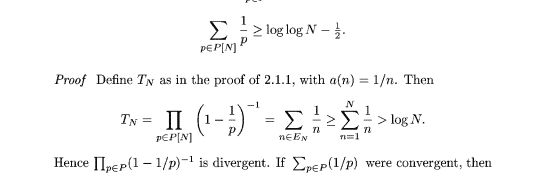
The series for $h(z)$ certainly converges when $|z|<1$. By termwise differentiation (which is valid for power series), we have
$$
h^{\prime}(z)=\sum_{m=1}^{\infty} z^{m-1}=\frac{1}{1-z}
$$
hence
$$
\frac{d}{d}(1-z) e^{h(z)}=-e^{h(z)}+\frac{1-z}{t} e^{h(z)}=0
$$
Hence $(1-z) e^{h(z)}$ is constant (say $=c$ ) for $|z|<1$. Take $z=0$ to get $c=e^{0}=1$. Further, when $|z| \leq \frac{1}{2}$,
$$
|h(z)| \leq \sum_{m=1}^{\infty}|z|^{m}=\frac{|z|}{1-|z|} \leq 2|z|
$$

MATH0083 COURSE NOTES :
Proof The second statement follows from the first one, since $u * \mu=e_{1}$. To prove the first statement, note first that $\Lambda(1)=\ell(1)=0$. Now choose $n>1$, with prime factorization
$$
n=p_{1}^{r_{1}} \ldots p_{k}^{r_{k}} .
$$
The divisors $i$ of $n$ for which $\Lambda(i) \neq 0$ are the numbers $p_{j}^{s_{j}}$ for $1 \leq j \leq k$ and $1 \leq s_{j} \leq r_{j}$. Each of these has $\Lambda(i)=\log p_{j}$. Hence
$$
\begin{aligned}
(\Lambda * u)(n) &=\sum_{i \mid n} \Lambda(i) \
&=\sum_{j=1}^{k} r_{j} \log p_{j} \
&=\log n
\end{aligned}
$$