这是一份 Imperial帝国理工大学 MATH97232作业代写的成功案例
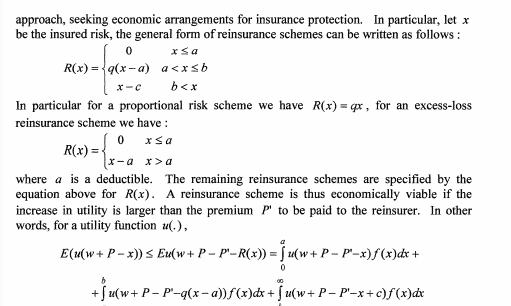
This is the case since when we are in state $i$, it is possible that a state $j$ will never be reached. This case corresponds to $i$ and j not communicating. Inversely, when $i$ and $j$ communicate (i.e. it is possible to switch from $i$ to $j$ in a finite number of transitions), we can then calculate the expectation of the passage time from $i$ to $j$. To do so, define explicitly $f_{i j}(n)=P_{i}\left(T_{j}=n\right)$ where $T_{j}$ is the first time the state $j$ is reached, or, $\left(x_{k}=j\right)$ written by :
$$
T_{j}=\operatorname{Inf}\left(k \geq 0, x_{k}=j\right)
$$
Let this expectation be given by :
$$
\mu_{i j}=\sum_{n=0}^{\infty} n f_{i j}(n)=E\left(T_{j} / x_{0}=i\right)
$$
$$
\mu_{i j}=1+\sum_{k \neq j} P_{i k} \mu_{k j}
$$
Note that if $k=j, \mu_{k j}=\mu_{k k}=E\left(T_{k} / k=k\right)=0$. In our case, we have :
$$
\begin{aligned}
&\mu_{21}=1+\mu_{21}(0.5)+\mu_{31}(0.4) \
&\mu_{31}=1+\mu_{21}(0.3)+\mu_{31}(0.6)
\end{aligned}
$$
which leads to
$$
\mu_{21}=\mu_{31}=10 \text { months }
$$

MATH97232 COURSE NOTES :
$$
E(x)=\int_{\Gamma} x(\alpha) d P(\alpha)=\int_{-\infty}^{+\infty} x d F(x)
$$
These integrals are called Stieljes integrals (when they exist). The variance is defined, however, by:
$$
\sigma_{x}^{2}=\int_{\Gamma}(x(\alpha)-E(x))^{2} d P(\alpha)=\int_{-\infty}^{+\infty}(x-E(x))^{2} d F(x)
$$
Finally, partial moments are defined by :
$$
E_{a}^{b}(X)=\int_{a}^{b} x d F(x)
$$