Tag Archives: Complex Analysis online tutor

复分析|MATH0013 Analysis 3: Complex Analysis代写2023

0
Posted on by

Assignment-daixieTM为您提供伦敦大学学院 London’s Global University MATH0013 Analysis 3: Complex Analysis复分析代写代考和辅导服务！

Instructions:

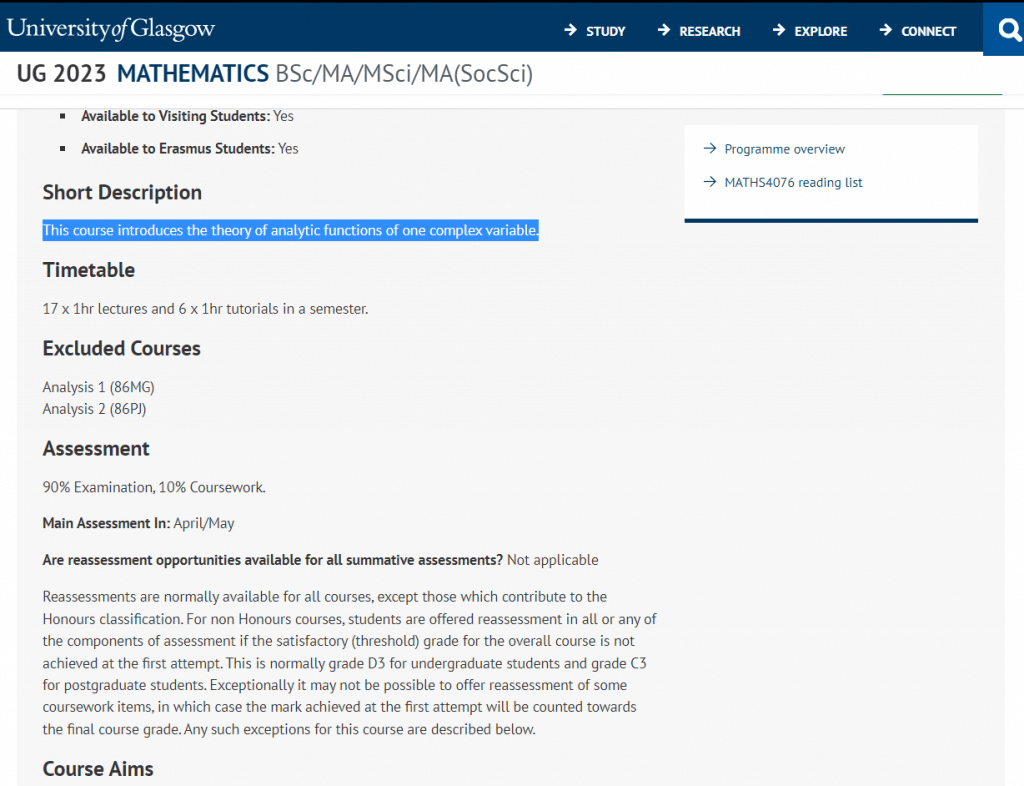
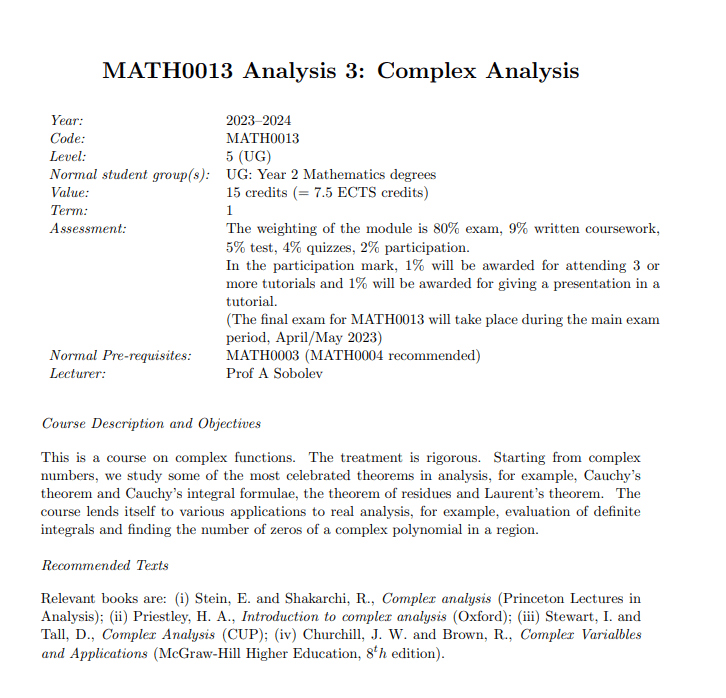
Complex analysis is a branch of mathematics that studies complex functions, which are functions that have complex numbers as their inputs and outputs. It is an important area of study because complex functions have many interesting and useful properties that are not shared by real-valued functions.

Starting from complex numbers is a good way to introduce the subject, as it allows you to develop the algebraic and geometric properties of complex numbers that are essential for understanding complex functions. Cauchy’s theorem and Cauchy’s integral formulae are fundamental results in complex analysis that relate the values of a complex function inside a closed contour to its values on the boundary of the contour. These theorems have many important applications, such as evaluating definite integrals and finding the number of zeros of a complex polynomial in a region.

The theorem of residues is another important result in complex analysis that allows you to compute certain types of integrals using the residues of a function. This theorem is particularly useful for evaluating integrals involving trigonometric and logarithmic functions.

Laurent’s theorem is a generalization of Taylor’s theorem for complex functions, which allows you to express a complex function as a power series with both positive and negative powers of the complex variable. This theorem is useful for studying the singularities of a complex function, which are points where the function is not defined or behaves in an unusual way.

Overall, the course on complex functions seems to cover a lot of important topics in complex analysis that have many applications in mathematics and other fields.

数学方法|MATH0010 Mathematical Methods 1代写2023

问题 1.

Let $G$ be an undirected graph with $n$ vertices. Then a Hamilton path is a simple path in $G$ that visits each vertex once (i.e., has $n$ vertices and $n-1$ edges), while a Hamilton cycle is a simple cycle in $G$ that visits each vertex once (i.e., has $n$ vertices and $n$ edges). Let HAMPATH and HAMCYCLE be the problems of deciding whether $G$ has a Hamilton path and Hamilton cycle respectively, given $G$ as input. (a) Show that if $G$ has a Hamilton cycle, then $G$ also has a Hamilton path.

证明 .

(a) If $G$ has a Hamilton cycle, then we can simply remove one edge from the cycle to obtain a Hamilton path. Since a Hamilton cycle has $n$ edges, removing one edge from it leaves a path with $n-1$ edges that visits every vertex exactly once. Therefore, if $G$ has a Hamilton cycle, it also has a Hamilton path.

问题 2.

(b) Give an example of a graph $G$ that has a Hamilton path but no Hamilton cycle.

证明 .

(b) Consider the graph $G$ consisting of three vertices $a,b,c$ and three edges $(a,b),(b,c),(c,a)$. This graph has a Hamilton path $a\rightarrow b\rightarrow c$, but no Hamilton cycle. To see this, note that any cycle in $G$ must contain all three vertices, and therefore must have length at least 3. But the only cycle in $G$ is of length 3, and it visits each vertex twice.

问题 3.

(c) Give a polynomial-time reduction from $H A M C Y C L E$ to $H A M P A T H$.

证明 .

(c) To reduce $HAMCYCLE$ to $HAMPATH$, we can simply remove one vertex $v$ from $G$ and ask whether the resulting graph $G’$ has a Hamilton path. If $G$ has a Hamilton cycle, then $G’$ has a Hamilton path by part (a). Conversely, if $G’$ has a Hamilton path, we can add the vertex $v$ back into the path to obtain a Hamilton cycle in $G$. This reduction takes polynomial time since we only need to remove one vertex and its adjacent edges, which can be done in constant time per vertex.

这是一份2023年的伦敦大学学院 MATH0013 Analysis 3: Complex Analysis复分析代写的成功案例

复分析代写|METHODS IN COMPLEX ANALYSIS MATHS4076 University of Glasgow Assignment

0
Posted on by

Assignment-daixieTM为您提供格拉斯哥大学University of Glasgow METHODS IN COMPLEX ANALYSIS MATHS4076复分析代写代考和辅导服务！

Instructions:

It deals with functions that are differentiable in the complex sense, meaning that they satisfy the Cauchy-Riemann equations. These functions are also called holomorphic functions.

The theory of analytic functions has many important applications in various fields, including physics, engineering, and mathematics. Some of the key topics that you might encounter in this course include power series expansions, Laurent series, singularities, residues, conformal mappings, and the Cauchy integral formula.

Throughout the course, you will learn about the properties and behavior of analytic functions, and you will develop techniques for analyzing and manipulating these functions. You will also study the connections between analytic functions and other areas of mathematics, such as complex geometry and number theory.

Overall, the study of analytic functions is a fascinating and important topic in mathematics, and it is essential for anyone interested in pursuing a career in pure or applied mathematics.

复分析代写|METHODS IN COMPLEX ANALYSIS MATHS4076 University of Glasgow Assignment

问题 1.

Let $\sum_{n=1}^{\infty} z_n$ be a convergent series of complex numbers. (1) Let $\alpha<\pi / 2$. Show that if for all $n,\left|\operatorname{Arg} z_n\right|<\alpha$, then the series $\sum_{n=1}^{\infty} z_n$ converges absolutely.

证明 . (1) Let $a_n = |z_n|$ for all $n$. Since $|\operatorname{Arg} z_n| < \alpha < \frac{\pi}{2}$, we have $\cos(\operatorname{Arg} z_n) > 0$ for all $n$. Hence, we have $$a_n = |z_n| = |z_n|\cos(\operatorname{Arg} z_n) < |z_n| \cos \alpha$$ By the comparison test, the series $\sum_{n=1}^\infty a_n$ converges. Therefore, by the absolute convergence test, the series $\sum_{n=1}^\infty z_n$ converges absolutely.

问题 2.

(2) Does the conclusion of the previous part hold if $\alpha=\pi / 2$ ?

证明 .

(2) The conclusion of part (1) does not hold if $\alpha = \frac{\pi}{2}$. For example, consider the series $\sum_{n=1}^\infty \frac{i^n}{n}$, where $i = \sqrt{-1}$. For all $n$, we have $|\operatorname{Arg} (i^n/n)| = \frac{\pi}{2}$. However, the series converges conditionally by the alternating series test.

问题 3.

(3) Assume that for all $n, \Re z_n \geq 0$ and that the series $\sum_{n=1}^{\infty} z_n^2$ also converges. Show that the series $\sum_{n=1}^{\infty} z_n^2$ converges absolutely.

证明 .

(3) Since $\Re z_n \geq 0$ for all $n$, we have $|z_n| = \sqrt{z_n \overline{z_n}} = \sqrt{z_n^2} = z_n$. Therefore, we have $$|z_n^2| = |z_n|^2 = z_n^2$$ Since the series $\sum_{n=1}^\infty z_n^2$ converges, we have $$\sum_{n=1}^\infty |z_n^2| = \sum_{n=1}^\infty z_n^2$$ converges, and hence the series $\sum_{n=1}^\infty z_n$ converges absolutely.

这是一份2023年的格拉斯哥大学University of Glasgow METHODS IN COMPLEX ANALYSIS MATHS4076代写的成功案例

复分析 Complex Analysis|MATH 8811Boston College Assignment

0
Posted on by

Assignment-daixieTM为您提供波士顿学院Boston College MATH 8811 Complex Analysis复分析代写代考和辅导服务！






Instructions:





Another important result in the global theory of analytic functions is the maximum modulus principle, which states that an analytic function attains its maximum modulus on the boundary of its domain. This result has many applications in complex analysis, including the proof of the fundamental theorem of algebra, which states that every non-constant polynomial with complex coefficients has at least one complex root.





In summary, the theory of analytic functions of one variable is a rich subject with many important results and applications. Its local and global aspects provide powerful tools for understanding the behavior of complex functions, and it has many connections to other areas of mathematics and physics.










几何学/拓扑学代写 Geometry/Topology I|MATH 8808 Boston College Assignment













问题 1. 





 For $z=1+\imath$ find $w$ such that the real parts of the following numbers are equal to zero
a) $z+w_i$
b) $z \cdot w$
c) $\frac{z}{w} ;$
d) $\frac{w}{z}$.





 
 




证明 . 





Solution. Let $z=1+\imath=(1,1)$ and $w=x+i y=(x, y)$. Then we have
a)
$$
\operatorname{Re}(z+w)=0 \Longleftrightarrow 1+x=0
$$
Hence $x=-1$ and $y \in \mathbb{R}$ is arbitrary.
b)
$$
\operatorname{Re}(z \cdot w)=0 \Longleftrightarrow \operatorname{Re}(x-y+i(x+y))=0 .
$$
Hence $x-y=0$. Therefore $w$ is given by $w=x+\imath x=x(1+\imath)=x \cdot z$, for arbitrary $x \in \mathbb{R}$
c) We have $\frac{z}{w}=0$. Hence
$$
\frac{z}{w}=\frac{1+\imath}{x+2 y} \cdot \frac{x-v y}{x-v y}=\frac{x+y+\imath(x-y)}{x^2+y^2}
$$
Therefore
$$
\operatorname{Re}\left(\frac{z}{w}\right)=0 \Longleftrightarrow \frac{x+y}{x^2+y^2}=0 \Rightarrow x=-y, x \neq 0 .
$$
Finally we have $w=x(1-2)$ for $x \in \mathbb{R}$ and $x \neq 0$.
d) From $\operatorname{Re}\left(\frac{w}{z}\right)=0$ it follows that for every $x \in \mathbb{R}$ :
$$
\frac{w}{z}=\frac{x+\imath y}{1+\imath} \cdot \frac{1-\imath}{1-\imath}=\frac{x+y+\imath(x-y)}{2} .
$$
Hence $w=x(1-\imath)$ for every $x \in \mathbb{R}$.


















问题 2. 





Let $\mathbb{C}^$ be the set of all complex numbers different from zero. a) Prove that the set $T$ of all complex numbers with modulus 1 is a multiplicative subgroup of the group $\left(\mathbb{C}^, \cdot\right)$.
b) The multiplicative group $\mathbb{C}^*$ is isomorphic with $\mathbb{R}^{+} \times T$.





 
 




证明 . 





Solution. a) We have $T={z|| z \mid=1} \subset \mathbb{C}^* . T$ under the multiplication by the equality
$$
\left|z_1 \cdot z_2\right|=\left|z_1\right| \cdot\left|z_2\right| \text {. }
$$
The associativity follows by the associativity of multiplication in $\mathbb{C}$. The neutral element is 1. The inverse of $z=(a, b) \in T$ is
$$
\left(\frac{a}{a^2+b^2}, \frac{-b}{a^2+b^2}\right) \in T \text {. }
$$
b) It is easy to check that an isomorphism is given by
$$
f(z)=(|z|, \cos \theta+i \sin \theta)
$$
where $\theta=\arg z$. nopagebreak c) We define an equivalence relation $\sim$ on $\mathbb{R}$ by $r_1 \sim r_2 \Longleftrightarrow r_1-r_2=2 k \pi, \quad k$ is an integer. Let $\mathbb{R}$ be the corresponding quotient set. Prove that $\mathbb{R}^{+} \times T$ isomorphic with $\mathbb{R}^{+} \times \hat{\mathbb{R}}$. The group $\mathbb{C}^*$ is isomorphic with $\mathbb{R}^{+} \times \tilde{\mathbb{R}}$ (check). Therefore by b) and transitivity of the isomorphisms of groups it follows c).














问题 3. 





 Starting with a complex number $z \neq 0$, find where the complex numbers $2 z, 3 z, \ldots, n z$ are ?





 
 




证明 . 





Solution. For $z=\rho(\cos \theta+\imath \sin \theta)$ we have
$$
n z=n \rho(\cos \theta+i \sin \theta)
$$
the complex numbers $n x$, for $n=1,2, \ldots$ are on the half straight line $y=\tan \theta \cdot x$ with modulus $n \rho$.




















这是一份2023年的波士顿学院Boston College MATH 8811几线性代数代写的成功案例




























































































































































			

	
	



		
			


	

		
复分析代写Complex Analysis|MATH 116 Stanford University Assignment


				0
		
				

			Posted on  by 		

		
	


		

		

Assignment-daixieTM为您提供斯坦福大学Stanford University MATH 116 Complex Analysis复分析代写代考和辅导服务！





Instructions:





Analytic functions are functions that can be represented by a power series expansion in the complex plane, meaning that they are differentiable and have no singularities (except perhaps at isolated points).





The Cauchy integral formula states that if f(z) is a complex analytic function and γ is a simple closed contour in the complex plane that encloses a point z₀, then the value of the integral of f(z) along γ is equal to 2πi times the value of f(z) at z₀. This formula is a powerful tool for computing complex integrals.





Power series are series of the form Σn=0∞ an(z-z₀)^n, where an are complex coefficients, z₀ is a fixed complex number, and z is a complex variable. Analytic functions can be represented as power series expansions, which allows us to manipulate them algebraically and compute their values at specific points.





Laurent series are similar to power series, but they allow for terms with negative exponents. Laurent series can be used to represent functions that have isolated singularities in the complex plane, and they are useful for computing complex integrals using the calculus of residues.





The calculus of residues is a method for computing complex integrals by using the residues of a function at its singularities. The residue of a function at a point z₀ is a complex number that can be computed using the Laurent series expansion of the function around that point.





Conformal mapping is a technique for mapping one region of the complex plane onto another in a way that preserves angles and shapes. Conformal mappings are useful for solving problems in complex analysis, such as finding the solutions to differential equations with boundary conditions.





Analytic continuation is a method for extending the domain of an analytic function beyond its original domain by using the properties of the function to construct a new function that agrees with the original function on its domain and is analytic on a larger domain.





Riemann surfaces are surfaces that generalize the complex plane to allow for the existence of multiple values of a function. Riemann surfaces are used in complex analysis to study functions that have branch points or other singularities.





Fourier series and integrals are techniques for representing periodic functions as infinite sums or integrals of complex exponential functions. Fourier series and integrals are used in a wide variety of applications, including signal processing, image compression, and quantum mechanics.



















复分析代写Complex Analysis|MATH 116 Stanford University Assignment













问题 1. 





Let $h$ be a continuous function $h: R^n \rightarrow R^n$. Let $x_0 \in R^n$. Suppose that $h^n\left(x_0\right) \rightarrow z$ as $n \rightarrow \infty$. Show that $h(z)=z$.





 
 




证明 . 





Solution
The ellipse meets the $x$-axis at the points $(a, 0)$ and $(-a, 0)$. By Theorem $5.19$, we do not need to find a parametrisation of $\gamma$ :
$$
\int_\gamma \cos z d z=[\sin z]_a^{-a}=-2 \sin a .
$$














问题 2. 





Let $f(z)=1 /\left(z^3+1\right)$ and let $\gamma(t)=R e^{i t}(0 \leq t \leq \pi)$. Show that
$$
\lim {R \rightarrow \infty}\left|\int\gamma f(z) d z\right|=0
$$





 
 




证明 . 





For each point on $\gamma^$, $$ |f(z)|=\left|\frac{1}{R^3 e^{3 i t}+1}\right|=\frac{1}{\left|R^3 e^{3 i t}+1\right|} . $$ Now, by Theorem $2.1$, $$ \left|R^3 e^{3 i t}+1\right| \geq\left|R^3 e^{3 i t}\right|-1=R^3-1, $$ and the length of $\gamma^$ is $R \pi$. Hence
$$
0 \leq\left|\int_\gamma f(z) d z\right| \leq \frac{R \pi}{R^3-1},
$$
which clearly tends to zero as $R$ tends to infinity.
















问题 3. 





For each $n \geq 1$, let $f_n$ be a complex function with domain $S$, and suppose that there exist positive numbers $M_n(n \geq 1)$ such that $\left|f_n\right| \leq M_n$. If $\sum_{n=1}^{\infty} M_n$ is convergent, then $\sum_{n=1}^{\infty} f_n$ is uniformly convergent in $S$.





 
 




证明 . 









For each $z$ in $S$ and each $n \geq 1$
$$
\left|f_n(z)\right| \leq\left|f_n\right| \leq M_n,
$$
and so, by the Comparison Test, $\sum_{n=1}^{\infty} f_n(z)$, being absolutely convergent, is convergent. Denote its sum by $F(z)$ and its sum to $N$ terms by $F_N(z)$. Let $\epsilon>0$. Since $\sum_{n=1}^{\infty} M_n$ is convergent, there exists $N$ such that, for all $n>N$,
$$
\sum_{k=n+1}^{\infty} M_k<\epsilon / 2 \text {. } $$ Hence, for all $m>n>N$ and all $z$ in $S$,
$$
\left|\sum_{k=n+1}^m f_k(z)\right| \leq \sum_{k=n+1}^m\left|f_k(z)\right| \leq \sum_{k=n+1}^m M_k<\epsilon / 2 . $$ Letting $m$ tend to $\infty$, we deduce that, for all $z$ in $S$, $$ \left|F(z)-F_n(z)\right| \leq \epsilon / 2<\epsilon . $$ Hence $\left|F-F_n\right|<\epsilon$ for all $n>N$, and the proof is complete.




















这是一份2023年的斯坦福大学Stanford University MATH 116复分析代写的成功案例




























































































































































			

	
	



		
			


	

		
复分析代写 Complex Analysis|MATH 333 Duke University Assignment


				0
		
				

			Posted on  by 		

		
	


		

		

Assignment-daixieTM为您提供杜克大学Duke University MATH 333 Complex Analysis复分析代写代考和辅导服务！










Instructions:





Complex analysis is the branch of mathematics that deals with functions of complex variables. It is a powerful tool for studying complex functions and their properties, as well as for solving problems in a wide range of fields, including physics, engineering, and finance.





At its core, complex analysis is concerned with the study of complex functions, which are functions that take complex numbers as inputs and outputs. These functions can be expressed as power series, integrals, or contour integrals, and they have many interesting and useful properties, such as analyticity, holomorphy, and conformality.





Analyticity is a property of complex functions that implies that they are differentiable infinitely many times within their domain. Holomorphy is a related property that implies that a function is analytic in a domain and has no singularities within that domain. Conformality is a property that is related to the preservation of angles and shapes under a transformation.





Complex analysis also involves the study of complex integrals and complex contour integrals, which are integrals that are taken over complex domains. These integrals are often used to solve complex equations and to evaluate complex functions.





Some of the applications of complex analysis include the study of complex functions in quantum mechanics, fluid mechanics, and electromagnetism. It is also used in the study of differential equations, partial differential equations, and numerical analysis. In addition, complex analysis has applications in finance, such as in the pricing of financial derivatives and the analysis of financial markets.



















复分析代写 Complex Analysis|MATH 333 Duke University Assignment













问题 1. 





Find all the complex roots of the equation $\cos z=3$.





 
 




证明 . 





Solution. Since $\cos z=\left(e^{i z}+e^{-i z}\right) / 2$, it comes down to solve the equation $e^{i z}+$ $e^{-i z}=6$, i.e.,
$$
w+w^{-1}=6 \Leftrightarrow w^2-6 w+1=0
$$
if we let $w=e^{i z}$. The roots of $w^2-6 w+1=0$ are $w=3 \pm 2 \sqrt{2}$. Therefore, the solutions for $\cos z=3$ are
$$
i z=\log (3 \pm 2 \sqrt{2}) \Leftrightarrow z=-i(\ln (3 \pm 2 \sqrt{2})+2 n \pi i)=2 n \pi-i \ln (3 \pm 2 \sqrt{2})
$$
for $n$ integers.














问题 2. 





Find all solutions $z \in \mathbb{C}$ of of the following (express your answers in the form $x+i y$ ):
(a) $\log z=4 i$;
(b) $z^i=i$.









 
 




证明 . 





Solution. (a) We have that $\exp (\log z)=z$. Thus
$$
z=\exp (\log z)=\exp (4 i)=\cos 4+i \sin 4
$$
Notice that, if $z=\exp (4 i)$, then we have
$$
\log (\exp (4 i))=4 i+2 n \pi i \quad(n \in \mathbb{Z})
$$
In particular, for $n=0$, we have that $\log [\exp (4 i)]=4 i$.
(b) Method one: We know that $z^i=\exp (i \log z)$. Thus
$$
\exp (i \log z)=i
$$
Since $i=\exp (i(\pi / 2+2 n \pi))$, with $n \in \mathbb{Z}$, then we have
$$
\begin{aligned}
\exp \left(i\left(\frac{\pi}{2}+2 n \pi\right)\right) & =\exp [i(\ln |z|+i \arg (z))] \
& =\exp [-\arg (z)+i \ln |z|] \
& =\exp [-\arg (z)] \cdot \exp [i \ln |z|]
\end{aligned}
$$
Thus
$$
\arg (z)=2 k \pi(k \in \mathbb{Z}) \quad \text { and } \quad \ln |z|=\frac{\pi}{2}+2 n \pi \quad(n \in \mathbb{Z})
$$
Hence,
$$
z=\exp \left[\frac{\pi}{2}+2 n \pi\right] \quad(n \in \mathbb{Z}) .
$$
Method two: Consider the following identity
$$
\log [\exp (i \log z)]=\log i
$$
Thus
$$
\log [\exp (i \log z)]=i \log z+2 n_1 \pi i \quad\left(n_1 \in \mathbb{Z}\right)
$$
Hence, substituting in , we obtain
$$
i \log z+2 n_1 \pi i=\log i \quad\left(n_1 \in \mathbb{Z}\right)
$$
Thus
$$
\log z=-i \log i-2 n_1 \pi \quad\left(n_1 \in \mathbb{Z}\right)
$$
From the polar form of $i$, we have that $r=1$ and $\Theta=\frac{\pi}{2}$. Thus
$$
\log i=\ln 1+i\left(\frac{\pi}{2}+2 n_2 \pi\right) \quad\left(n_2 \in \mathbb{Z}\right)
$$
So
$$
\log z=-i\left[\ln 1+i\left(\frac{\pi}{2}+2 n_2 \pi\right)\right]-2 n_1 \pi=\frac{\pi}{2}+2 \pi\left(n_2-n_1\right)
$$









with $n_1, n_2 \in \mathbb{Z}$. Since $n_2-n_1 \in \mathbb{Z}$, we have
$$
\log z=\frac{\pi}{2}+2 n \pi \quad(n \in \mathbb{Z})
$$
Therefore, $z^i=i$ when
$$
z=\exp \left[\frac{\pi}{2}+2 n \pi\right] \quad(n \in \mathbb{Z})
$$










问题 3. Show that if $f(z)$ is continuous at $z_0$, so is $|f(z)|$.





 
 




证明 . 





Proof. Let $f(z)=u(x, y)+i v(x, y)$. Since $f(z)$ is continuous at $z_0=x_0+y_0 i, u(x, y)$ and $v(x, y)$ are continuous at $\left(x_0, y_0\right)$. Therefore,
$$
(u(x, y))^2+(v(x, y))^2
$$
is continuous at $\left(x_0, y_0\right)$ since the sums and products of continuous functions are continuous. It follows that
$$
|f(z)|=\sqrt{(u(x, y))^2+(v(x, y))^2}
$$
is continuous at $z_0$ since the compositions of continuous functions are continuous.


























这是一份2023年的杜克大学Duke University MATH 333复分析代写的成功案例