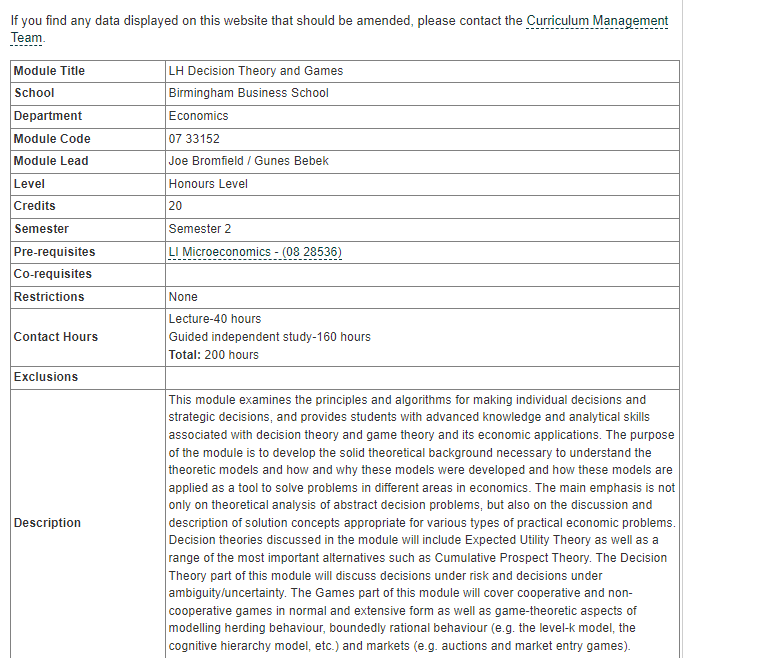
Assignment-daixieTM为您提供伯明翰大学University of Birmingham Decision Theory and Games 07 33152决策论和博弈论代写代考和辅导服务!
Instructions:
Covers a wide range of topics related to decision-making in economics, including decision theory, game theory, and their applications in different areas of economics. The module aims to provide students with a solid theoretical background to understand and analyze decision problems, as well as to discuss and describe solution concepts appropriate for practical economic problems.
The module covers various decision theories, including the widely used Expected Utility Theory and alternative theories such as Cumulative Prospect Theory. Additionally, it examines decisions under risk and decisions under ambiguity/uncertainty. It seems that the course will provide students with a comprehensive understanding of different types of decision-making scenarios, which will be useful for solving real-world problems in economics.
Provide an example of a 2-player game with strategy set $[0, \infty)$ for either player and payoffs continuous in the strategy profile, such that no strategy survives iterated deletion of strictly dominated strategies $\left(S^{\infty}=\emptyset\right)$, but the set of strategies remaining at every stage is nonempty $\left(S^k \neq \emptyset\right.$ for $\left.k=1,2, \ldots\right)$.
One example of such a game is the “Escalation Game” where two players, Player 1 and Player 2, choose a level of effort to put into a conflict. Each player can choose any non-negative amount of effort, represented by a real number in the interval $[0,\infty)$. The payoff for each player is the difference between their chosen level of effort, multiplied by a constant parameter $c>0$. Specifically, if Player 1 chooses an effort level of $x_1$ and Player 2 chooses an effort level of $x_2$, then Player 1’s payoff is $c(x_1-x_2)$ and Player 2’s payoff is $c(x_2-x_1)$.
If one player chooses a lower level of effort than the other, then they will lose the conflict and receive a negative payoff. If both players choose the same level of effort, they will tie and receive a payoff of 0. However, if both players choose a high level of effort, the conflict becomes too costly and both players receive a negative payoff.
Now, let us show that no strategy survives iterated deletion of strictly dominated strategies in this game. Consider the following argument:
- Any strategy profile where one player chooses a positive effort level strictly dominates a profile where that player chooses 0 effort. Therefore, we can eliminate all strategies where one player chooses 0 effort.
- Similarly, any strategy profile where one player chooses an effort level strictly greater than the other player’s effort level strictly dominates a profile where the first player chooses the same effort level as the second player. Therefore, we can eliminate all strategies where one player chooses a lower effort level than the other player.
However, after these eliminations, there are still infinitely many strategies remaining for both players (e.g., any positive real number is a valid strategy). Therefore, the set of strategies remaining at every stage is nonempty.
A game $G=(N, S, u)$ is said to be symmetric if $S_1=S_2=\cdots=S_n$ and there is some function $f: S_1 \times S_1^{n-1} \rightarrow \mathbb{R}$ such that $f\left(s_i, s_{-i}\right)$ is symmetric with respect to the entries in $s_{-i}$, and $u_i(s)=f\left(s_i, s_{-i}\right)$ for every player $i$. (1) Consider a symmetric game $G=(N, S, u)$ in which $S_1$ is a compact and convex subset of a Euclidean space and $u_i$ is continuous and quasiconcave in $s_i$. Show that there exists a symmetric pure-strategy Nash equilibrium (i.e. a pure-strategy Nash equilibrium where every player uses the same strategy).
To show that there exists a symmetric pure-strategy Nash equilibrium, we will use Kakutani’s fixed-point theorem, which states that if $S$ is a nonempty, compact, convex subset of a Euclidean space and $f: S \rightarrow S$ is a continuous, convex-valued mapping, then there exists a fixed point of $f$ (i.e. a point $s \in S$ such that $f(s) = s$).
Let $F(s) = (u_1(s), u_2(s), \ldots, u_n(s))$ be the vector of payoffs for all players when all players play strategy $s$. Since $u_i$ is quasiconcave in $s_i$, $F$ is quasiconcave in $s$. Furthermore, $S$ is a nonempty, compact, convex subset of a Euclidean space, and $F$ is continuous. Therefore, we can apply Kakutani’s fixed-point theorem to the mapping $F: S \rightarrow S$.
Since $S$ is convex, any fixed point of $F$ must be a symmetric pure-strategy Nash equilibrium. To see why, suppose that $s^$ is a fixed point of $F$, i.e. $F(s^) = s^$. Then, for any player $i$ and any deviation $s_i’$ from $s_i^$, we have $u_i(s_i^, s_{-i}^) \geq u_i(s_i’, s_{-i}^)$, since $s^$ is a Nash equilibrium. But since $u_i$ is quasiconcave in $s_i$, this implies that $u_i(s_i^, s_{-i}^) \geq u_i(s_i, s_{-i}^)$ for all $s_i \in S_i$. Therefore, $s^$ is a symmetric pure-strategy Nash equilibrium.
Suggest a definition for symmetric Bayesian games, $G=(N, A, \Theta, u, T, p)$, and find broad conditions on such a game $G$ that ensure that $G$ has a symmetric Bayesian Nash equilibrium.
In a symmetric Bayesian game, the set of actions $A_i$ is the same for all players $i$, and the set of types $\Theta_i$ is also the same for all players $i$. The payoff function $u_i$ is still a function of the joint action profile and the types, but we also have a common prior distribution $p$ over the types.
A symmetric Bayesian Nash equilibrium is a strategy profile and belief system for each player such that no player can improve their expected payoff by unilaterally changing their strategy or beliefs, given that all other players are using the same strategy profile and belief system.
To ensure that a symmetric Bayesian game has a symmetric Bayesian Nash equilibrium, we can require the following conditions:
- The set of actions and types is nonempty, compact, and convex.
- The payoff function is continuous and quasiconcave in the joint action profile and the types.
- The prior distribution is continuous and has full support over the set of types.
- The players’ beliefs are updated using Bayes’ rule, i.e. their posterior distribution over the types is proportional to the product of the likelihood function and the prior distribution.
- The likelihood function satisfies some regularity conditions, such as monotonicity and concavity in the types.
Under these conditions, we can apply Kakutani’s fixed-point theorem to the mapping from the set of belief systems to the set of best responses, which maps each belief system to the set of strategies that are best responses given that belief system. This mapping is continuous, convex-valued, and has a fixed point by the same argument as in part (1). Therefore, there exists a symmetric Bayesian Nash equilibrium in this game.
