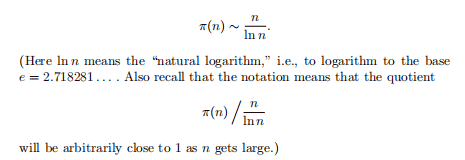Tag Archives: Discrete Mathematics代写

离散数学|MATH1004/MATH1904 Discrete mathematics代写 Sydney代写

0
Posted on by

这是一份Sydney悉尼大学MATH1004/MATH1904的成功案例

离散数学|MATH1004/MATH1904 Discrete mathematics代写 Sydney代写


A party $B$ sends a message to a party $A$ in the McEliece encryption scheme as follows:$B$ does the following to encrypt the message:

look up $A$ ‘s public key $(\widehat{G}, t)$;
$\diamond$ represent the message $m$ as a binary string of length $k$; if the message is too big, break it into blocks;
$\diamond$ choose a random error vector $z$ of length $n$ and Hamming weight $\leq t$;

compute $c=m \widehat{G}+z$;
send $c$ to $A$.

A does the following to decrypt the received message:
$\diamond$ compute $\widehat{c}=c P^{-1}$;
$\diamond$ use the decoding algorithm for the code generated by $G$ to decode $\widehat{c}$ to $\widehat{m}$;
$\diamond$ compute $m=\widehat{m} S^{-1}$.


英国论文代写Viking Essay为您提供作业代写代考服务

MATH1004/MATH1904 COURSE NOTES ：

Circuit axioms: A subset $\mathcal{C}$ of $2^{E}$ is the set of circuits of a matroid on $E$ if and only if $\mathcal{C}$ satisfies:

  • $\emptyset / \in \mathcal{C}$
  • no member of $\mathcal{C}$ is a proper subset of another;
  • circuit elimination: if $C_{1}, C_{2}$ are distinct members of $\mathcal{C}$ and $e \in C_{1} \cap C_{2}$, then $\mathcal{C}$ has a member $C_{3}$ such that $C_{3} \subseteq\left(C_{1} \cup C_{2}\right)-{e}$.
    Note: The circuit elimination axiom can be strengthened to the following:
  • strong circuit elimination: if $C_{1}, C_{2} \in \mathcal{C}, f \in C_{1}-C_{2}$, and $e \in C_{1} \cap C_{2}$, then $\mathcal{C}$ has a member $C_{3}$ such that $f \in C_{3} \subseteq\left(C_{1} \cup C_{2}\right)-{e}$.














离散数学 Discrete Mathematics MATH223001/MATH223101

0
Posted on by

这是一份leeds利兹大学MATH223001/MATH223101作业代写的成功案例

离散数学 Discrete Mathematics MATH223001/MATH223101
问题 1.

We apply the lower bound in Lemma 2.5.1 to the logarithms. For a typical term, we get
$$
\ln \left(\frac{m+t-k}{m-k}\right) \geq \frac{\frac{m+t-k}{m-k}-1}{\frac{m+t-k}{m-k}}=\frac{t}{m+t-k}
$$

证明 .

and so
$$
\begin{aligned}
\ln \left(\frac{m+t}{m}\right) &+\ln \left(\frac{m+t-1}{m-1}\right)+\cdots+\ln \left(\frac{m+1}{m-t+1}\right) \
& \geq \frac{t}{m+t}+\frac{t}{m+t-1}+\cdots+\frac{t}{m+1} .
\end{aligned}
$$
We replace each denominator by the largest one to decrease the sum:
$$
\frac{t}{m+t}+\frac{t}{m+t-1}+\cdots+\frac{t}{m+1} \geq \frac{t^{2}}{m+t} .
$$

英国论文代写Viking Essay为您提供作业代写代考服务

MATH223001/MATH223101 COURSE NOTES ：

For $n=1$ and $n=2$, if we require that $L_{n}$ be of the given form, then we get
$$
L_{1}=1=a+b, \quad L_{2}=3=a \frac{1+\sqrt{5}}{2}+b \frac{1-\sqrt{5}}{2}
$$
Solving for $a$ and $b$, we get
$$
a=\frac{1+\sqrt{5}}{2}, \quad b=\frac{1-\sqrt{5}}{2}
$$
Then
$$
L_{n}=\left(\frac{1+\sqrt{5}}{2}\right)^{n}+\left(\frac{1-\sqrt{5}}{2}\right)^{n}
$$








离散数学 Discrete Mathematics MATH00103

0
Posted on by

这是一份UCL伦敦大学 MATH00103作业代写的成功案例

离散数学 Discrete Mathematics MATH00·03
问题 1.

translates into
$$
c \cdot q^{n+1}=c \cdot q^{n}+c \cdot q^{n-1}
$$
which after simplification becomes
$$
q^{2}=q+1
$$


证明 .

So both numbers $c$ and $n$ disappear. ${ }^{1}$
So we have a quadratic equation for $q$, which we can solve and get
$$
q_{1}=\frac{1+\sqrt{5}}{2} \approx 1.618034, \quad q_{2}=\frac{1-\sqrt{5}}{2} \approx-0.618034 .
$$
This gives us two kinds of geometric progressions that satisfy the same recurrence as the Fibonacci numbers:
$$
G_{n}=c\left(\frac{1+\sqrt{5}}{2}\right)^{n}, \quad G_{n}^{\prime}=c\left(\frac{1-\sqrt{5}}{2}\right)^{n}
$$

英国论文代写Viking Essay为您提供作业代写代考服务

MATH00103 COURSE NOTES ：

(a) if $a \mid b$ and $b \mid c$ then $a \mid c$;
(b) if $a \mid b$ and $a \mid c$ then $a \mid b+c$ and $a \mid b-c$;
(c) if $a, b>0$ and $a \mid b$ then $a \leq b$;
(d) if $a \mid b$ and $b \mid a$ then either $a=b$ or $a=-b$.







离散数学|Discrete Mathematics代写 

0
Posted on by

这是一份oxford牛津大学作业代写的成功案例

离散数学|Discrete Mathematics代写
问题 1.

Unfortumately, the answer is yes. The smallest such number is $341=11 \cdot 31$. This is not a prime, but it satisfies
$$
341 \mid 2^{340}-1 .
$$
(How do we know that this divisibility relation holds without extensive computation? We can use Fermat’s Theorem. It is sufficient to argue that both 11 and 31 are divisors of $2^{340}-1$, since then so is their product, 11 and 31 being different primes. By Fermat’s Theorem,
$$
11 \mid 2^{10}-1
$$

证明 .

Next we invoke the result : It implies that
$$
2^{10}-1 \mid 2^{340}-1
$$
Hence
$$
11 \mid 2^{340}-1
$$
For 31, we don’t need Fermat’s Theorem, but only again:
$$
31=2^{5}-1 \mid 2^{340}-1
$$


英国论文代写Viking Essay为您提供作业代写代考服务

Oxford COURSE NOTES ：

4.3.2. For $n=1$ and $n=2$, if we require that $L_{n}$ be of the given form, then we get
$$
L_{1}=1=a+b, \quad L_{2}=3=a \frac{1+\sqrt{5}}{2}+b \frac{1-\sqrt{5}}{2}
$$
Solving for $a$ and $b$, we get
$$
a=\frac{1+\sqrt{5}}{2}, \quad b=\frac{1-\sqrt{5}}{2}
$$
Then
$$
L_{n}=\left(\frac{1+\sqrt{5}}{2}\right)^{n}+\left(\frac{1-\sqrt{5}}{2}\right)^{n}
$$





离散数学|Discrete Mathematics代写 5CCM251A

0
Posted on by

这是一份kcl伦敦大学学院 5CCM251A作业代写的成功案

离散数学|Discrete Mathematics代写 5CCM251A
问题 1.

3.8.2. We apply the lower bound in Lemma 2.5.1 to the logarithms. For a typical term, we get
$$
\ln \left(\frac{m+t-k}{m-k}\right) \geq \frac{\frac{m+t-k}{m-k}-1}{\frac{m+t-k}{m-k}}=\frac{t}{m+t-k}
$$


证明 .

and so
$$
\begin{aligned}
\ln \left(\frac{m+t}{m}\right) &+\ln \left(\frac{m+t-1}{m-1}\right)+\cdots+\ln \left(\frac{m+1}{m-t+1}\right) \
& \geq \frac{t}{m+t}+\frac{t}{m+t-1}+\cdots+\frac{t}{m+1}
\end{aligned}
$$

英国论文代写Viking Essay为您提供实分析作业代写Real anlysis代考服务

5CCM251A COURSE NOTES ：

For $n=1$ and $n=2$, if we require that $L_{n}$ be of the given form, then we get
$$
L_{1}=1=a+b, \quad L_{2}=3=a \frac{1+\sqrt{5}}{2}+b \frac{1-\sqrt{5}}{2}
$$
Solving for $a$ and $b$, we get
$$
a=\frac{1+\sqrt{5}}{2}, \quad b=\frac{1-\sqrt{5}}{2}
$$
Then
$$
L_{n}=\left(\frac{1+\sqrt{5}}{2}\right)^{n}+\left(\frac{1-\sqrt{5}}{2}\right)^{n}
$$