Assignment-daixieTM为您提供伦敦大学学院 London’s Global University MATH0015 Fluid Mechanics流体力学代写代考和辅导服务!
Instructions:
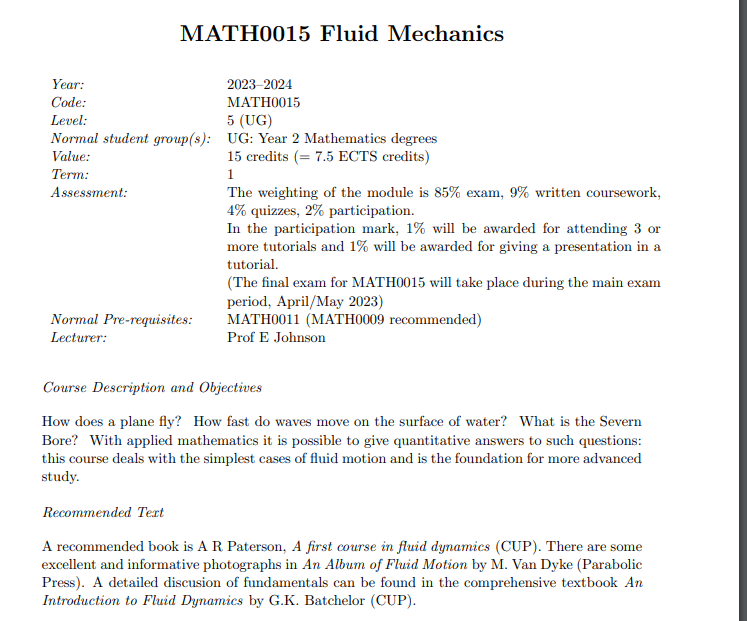
Fluid Mechanics is the study of fluids, which can be either liquids or gases, and their behavior when subjected to various forces and conditions. It is a branch of physics and engineering that deals with the motion and equilibrium of fluids, as well as their interactions with solid objects.
Fluid mechanics involves the study of topics such as fluid statics, which deals with fluids at rest, and fluid dynamics, which deals with fluids in motion. Fluid mechanics also encompasses topics such as viscosity, turbulence, and boundary layers, which are important in understanding the behavior of fluids.
Applications of fluid mechanics are found in various fields, including aerospace engineering, civil engineering, chemical engineering, mechanical engineering, and biomedical engineering. Understanding fluid mechanics is important for the design and analysis of many devices and systems, such as pumps, turbines, pipes, and blood flow in the human body.
Overall, fluid mechanics plays an important role in understanding the behavior of fluids and their applications in various fields of engineering and science.
Consider a sinusoidal traveling wave of small amplitude $(\mathrm{Ak}<<1)$ such that the free surface is given by: $$ \eta=A \cos (\mathrm{kx}-\omega t) $$ The corresponding velocities are given by: $$ \mathrm{u}=\mathrm{A} \omega \mathrm{e}^{\mathrm{ky}} \cos (\mathrm{kx}-\omega t) $$ and $$ v=A \omega e^{k y} \sin (k x-\omega t) $$ ( $\mathrm{y}$ is the vertical coordinate and is negative in the direction of $\mathrm{g} ; \mathrm{x}$ is the horizontal coordinate) a) Sketch the streamlines at $\mathrm{t}=\mathrm{o}$ that pass through: $\mathrm{x}=o, \mathrm{y}=o ; \mathrm{x}=0, \mathrm{y}=\frac{-2 \pi}{k}$
a) To sketch the streamlines at $t=0$ that pass through $(x,y)=(0,0)$ and $(x,y)=(0,-\frac{2\pi}{k})$, we need to plot a few representative particle trajectories.
The particle trajectory can be obtained by integrating the velocity vector along the path. For small amplitude waves, we can assume that the fluid motion is irrotational, and thus the streamlines are parallel to the velocity vector. Therefore, the particle trajectory will be perpendicular to the streamline at each point.
Let’s start with the point $(0,0)$. The velocity vector at this point is given by $(u,v)=(A\omega,0)$, so the streamline will be a horizontal line. The particle trajectory passing through $(0,0)$ will be a vertical line, as shown below:
Now, let’s consider the point $(0,-\frac{2\pi}{k})$. The velocity vector at this point is given by $(u,v)=(0,A\omega e^{-2\pi})$. Since $v$ is negative, the particle will move downwards along the streamline. The streamline itself can be obtained by integrating the velocity vector, which gives:
$\frac{d y}{d x}=\frac{v}{u}=-\frac{e^{-2 \pi}}{k}$
Integrating this expression yields:
$y=-\frac{e^{-2 \pi}}{k} x+C$
where $C$ is a constant. We can determine $C$ by setting $x=0$ and $y=-\frac{2\pi}{k}$, which gives $C=-\frac{2\pi}{k}$. Therefore, the streamline passing through $(0,-\frac{2\pi}{k})$ is given by:
$y=-\frac{e^{-2 \pi}}{k} x-\frac{2 \pi}{k}$
The particle trajectory passing through $(0,-\frac{2\pi}{k})$ will be perpendicular to this streamline and can be obtained by integrating the velocity vector along the streamline. The resulting particle trajectories are shown below:
b) Sketch the particle line at $\mathrm{t}=\frac{2 \pi}{\omega}$ for the particle which was at $\mathrm{x}=\mathrm{o}, \mathrm{y}=\mathrm{o}$ at $\mathrm{t}=\mathrm{o}$
b) To sketch the particle line at $t=\frac{2\pi}{\omega}$ for the particle which was at $(x,y)=(0,0)$ at $t=0$, we need to find the position of the particle at $t=\frac{2\pi}{\omega}$.
The particle position can be obtained by integrating the velocity vector along the particle trajectory. For a particle starting at $(0,0)$, the particle trajectory is a vertical line, as shown in part (a). The velocity vector along this trajectory is given by $(u,v)=(A\omega e^{ky},0)$. Integrating this expression from $y=0$ to $y=-\frac{2\pi}{k}$ and from $t=0$ to $t=\frac{2\pi}{\omega}$ gives:
$x=0 \quad$ and $\quad y=-\frac{2 \pi}{k}$
Therefore, the particle that was at $(x,y)=(0,0)$ at $t=0$ will be at $(x,y)=(0,-\frac{2\pi}{k})$ at $t=\frac{2\pi}{\omega}$
For an ideal rectilinear vortex, the velocity profile is given by $\mathrm{v}_{\mathrm{r}}=\mathrm{v}_{\mathrm{z}}=0 ; \mathrm{v}_\theta=\mathrm{K} / \mathrm{r}$. $A$ qualitatively similar result is associated with an ideal smoke ring. a) Why does a smoke ring propel itself? Why does the velocity of translation diminish with time?
A smoke ring is a toroidal vortex ring that propagates through the air. It is created by a sudden release of fluid, such as smoke, from a circular orifice. The smoke ring propels itself because it is a closed loop of fluid that has rotational motion. The fluid inside the smoke ring rotates in the same direction as the ring itself, creating a low-pressure area in the center of the ring.
As the smoke ring moves forward, the low-pressure area at the center of the ring pulls in air from behind, which helps to maintain the shape of the ring. This process of entraining air into the ring creates a drag force, which slows down the velocity of the smoke ring over time.
The velocity of translation of the smoke ring diminishes with time because of the loss of energy due to drag forces. As the smoke ring moves through the air, it creates turbulence and friction with the surrounding air, which causes the fluid within the ring to lose energy. This loss of energy translates into a decrease in the velocity of translation of the smoke ring over time. Eventually, the smoke ring will come to a stop as all of its kinetic energy is dissipated through friction with the air.