这是一份 Imperial帝国理工大学 PHYS96018作业代写的成功案例

To begin our analysis it is helpful to consider a simpler problem, i.e. how to solve the linear equation
$$
\frac{\mathrm{d} \varphi}{\mathrm{d} t}=A \varphi
$$
on a finite-dimensional vector space $V$. If the matrix $A$ is diagonalizable and all eigenvalues are simple (i.e. without degeneracy), the space $V$ has a basis of right eigenvectors $\left{v_{j}\right}$ :
$$
A v_{j}=\varepsilon_{j} v_{j}
$$
$$
\varphi(0)=\sum_{k=1}^{N} B_{k} v_{k},
$$
where the coefficients of linear combination are obtained by using the left eigenvectors $v^{j}$ satisfying
$$
v^{j} A=\varepsilon_{j} v^{j}
$$
in the form
$$
B_{j}=\left(v^{j}, \varphi(0)\right)
$$

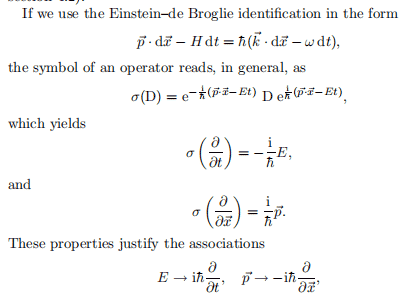
PHYS96018 COURSE NOTES :
The operator of the electric dipole moment reads $d=-e x$, or, upon transformation to a spherical basis, defined by $d_{\pm 1}=\mp\left(d_{1} \pm i d_{2}\right) / \sqrt{2}$, $d_{0}=d_{3}$,
$d_{\mu}=-e \sqrt{\frac{4 \pi}{3}} r Y_{1 \mu} .$
Converting the field $\boldsymbol{E}$ to the same basis,
$$
E_{+1}=-\frac{1}{\sqrt{2}}\left(E_{1}+\mathrm{i} E_{2}\right), \quad E_{-1}=\frac{1}{\sqrt{2}}\left(E_{1}-\mathrm{i} E_{2}\right), \quad E_{0}=E_{3},
$$
the scalar product becomes
$$
\boldsymbol{d} \cdot \boldsymbol{E}=\sum_{\mu} d_{\mu}^{} E_{\mu}=\sum_{v} d_{v} E_{v}^{} .
$$