这是一份umass麻省大学 MATH 497K作业代写的成功案例
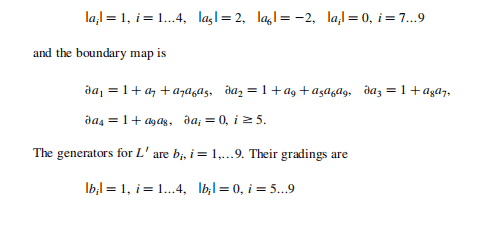
Deform spinning is another construction that takes $n$-knots to $n+1$ knots. The tersest description of deform spinning comes from once again thinking of a simple spin as a special case of a frame spin, i.e., as
$$
\left[\left(S^{n+1}, S^{n-1}\right)-S^{1} \times\left(B^{n}, B^{n-2}\right)\right] \bigcup_{a}\left[S^{1} \times\left(B_{K}^{n}, B_{K}^{n-2}\right)\right]
$$
where $\cup_{a}$ indicates gluing along the common boundary in the obvious (untwisted) fashion. Suppose now that we have a 1-parameter family $f_{\psi}$ of deformations of $B_{K}^{n}$ rel $\partial B_{K}^{n}$ such that $f_{0}$ is the identity and $f_{2} \pi^{n}\left(B_{K}^{n-2}\right)=B_{K}^{n-2}$. The family $f_{\psi}$ should also depend piecewise linearly on the parameter $\psi$. Litherland then describes the deform spin of $K$ as
$$
\left[\left(S^{n+1}, S^{n-1}\right)-S^{1} \times\left(B^{n}, B^{n-2}\right)\right] \bigcup_{a}\left(S^{1} \times B_{K}^{n}, \bigcup_{\psi \in S^{1}} \psi \times f_{\psi}\left(B_{K}^{n-2}\right)\right)
$$

MATH 497K COURSE NOTES :
Litherland also shows that, thinking of the collection $f_{\psi}$ as a PL map
$$
f: B_{K}^{n} \times[0,2 \pi] \rightarrow B_{K}^{n} \times[0,2 \pi],
$$
the type of the deform spun knot is dependent only upon the pseudo-isotopy class of $f$ rel $\partial B_{K}^{r}$ as a map of pairs ( $f$ and $g$ are pseudo-isotopic rel $\partial B_{K}^{n}$ as maps of pairs if there is a PL homeomorphism
$$
H:\left(B_{K}^{n}, B_{K}^{n-2}\right) \times[0,2 \pi] \rightarrow\left(B_{K}^{n}, B_{K}^{n-2}\right) \times[0,2 \pi]
$$
such that $\left.H\right|{B{K}^{n} \times 0}$ and $\left.H\right|{a B{K}^{n} \times(0,2 \pi]}$ are the identity maps and $\left.\left.H\right|{B{E}^{n} \times 2 \pi}=f_{2 \pi} g_{2 \pi}^{-1}\right)$.