Assignment-daixieTM为您提供伦敦大学学院 London’s Global University MATH0016 Mathematical Methods 3数学方法代写代考和辅导服务!
Instructions:
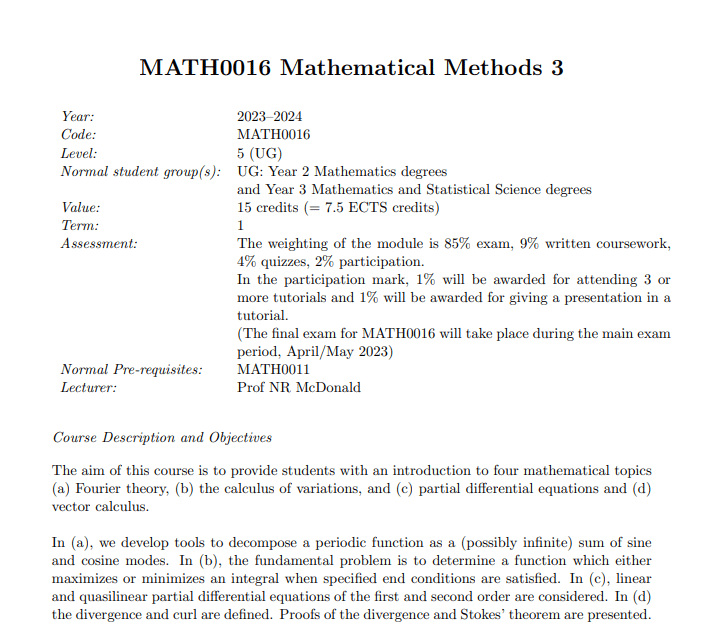
To summarize, the course covers the following topics:
(a) Fourier theory: This topic deals with decomposing a periodic function into a sum of sine and cosine functions, which can help analyze and understand the behavior of the function over time. The Fourier transform is a powerful tool used in signal processing, image analysis, and many other fields.
(b) The calculus of variations: This is a branch of mathematics that deals with finding functions that maximize or minimize an integral, subject to certain conditions. This topic has applications in many fields, including physics, engineering, and economics.
(c) Partial differential equations: These are equations that involve partial derivatives of a function, and they are commonly used to describe physical phenomena such as fluid dynamics and heat transfer. The course will cover both linear and quasilinear partial differential equations of the first and second order.
(d) Vector calculus: This topic deals with the manipulation of vector fields, including the divergence and curl operations. These operations are useful in many areas of physics and engineering, including electromagnetism and fluid dynamics. The course will also cover the divergence and Stokes’ theorem.
I hope this helps clarify what you’ll be studying in the course! Let me know if you have any questions or if there’s anything else I can help you with.
Solve the initial value problem
$$
y^{\prime} y^{\prime \prime}-t=0, \quad y(1)=2, \quad y^{\prime}(1)=1 .
$$
We can begin by using the method of integration by parts to integrate $y’y”$. Let $u = y’$ and $v’ = y”$, so that $u’ = y”$ and $v = y’$. Then we have
$y^{\prime} y^{\prime \prime}=u v^{\prime}=\frac{1}{2}\left(u^2\right)^{\prime}$.
Using this, we can rewrite the differential equation as
$\frac{1}{2}\left(y^{\prime 2}\right)^{\prime}-t=0$
Integrating once with respect to $t$, we get
$\frac{1}{2} y^{\prime 2}-\frac{1}{2} t^2=C_1$,
where $C_1$ is a constant of integration. We can then solve for $y’$ to get
$y^{\prime}= \pm \sqrt{t^2+2 C_1}$.
Using the initial condition $y(1) = 2$, we get
$y^{\prime}(1)= \pm \sqrt{1+2 C_1}=1$
Solving for $C_1$, we find that $C_1 = 0$. Thus, we have
$y^{\prime}= \pm \sqrt{t^2}, \quad y(1)=2$.
Since $y$ is increasing, we take the positive sign, giving
$y^{\prime}=t, \quad y(1)=2$.
Integrating with respect to $t$, we get
$y=\frac{1}{2} t^2+C_2$.
Using the initial condition $y(1) = 2$, we get $C_2 = \frac{3}{2}$, so the solution to the initial value problem is
$y=\frac{1}{2} t^2+\frac{3}{2}$
Consider the differential equation $y^{\prime}=y(5-y)(y-4)^2$. (a)Determine the critical points (stationary solutions).
To determine the critical points, we need to find the values of $y$ for which $y’=0$. Thus, we solve the equation:
$y^{\prime}=y(5-y)(y-4)^2=0$
This equation is satisfied when $y=0,5$, or $4$. Therefore, the critical points are $y=0,5,$ and $4$.
(b)Sketch the graph of $f(y)=y(5-y)(y-4)^2$.
To sketch the graph of $f(y)=y(5-y)(y-4)^2$, we can use the critical points found in part (a) and the behavior of $f(y)$ as $y$ approaches positive or negative infinity.
First, we note that $f(y)$ changes sign at $y=0,4,$ and $5$. We can determine the sign of $f(y)$ on each interval by testing a point in each interval. For example, for $y<0$, we can test $y=-1$, and we get:
$f(-1)=(-1)(5-(-1))(4-(-1))^2=-120<0$.
Therefore, $f(y)$ is negative on $(-\infty,0)$.
Next, we can consider the behavior of $f(y)$ as $y$ approaches infinity or negative infinity. Since the highest power of $y$ in $f(y)$ is $y^4$, we know that $f(y)$ approaches infinity as $y$ approaches positive or negative infinity.
Putting all of this together, we can sketch the graph of $f(y)$ as follows:
The graph has a local maximum at $y=4$ and a local minimum at $y=5$. The critical point $y=0$ is a double root.
