这是一份 Imperial帝国理工大学 PHYS96031作业代写的成功案例
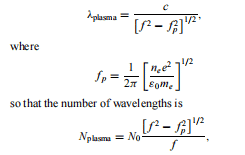
note that the electric field at a location $\mathbf{x}$ due to a charge $q$ at position $\mathbf{x}{1}$ is $$ \begin{aligned} \mathbf{E}\left(\mathbf{x}, \mathbf{x}{1}\right) &=\frac{q}{4 \pi \varepsilon_{0}} \frac{\mathbf{x}-\mathbf{x}{1}}{\left|\mathbf{x}-\mathbf{x}{1}\right|^{3}} \
&=-\frac{q}{\varepsilon_{0}} \int \frac{i \mathbf{k}}{k^{2}} e^{i k \cdot\left(\mathbf{x}-\mathbf{x}_{1}\right)} \frac{d^{3} k}{(2 \pi)^{3}}
\end{aligned}
$$
as long as the distance between $\mathbf{x}$ and $\mathbf{x}{1}$ is less than a Debye length, so negligible shielding occurs. [The validity of the Fourier transform can be demonstrated using $\left.\nabla \cdot \mathbf{E}=\left(e / \varepsilon{0}\right) \delta\left(\mathbf{x}-\mathbf{x}{1}\right)\right]$. The spatially fluctuating electric field is $$ \begin{aligned} \tilde{E}^{2} & \equiv \int n d^{3} x{1} \mathbf{E}\left(\mathbf{x}, \mathbf{x}{1}\right) \cdot \mathbf{E}\left(\mathbf{x}, \mathbf{x}{1}\right) \
&=n\left(\frac{q}{\varepsilon_{0}}\right)^{2} \int \frac{1}{k^{2}} \frac{d^{3} k}{(2 \pi)^{3}}
\end{aligned}
$$

PHYS96031 COURSE NOTES :
If the magnetic field is toroidally symmetric, it can be written in a hybrid covariant-contravariant form using $(R, \varphi, Z)$ cylindrical coordinates,
$$
\mathbf{B}=\mu_{0} G\left(\psi_{\mathrm{p}}\right) \nabla \varphi+\nabla \varphi \times \nabla \psi_{\mathrm{p}} .
$$
The curl of this expression gives the current $\mathbf{j}=-\nabla \varphi \times$ $\nabla \mathrm{G}+\left(\Delta_{} \psi_{\mathrm{p}}\right) \nabla \varphi / \mu_{0}$ with the Grad-Shafranov operator defined by $$ \Delta_{} \psi_{\mathrm{p}} \equiv R \frac{\partial}{\partial R}\left(\frac{1}{R} \frac{\partial \psi_{\mathrm{p}}}{\partial R}\right)+\frac{\partial^{2} \psi_{\mathrm{p}}}{\partial Z^{2}} .
$$
The equilibrium equation, $\nabla p=\mathbf{j} \times \mathbf{B}$, then yields the Grad-Shafranov equation for toroidally symmetric equilibria,
$$
\nabla_{*} \psi_{\mathrm{p}}=-\mu_{0}\left(\mu_{0} G \frac{d G}{d \psi_{\mathrm{p}}}+(2 \pi R)^{2} \frac{d p}{d \psi_{\mathrm{p}}}\right) .
$$
The pressure and the poloidal current outside at constant$\psi_{p}$ surface are the natural functions for defining the equilibrium in a Grad-Shafranov solver.