Tag Archives: Real Analysis代写

实分析 Real Analysis MAT00005C

0
Posted on by

这是一份YORK约克大学MAT00004C作业代写的成功案例

实分析 Real Analysis MAT00005C
问题 1.

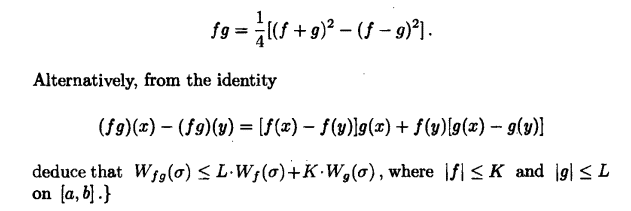
{Condition (b) is Riemann’s original formulation of integrability. 1 }
(a) $\Rightarrow$ (b): Assuming $f$ is Riemann-integrable, let
$$
\lambda=\int_{a}^{b} f
$$

证明 .

For each $\nu=1, \ldots, n$, choose a sequence $\left(x_{\nu}^{k}\right)$ in $\left[a_{\nu-1}, a_{\nu}\right]$ such that
$$
f\left(x_{\nu}^{k}\right) \rightarrow M_{\nu} \text { as } k \rightarrow \infty
$$
then
$$
\sum_{\nu=1}^{n} f\left(x_{\nu}^{k}\right) e_{\nu} \rightarrow \sum_{\nu=1}^{n} M_{\nu} e_{\nu}=S(\sigma)
$$
so by $()$ we have (*)
$$
\lambda-\epsilon \leq S(\sigma) \leq \lambda+\epsilon .
$$

英国论文代写Viking Essay为您提供作业代写代考服务

MAT00005C COURSE NOTES ：

Similarly,
$$
\lambda-\epsilon \leq s(\sigma) \leq \lambda+\epsilon \text {; }
$$
thus $S(\sigma)$ and $s(\sigma)$ both belong to the interval $[\lambda-\epsilon, \lambda+\epsilon]$, therefore
$$
W_{f}(\sigma)=S(\sigma)-s(\sigma) \leq 2 \epsilon .
$$
This proves that $f$ is Riemann-integrable and since
$$
S(\sigma) \rightarrow \int_{a}^{b} f \text { as } \mathrm{N}(\sigma) \rightarrow 0,
$$
it is clear from $\left({ }^{* *}\right)$ that
$$
\lambda=\int_{a}^{b} f . \diamond
$$








实分析 Real Analysis MATH20101

0
Posted on by

这是一份manchester帝国理工大学 GG14作业代写的成功案例

实分析 Real Analysis MATH20101
问题 1.

conclusion holds for $k=1$. Let us proceed by induction on $k$. If $C$ has no interior, then by Theorem $6.2 .6$ it is included in a hyperplane $H=f^{-1}{c}$ for some non-zero linear form $f$ and $c \in \mathbb{R}$. Then
$$
H={x: f(x) \geq c} \cap{x: f(x) \leq c},
$$
an intersection of two half-spaces. Also,
$$
H=u+V:={u+v: v \in V}
$$


证明 .

Let $V$ be a real vector space and $C$ a convex set in $V$. A real-valued function $f$ on $C$ is called convex iff
$$
f(\lambda x+(1-\lambda) y) \leq \lambda f(x)+(1-\lambda) f(y)
$$


英国论文代写Viking Essay为您提供作业代写代考服务

MATH20101 COURSE NOTES ：

$$
t \leq 3 \sum_{i=1}^{q} \lambda\left(J_{i}\right) \leq 6 j \sum_{i=1}^{q} \mu\left(J_{i}\right) \leq 6 j \mu(V) \leq 6 j \varepsilon .
$$
Thus $\lambda\left(P_{j}\right) \leq 6 j \varepsilon$. Letting $\varepsilon \downarrow 0$ gives $\lambda\left(P_{j}\right)=0, j=1,2, \ldots$, and letting $j \rightarrow \infty$ proves the lemma.
Now to prove Theorem 7.2.1, for each rational $r$ let
$$
g_{r}:=\max (g-r, 0), \quad f_{r}(x):=\int_{a}^{x} g_{r}(t) d t .
$$







实分析| Real Analysis代写 MT3502

0
Posted on by

这是一份andrews圣安德鲁斯大学 MT3502作业代写的成功案例

实分析| Real Analysis代写 MT3502
问题 1.

and $c_{n} \rightarrow a, d_{n} \rightarrow b$; then
$$
F\left(d_{n}\right)-F\left(c_{n}\right)=H\left(d_{n}\right)-H\left(c_{n}\right)
$$
for all $n$, so in the limit we have
$$
F(b)-0=H(b)-0
$$


证明 .

by the continuity of $F$ and In other words,
$$
\int_{a}^{b} f=\int_{a}^{b} f \cdot \diamond
$$


英国论文代写Viking Essay为您提供作业代写代考服务

MT3502 COURSE NOTES ：

Let $F:[a, b] \rightarrow \mathbb{R}$ and $G:[a, b] \rightarrow \mathbb{R}$ be the indefinite upper integrals of $f$ and $g$, respectively:
$$
F(x)=\int_{a}^{-x} f \text { and } G(x)=\int_{a}^{-x} g
$$
for $a \leq x \leq b$. If $a<c<d<b$ then, as in the proof of $9.6 .3$,
$$
\begin{aligned}
&F(d)=F(c)+\int_{c}^{-d} f, \
&G(d)=G(c)+\int^{-d} g
\end{aligned}
$$





实分析|Real Analysis  5CCM221A

0
Posted on by

这是一份KCL伦敦大学 5CCM221A作业代写的成功案例

实分析|Real Analysis  5CCM221A
问题 1.


Proof. Let $h_{n} \rightarrow 0, h_{n} \neq 0$. Since $E$ is continuous, $E\left(h_{n}\right) \rightarrow E(0)=$ 1. Let $x_{n}=E\left(h_{n}\right)$; then $x_{n}>0, x_{n} \rightarrow 1$ and $x_{n} \neq 1$ (because $\left.h_{n} \neq 0\right)$, so by $9.5 .10$
$$
\frac{L\left(x_{n}\right)}{x_{n}-1} \rightarrow 1
$$


证明 .

That is,
$$
\frac{h_{n}}{E\left(h_{n}\right)-1} \rightarrow 1
$$
whence the lemmn.

英国论文代写Viking Essay为您提供作业代写代考服务

5CCM221A COURSE NOTES ：


(i) Show that $A$ is an odd function: $A(-x)=-A(x)$ for all $x \in \mathbb{R}$.
{Hint: $f$ is an even function.}
(ii) $A$ is strictly increasing. {Hint: $\left.A^{\prime}=f .\right}$
(iii) For every positive integer $k$,
$$
\frac{1}{1+k^{2}} \leq A(k)-A(k-1) \leq \frac{1}{1+(k-1)^{2}} .
$$
{Hint: Integrate $f$ over the interval
(iv) Let
$$
s_{n}=\sum_{k=1}^{n} \frac{1}{1+k^{2}} .
$$





实分析|Real Analysis代写 MATH 3150

0
Posted on by

这是一份northeastern东北大学(美国)  MATH 3150作业代写的成功案例

实分析|Real Analysis代写 MATH 3150
问题 1.

Proof. Write $h=g \circ f .$ By 8.1.5, there exists a function $A: S \rightarrow \mathbb{R}$, continuous at $c$, such that
$$
f(x)-f(c)=A(x)(x-c) \text { for all } x \in \mathbf{S}
$$
Similarly, there is a function $B: T \rightarrow \mathbb{R}$, continuous at $f(c)$, such that

证明 .

(2) $g(y)-g(f(c))=B(y)(y-f(c))$ for all $y \in \mathrm{T}$.
If $s \in \mathrm{S}$ then $f(x) \in \mathrm{T}$; putting $y=f(x)$ in (2), we have
$$
\begin{aligned}
g(f(x))-g(f(c)) &=B(f(x)) \cdot(f(x)-f(c)) \
&=B(f(x)) \cdot A(x)(x-c)
\end{aligned}
$$

英国论文代写Viking Essay为您提供实分析作业代写Real anlysis代考服务

MATH 3150COURSE NOTES ：

$$
\sigma={a<b}, \quad \tau \doteq{a<c<b}
$$
Writing $M=\sup f$ as before, and
$$
\begin{aligned}
M^{\prime} &=\sup {f(x): a \leq x \leq c}, \
M^{\prime \prime} &=\sup {f(x): c \leq x \leq b},
\end{aligned}
$$
we have
$$
S(\sigma)=M(b-a) \quad \text { and } \quad S(\tau)=M^{\prime}(c-a)+M^{\prime \prime}(b-c)
$$