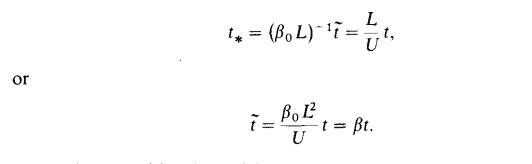
$$
\frac{d}{d t}\left(\int_{\mathrm{CV}} \rho d V\right)-\rho_{1} A_{1} V_{1}-\rho_{2} A_{2} V_{2}=0
$$
Now if $A_{t}$ is the tank cross-sectional area, the unsteady term can be evaluated as follows:
$$
\frac{d}{d t}\left(\int_{\mathrm{CV}} \rho d V\right)=\frac{d}{d t}\left(\rho_{\mathrm{w}} A_{t} h\right)+\frac{d}{d t}\left[\rho_{a} A_{r}(H-h)\right]=\rho_{w} A_{t} \frac{d h}{d t}
$$
The $\rho_{a}$ term vanishes because it is the rate of change of air mass and is zero because the air is trapped at the top. Substituting we find the change of water height
$$
\frac{d h}{d t}=\frac{\rho_{1} A_{1} V_{1}+\rho_{2} A_{2} V_{2}}{\rho_{w} A_{t}}
$$
Ans. (a)
For water, $\rho_{1}=\rho_{2}=\rho_{\mathrm{w}}$, and this result reduces to
$$
\frac{d h}{d t}=\frac{A_{1} V_{1}+A_{2} V_{2}}{A_{t}}=\frac{Q_{1}+Q_{2}}{A_{t}}
$$

MATH4074 COURSE NOTES :
applies with one inlet and exit:
$$
\sum \mathbf{F}=m_{2} \mathbf{V}{2}-\dot{m}{1} \mathbf{V}{1}=\left(\rho{2} A_{2} V_{2}\right) \mathbf{V}{2}-\left(\rho{1} A_{1} V_{1}\right) \mathbf{V}{1} $$ The volume integral term vanishes for steady flow, but from conservation of mass in Example $3.3$ we saw that $$ \dot{m}{1}=\dot{m}{2}=\dot{m}=\text { const } $$ Therefore a simple form for the desired result is $$ \sum \mathbf{F}=m\left(\mathbf{V}{2}-\mathbf{V}_{1}\right)
$$
Ans.
This is a vector relation and is sketched.The term $\Sigma$ F represents the net force acting on the control volume due to all causes; it is needed to balance the change in momentum of the fluid as it tums and decelerates while passing through the control volume.