$$
z^{\prime}=15\left(\frac{51}{23}\right)-5\left(\frac{58}{23}\right)+10\left(\frac{9}{23}\right)=\frac{565}{23} \text {. }
$$
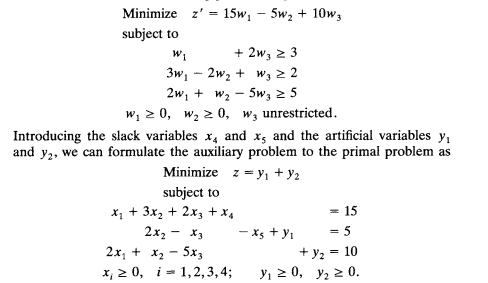
We see from Tableau $3.18$ that an optimal solution to the primal problem is
$$
x_{1}=\frac{120}{23}, \quad x_{2}=\frac{65}{23}, \quad x_{3}=\frac{15}{23}
$$
and the value of the objective function is $\frac{565}{23}$, which is the same as the value for the dual problem.
We can also solve the primal problem using the big $M$ method, obtaining the final tableau (Tableau 3.20). Using (2) we can obtain an optimal solution to the dual problem from Tableau $3.20$ as follows. Since the first initial basic variable is $x_{4}$, we then find
$$
w_{1}=\hat{w}{1}=c{4}+\left(z_{4}-c_{4}\right)=0+\frac{51}{23}=\frac{51}{23} .
$$
The second initial basic variable is $y_{1}$, so that
$$
\hat{w}{2}=-M+\left(M-\frac{58}{23}\right)=-\frac{58}{23} . $$ But since the second constraint was multiplied by $-1$ in forming the dual problem, we must multiply $\hat{w}{2}$ by $-1$ to obtain the value of the dual variable at the optimum. We have
$$
w_{2}=-\hat{w}{2}=\frac{58}{23} \text {. } $$ Proceeding as in the case of the first dual variable, we find $$ w{3}=\hat{w}_{3}=-M+M+\frac{9}{23}=\frac{9}{23} .
$$
Substituting these values into the objective function, we get
$$
z^{\prime}=15\left(\frac{51}{23}\right)-5\left(\frac{58}{23}\right)+90\left(\frac{9}{23}\right)=\frac{565}{23}
$$

STAT0025 COURSE NOTES :
- Maximize $z=2 x_{1}+x_{2}+3 x_{3}$ subject to
$$
\begin{gathered}
2 x_{1}-x_{2}+3 x_{3} \leq 6 \
x_{1}+3 x_{2}+5 x_{3} \leq 10 \
2 x_{1} \quad+x_{3} \leq 7 \
x_{1} \geq 0, \quad x_{2} \geq 0, \quad x_{3} \geq 0
\end{gathered}
$$ - Maximize $z=x_{1}+x_{2}+x_{3}+x_{4}$ subject to
$$
\begin{gathered}
x_{1}+2 x_{2}-x_{3}+3 x_{4} \leq 12 \
x_{1}+3 x_{2}+x_{3}+2 x_{4} \leq 8 \
2 x_{1}-3 x_{2}-x_{3}+2 x_{4} \leq 7 \
x_{1} \geq 0, \quad x_{2} \geq 0, \quad x_{3} \geq 0, \quad x_{4} \geq 0 .
\end{gathered}
$$