这是一份kcl伦敦大学学院 6CCM326A作业代写的成功案
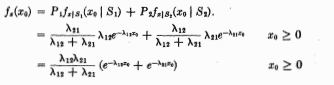
$$
b^{\prime}=a^{\prime} \sum_{i=1}^{p-1} \zeta^{i}=((p-3) / 4) \sum_{i=1}^{p-1} \zeta^{i}=((p-3) / 4)(-1)=-(p-3) / 4
$$
and then
$$
b=(p-1) / 2+b^{\prime}=(p-1) / 2-(p-3) / 4=(p+1) / 4
$$
Now
$$
\begin{aligned}
\left(X-\alpha_{0}\right)\left(X-\alpha_{1}\right) &=X^{2}-\left(\alpha_{0}+\alpha_{1}\right) X+\alpha_{0} \alpha_{1} \
&=X^{2}+X+b,
\end{aligned}
$$
so
$$
\begin{array}{ll}
\left(X-\alpha_{0}\right)\left(X-\alpha_{1}\right)=X^{2}+X-(p-1) / 4 & \text { if } p \equiv 1(\bmod 4) \
\left(X-\alpha_{0}\right)\left(X-\alpha_{1}\right)=X^{2}+X+(p+1) / 4 & \text { if } p \equiv 3(\bmod 4)
\end{array}
$$

6CCM326ACOURSE NOTES :
$$
\theta=\alpha_{1} Y_{1}+\cdots+\alpha_{n} Y_{n} \in \tilde{\mathbf{E}}
$$
and let
$$
F(Z)=\prod_{\tau^{\prime} \in S_{n}}\left(Z-\tau^{\prime}(\theta)\right) \in \tilde{\mathbf{E}}[Z]
$$
The polynomial $F(Z)$ is a symmetric function of its roots, so by Lemma its coefficients are functions of the elementary symmetric functions of its roots and hence of $Y_{1}, \ldots, Y_{n}$ and the coefficients of $f(X)$. Thus, $F(Z) \in$ $\tilde{\mathbf{F}}[Z]$. Now we may factor $F(Z)$ into a product of irreducibles in $\tilde{\mathbf{F}}[Z]$,
$$
F(Z)=F_{1}(Z) \cdots F_{t}(Z)
$$