这是一份BATH巴斯大学MA10212作业代写的成功案例
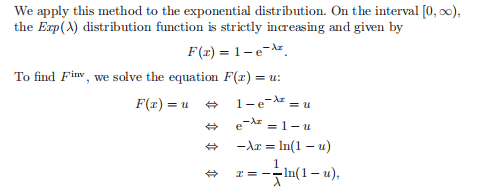
This point of view also leads the way to how one should define the expected value of a continuous random variable. Let, for example, $X$ be a continuous random variable whose probability density function $f$ is zero outside the interval $[0,1]$. It seems reasonable to approximate $X$ by the discrete random variable $Y$, taking the values
$$
\frac{1}{n}, \frac{2}{n}, \ldots, \frac{n-1}{n}, 1
$$
with as probabilities the masses that $X$ assigns to the intervals $\left[\frac{k-1}{n}, \frac{k}{n}\right]$ :
$$
\mathrm{P}\left(Y=\frac{k}{n}\right)=\mathrm{P}\left(\frac{k-1}{n} \leq X \leq \frac{k}{n}\right)=\int_{(k-1) / n}^{k / n} f(x) \mathrm{d} x
$$
We have a good idea of the size of this probability. For large $n$, it can be approximated well in terms of $f$ :
$$
\mathrm{P}\left(Y=\frac{k}{n}\right)=\int_{k / n-1 / n}^{k / n} f(x) \mathrm{d} x \approx \frac{1}{n} f\left(\frac{k}{n}\right) .
$$
The “center-of-gravity” interpretation suggests that the expectation $\mathrm{E}[Y]$ of $Y$ should approximate the expectation $\mathrm{E}[X]$ of $X$. We have
$$
\mathrm{E}[Y]=\sum_{k=1}^{n} \frac{k}{n} \mathrm{P}\left(Y=\frac{k}{n}\right) \approx \sum_{k=1}^{n} \frac{k}{n} f\left(\frac{k}{n}\right) \frac{1}{n}
$$
By the definition of a definite integral, for large $n$ the right-hand side is close to
$$
\int_{0}^{1} x f(x) \mathrm{d} x .
$$
This motivates the following definition.

MA10212 COURSE NOTES :
THE CHANGE-OF-VARIA BLE FORMULA. Let $X$ be a random variable, and let $g: \mathbb{R} \rightarrow \mathbb{R}$ be a function.
If $X$ is discrete, taking the values $a_{1}, a_{2}, \ldots$, then
$$
\mathrm{E}[g(X)]=\sum_{i} g\left(a_{i}\right) \mathrm{P}\left(X=a_{i}\right)
$$
If $X$ is continuous, with probability density function $f$, then
$$
\mathrm{E}[g(X)]=\int_{-\infty}^{\infty} g(x) f(x) \mathrm{d} x
$$