这是一份liverpool利物浦大学MATH349的成功案例

$$
\bar{x}^{1}=\frac{x^{1}-\beta c x^{4}}{\sqrt{1-\beta^{2}}} ; \quad \bar{x}^{2}=x^{2} ; \quad \bar{x}^{3}=x^{3} ; \quad \bar{x}^{4}=\frac{x^{4}-\beta x^{1} / c}{\sqrt{1-\beta^{2}}}
$$
Notice that solving the first equation for $x^{1}$ gives
$$
x^{1}=\bar{x}^{1} \sqrt{1-\beta^{2}}+\beta c x^{4} .
$$
Since $x^{4}$ is just time $t$ here, it means that the origin of the $\bar{x}$-system has coordinates $(\beta c t, 0$, 0 ) in terms of the original coordinates. In other words, it is moving in the $x$-direction with a velocity of
$$
v=\beta c
$$
so we must interpret $\beta$ as the speed in “warp;”
$$
\beta=\frac{v}{c} .
$$

MATH349 COURSE NOTES ：

$$
\frac{D X}{d t}=\pi \frac{d X}{d t}
$$
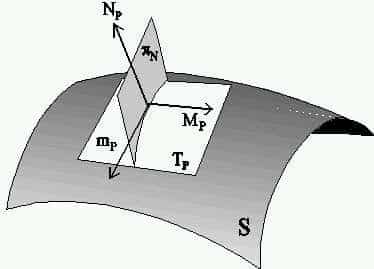
which, by the lemma, has local coordinates given by
$$
\frac{D X^{i}}{d t}=g^{i r}\left(\frac{d X}{d t} \cdot \frac{\partial}{\partial x^{r}}\right) .
$$
To evaluate the term in parentheses, we use ambeint coordinates. $d X / d t$ has ambient coordinates
$$
\frac{d}{d t}\left(X^{p} \frac{\partial y_{s}}{\partial x^{p}}\right)=\frac{d X^{p}}{d t} \frac{\partial y_{s}}{\partial x^{p}}+X^{p} \frac{\partial^{2} y_{s}}{\partial x^{p} \partial x^{q}} \frac{d x^{q}}{d t}
$$
Thus, dotting with $\partial / \partial x^{k}=\partial y_{s} / \partial x^{r}$ gives
$$
\frac{d X^{p}}{d t} \frac{\partial y_{s}}{\partial x^{p}} \frac{\partial y_{s}}{\partial x^{r}}+X^{p} \frac{\partial^{2} y_{s}}{\partial x^{p} \partial x^{q}} \frac{\partial y_{s}}{\partial x^{r}} \frac{d x^{q}}{d t}
$$