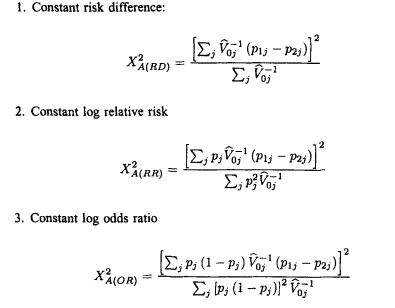Tag Archives: umass代写

应用统计学的方法|Methods of Applied Statistics代写 STAT 501

0
Posted on by

这是一份umass麻省大学 STAT 501作业代写的成功案例

应用统计学的方法|Methods of Applied Statistics代写 STAT 501
问题 1.

Let $X_{1}, X_{2}, X_{3}, \ldots$ be a sequence of mutually independent random variables, each with the same distribution as $X$. Two related sequences are of interest:

  1. The sequence of running sums:
    $$
    S_{m}=\sum_{i=1}^{m} X_{i} \text { for } m=1,2,3, \ldots
    $$

证明 .

  1. The sequence of running averages:
    $$
    \bar{X}{m}=\frac{1}{m} \sum{i=1}^{m} X_{i} \text { for } m=1,2,3, \ldots
    $$


英国论文代写Viking Essay为您提供作业代写代考服务

STAT501 COURSE NOTES ：

Using complements, it is sufficient to demonstrate that
$$
\lim {m \rightarrow \infty} P\left(\mu-\epsilon<\bar{X}{m}<\mu+\epsilon\right)=1
$$
Since $S D\left(\bar{X}{m}\right)=\sigma / \sqrt{m}$, if $\epsilon=k \sigma / \sqrt{m}$ (correspondingly, $k=\epsilon \sqrt{m} / \sigma$ ) is substituted into the Chebyshev inequality, then we get the following lower bound on probability: $$ P\left(\mu-\epsilon<\bar{X}{m}<\mu+\epsilon\right) \geq 1-\frac{1}{k^{2}}=1-\frac{\sigma^{2}}{\epsilon^{2} m}
$$
As $m \rightarrow \infty$, the quantity on the right in (ii) approaches 1 , implying that the limit in (i) is greater than or equal to 1 . Since probabilities must be between 0 and 1 , the limit must be exactly $1 .$





基本概念/统计学|Fundamental Concepts/Stats代写 STAT 310

0
Posted on by

这是一份umass麻省大学 STAT 310作业代写的成功案例

基本概念/统计学|Fundamental Concepts/Stats代写 STAT 310
问题 1.

$$
L(\pi)=\left(\begin{array}{c}
N \
x
\end{array}\right) \pi^{x}(1-\pi)^{N-x}
$$
and the log likelihood is
$$
\ell(\pi)=\log \left(\begin{array}{c}
N \
x
\end{array}\right)+x \log \pi+(N-x) \log (1-\pi) .
$$
Thus the total score is
$$
U(\pi)=\frac{d \ell}{d \pi}=\frac{x}{\pi}-\frac{(N-x)}{1-\pi}=\frac{x-N \pi}{\pi(1-\pi)} .
$$

证明 .

When set equal to zero, the solution yields the $M L E$ :
$$
\widehat{\pi}=x / N=p .
$$
The observed information is
$$
i(\pi)=\frac{-d^{2} \ell}{d \pi^{2}}=\frac{x}{\pi^{2}}+\frac{(N-x)}{(1-\pi)^{2}}
$$
Since $E(x)=N \pi$, the expected information is
$$
I(\pi)=E[i(\pi)]=\frac{E(x)}{\pi^{2}}+\frac{E(N-x)}{(1-\pi)^{2}}=\frac{N}{\pi(1-\pi)}
$$


英国论文代写Viking Essay为您提供作业代写代考服务

STAT 310 COURSE NOTES ：

Now consider the score test for $H_{0}: \beta=0$ in the conditional logit model. It is readily shown that
$$
U(\beta){\mid \beta=0}=(f-g) / 2 $$ and that $$ I(\beta){\mid \beta=0}=\frac{E(M)}{4} \cong \frac{M}{4}
$$
Therefore, the efficient score test is
$$
X^{2}=\frac{(f-g)^{2}}{M}
$$
which is McNemar’s test.





随机过程和模拟|Stochastic Processes and Simulations代写 MATH 597U

0
Posted on by

这是一份umass麻省大学 MATH 597U作业代写的成功案例

随机过程和模拟|Stochastic Processes and Simulations代写 MATH 597U
问题 1.

Note that $S_{t}$ and $\phi$ commute for each $t$, since $C_{t}^{}$ commutes with its generator $Y^{}$ and $C_{t}$ with $Y$. Now, using the perturbation expansion
$$
T_{, r}(\rho)=S_{, t}(\rho)+\int_{0}^{t} T_{*, t-s} \circ J \circ S_{s}(\rho) d s,
$$

证明 .

we see that $T_{, t}(\rho) \geq S_{, t}(\rho)$ for positive $\rho$, and furthermore,
$$
\begin{aligned}
&\left|T_{, t}(\phi(\rho))-S_{, t}(\phi(\rho))\right|_{1} \
&\leq \int_{0}^{t}\left|T_{, t-s} \circ J \circ S_{, s}(\phi(\rho))\right|_{1} d s \
&\left.=\int_{0}^{t} | T_{, t-s} \circ J_{1} \circ S_{, s}(\rho)\right)\left|_{1} d s \leq 4 t\right| \rho |_{1}
\end{aligned}
$$


英国论文代写Viking Essay为您提供作业代写代考服务

MATH 597U COURSE NOTES ：

For a given completely positive map $T$ on $\mathcal{A}$, we formally define the Lindbladian
$$
\mathcal{L}=\sum_{k \in \mathbb{Z}^{d}} \mathcal{L}{k}, $$ where $$ \mathcal{L}{k}(x)=\tau_{k} \mathcal{L}{0}\left(\tau{-k} x\right) \text {, for all } x \in \mathcal{A},
$$
with
$$
\mathcal{L}{0}(x)=-\frac{1}{2}{T(1), x}+T(x) $$ and ${A, B}:=A B+B A$. In particular we consider the Lindbladian $\mathcal{L}$ for the completely positive map $$ T(x)=\sum{l=0}^{\infty} a(l)^{*} x a(l), \text { for all } x \in \mathcal{A},
$$





科学计算入门|Intr. Scientific Computing代写 MATH 551

0
Posted on by

这是一份umass麻省大学 MATH 551作业代写的成功案例

科学计算入门|Intr. Scientific Computing代写 MATH 551
问题 1.

The proof of this result is simple and instructive, so we sketch it here. If $x^{}$ is a fixed point, then for the error at the $k$ th iteration we have $$ e_{k+1}=x_{k+1}-x^{}=g\left(x_{k}\right)-g\left(x^{}\right) $$ By the Mean Value Theorem, there is a point $\theta_{k}$ between $x_{k}$ and $x^{}$ such that
$$
g\left(x_{k}\right)-g\left(x^{}\right)=g^{\prime}\left(\theta_{k}\right)\left(x_{k}-x^{}\right)
$$

证明 .

so that
$$
e_{k+1}=g^{\prime}\left(\theta_{k}\right) e_{k}
$$
We do not know the value of $\theta_{k}$, but if $\left|g^{\prime}\left(x^{}\right)\right|<1$, then by starting the iterations close enough to $x^{}$, we can be assured that there is a constant $C$ such that $\left|g^{\prime}\left(\theta_{k}\right)\right| \leq C<1$, for $k=0,1, \ldots$ Thus, we have
$$
\left|e_{k+1}\right| \leq C\left|e_{k}\right| \leq \cdots \leq C^{k}\left|e_{0}\right|
$$
and since $C^{k} \rightarrow 0$, then $\left|e_{k}\right| \rightarrow 0$ and the sequence converges.


英国论文代写Viking Essay为您提供作业代写代考服务

MATH 551 COURSE NOTES ：

Newton’s Method. We illustrate Newton’s method by again finding a root of the equation
$$
f(x)=x^{2}-4 \sin (x)=0 .
$$
The derivative of this function is given by
$$
f^{\prime}(x)=2 x-4 \cos (x),
$$
so that the iteration scheme is given by
$$
x_{k+1}=x_{k}-\frac{x_{k}^{2}-4 \sin \left(x_{k}\right)}{2 x_{k}-4 \cos \left(x_{k}\right)} .
$$





应用数学的线性代数|Linear Algebra for Applied Mathematics代写 MATH 545

0
Posted on by

这是一份umass麻省大学 MATH 545作业代写的成功案例

应用数学的线性代数|Linear Algebra for Applied Mathematics代写 MATH 545
问题 1.

$$
A^{(n-1)}=U
$$
Define
$$
Q=Q_{1}, \cdots Q_{n-1}, P=P_{n-1} P_{n-2} \ldots P_{1},
$$

证明 .

and
$$
L=P\left(M_{n-1} P_{n-1} \ldots M_{1} P_{\mathrm{i}}\right)^{-1} .
$$
Then it can he shown that
$$
P A Q=L U,
$$
where $P$ and $Q$ are both permutution matrices and $L$ is unit triangular and $U$ is upper triangular.


英国论文代写Viking Essay为您提供作业代写代考服务

MATH 545 COURSE NOTES ：

$$
A=\left(\begin{array}{cc}
0.00011 & 1 \
1 & 1
\end{array}\right) \text {. }
$$

  1. Gaussian elimination without pivoting gives
    $$
    A^{(1)}=U=\left(\begin{array}{cc}
    0.0001 & 1 \
    0 & -10^{4}
    \end{array}\right) \text {, }
    $$$\hat{\rho}=$ the growth factor $=10^{4}$.
  2. Gaussian elimination with partial pivoting yields
    $$
    \begin{gathered}
    A^{(1)}=U=\left(\begin{array}{ll}
    1 & 1 \
    0 & 1
    \end{array}\right), \
    \max \left|a_{i j}^{(1)}\right|=1, \max \left|a_{i j}\right|=1, \
    p=\text { the growth factor }=1 .
    \end{gathered}
    $$





金融数学入门|Intro to Mathematics of Finance代写 MATH 537

0
Posted on by

这是一份umass麻省大学 MATH 537作业代写的成功案例

金融数学入门|Intro to Mathematics of Finance代写 MATH 537
问题 1.

Suppose that $\phi \in S F(0)$. Then, since $\tilde{V}(\phi)$ is a supermartingale, we have
$$
x=V_{0}\left(\phi_{0}\right) \geq E^{\mu}\left(e^{-r T} V_{T}\left(\phi_{T}\right)\right)
$$
If, further, $\phi$ is an $\left(x, f_{T}\right)$-hedge, then
$$
x \geq E^{\mu}\left(e^{-r T} f_{T}\right) .
$$

证明 .

Consequently, the rational investment price $C\left(T, f_{T}\right)$ satisfies
$$
C\left(T, f_{T}\right) \geq E^{\mu}\left(e^{-r T} f_{T}\right) .
$$
$$
d S_{t}^{1}=S_{t}^{1}\left(\mu d t+\sigma d B_{t}\right),
$$
where $B$ is a standard Brownian motion under $P$. Write $S^{1}(\mu)$ for the solution of $(7.27)$. Then, from $(7.28)$, under the measure $P^{\mu}$, the process $S^{1}(\mu)$ satisfies
$$
d S_{t}^{1}=S_{t}^{1}\left(r d t+\sigma d W_{t}^{\mu}\right),
$$


英国论文代写Viking Essay为您提供作业代写代考服务

MATH537 COURSE NOTES ：

the rational price for the option $f$ is independent of $\mu$ and is given by
$$
C(T, f)=E\left(e^{-r T} f\left(S_{T}^{1}(r)\right)\right)
$$
where $S^{1}$ is the solution of
$$
d S_{t}^{1}=S_{t}^{1}\left(r d t+\sigma d W_{t}\right) .
$$
Here $W$ is a standard Brownian motion on $(\Omega, \mathcal{F}, P)$.
The wealth process of the corresponding minimal hedge is
$$
V_{t}\left(\phi_{t}^{*}\right)=E\left(e^{-r(T-t)} f\left(S_{T}^{1}(r)\right) \mid \mathcal{F}_{t}\right)
$$





非线性动力学|Nonlinear Dynamics代写 MATH 532H

0
Posted on by

这是一份umass麻省大学 MATH 532H作业代写的成功案例

非线性动力学|Nonlinear Dynamics代写 MATH 532H
问题 1.

(a) Perturbation associated with $\Delta \mathrm{h}{a \perp}^{\mathrm{dc}}\left(\omega{\mathrm{p}}=\omega\right)$ :
$$
\left(\begin{array}{c}
\tilde{b}{1} \ \tilde{b}{2}
\end{array}\right)=\Delta \mathrm{h}{a \perp}^{\mathrm{dc}}\left(\begin{array}{c} \cos \theta{0} \
\mathrm{i}
\end{array}\right) \mathrm{e}^{-\mathrm{i}\left(\phi_{0}+\psi_{\mathrm{d} c}\right)}
$$
(b) Perturbation associated with $\Delta \mathrm{h}{a \perp}^{\mathrm{rf}}\left(\omega{\mathrm{p}}=2 \omega\right)$ :
$$
\left(\begin{array}{l}
\tilde{b}{1} \ \tilde{b}{2}
\end{array}\right)=\Delta \mathrm{h}{a \perp}^{\mathrm{rf}}\left(\begin{array}{c} \cos \theta{0} \
\mathrm{i}
\end{array}\right) \mathrm{e}^{\mathrm{i}\left(\psi_{\perp}-\phi_{0}\right)}
$$

证明 .

(c) Perturbation associated with $\Delta \mathrm{h}{a z}^{\mathrm{rf}}\left(\omega{\mathrm{p}}=\omega\right)$ :
$$
\left(\begin{array}{l}
\tilde{b}{1} \ \tilde{b}{2}
\end{array}\right)=\Delta \mathrm{h}{a z}^{\mathrm{rf}}\left(\begin{array}{c} -\sin \theta{0} \
0
\end{array}\right) \mathrm{e}^{\mathrm{i} \psi{ }^{\mathrm{x}}}
$$
(d) Perturbation associated with $\Delta \kappa\left(\omega_{\mathrm{p}}=\omega\right)$ :
$$
\left(\begin{array}{l}
\tilde{b}{1} \ \tilde{b}{2}
\end{array}\right)=\Delta \kappa\left(\begin{array}{c}
\cos 2 \theta_{0} \
i \cos \theta_{0}
\end{array}\right) \mathrm{e}^{-\mathrm{i}\left(\phi_{0}+\psi_{\mathrm{AN}}\right)}
$$


英国论文代写Viking Essay为您提供作业代写代考服务

MATH 532H COURSE NOTES ：

Let us introduce the new amplitudes $\left(z_{q 1}, z_{q_{2}}\right)$ defined by the formula:
$$
\left(\begin{array}{l}
c_{q 1} \
c_{q 2}
\end{array}\right)=\Pi_{q}(t)\left(\begin{array}{l}
z_{q 1} \
z_{q 2}
\end{array}\right)
$$
By substituting this expression into Eq. and by taking account of Eq. one finds:
$$
\frac{\mathrm{d}}{\mathrm{d} t}\left(\begin{array}{l}
z_{q 1} \
z_{q 2}
\end{array}\right)=\Omega_{q}\left(\begin{array}{l}
z_{q 1} \
z_{q 2}
\end{array}\right),
$$
where $\Omega_{q}$ is the time-independent matrix from Eq. (8.39). In terms of the discriminant:
$$
\omega_{q}^{2}=\operatorname{det} \Omega_{q}-\frac{\left(\operatorname{tr} \Omega_{q}\right)^{2}}{4}
$$





现代分析入门|Introduction to Modern Analysis代写 MATH 523H

0
Posted on by

这是一份umass麻省大学 MATH 523H作业代写的成功案例

组合数学|Combinatorics代写 MATH 513
问题 1.

Finally, we discuss one useful formula relating the resolvent and spectral projections. It is a matter of computation to see that
$$
f_{z}(x) \rightarrow \begin{cases}0 & x \notin[a, b] \ \frac{1}{2} & x=a \text { or } x=b \ 1 & x \in(a, b)\end{cases}
$$
if $\varepsilon \downarrow 0$ where
$$
f_{z}(x)=\frac{1}{2 \pi i} \int_{a}^{b}\left(\frac{1}{x-\lambda-i \varepsilon}-\frac{1}{x-\lambda+i \varepsilon}\right) d \lambda
$$

证明 .

Moreover, $\left|f_{t}(x)\right|$ is bounded uniformly in $\varepsilon$, so by the functional calculus, one has:

(Stone’s formula) Let $A$ be a bounded self-adjoint operator. Then
$$
\operatorname{s-lim}{\varepsilon \downarrow 0}(2 \pi i)^{-1} \int{a}^{b}\left[(A-\lambda-i \varepsilon)^{-1}-(A-\lambda+i \varepsilon)^{-1}\right] d \lambda=\frac{1}{2}\left[P_{[a, b]}+P_{(a, b)}\right]
$$


英国论文代写Viking Essay为您提供作业代写代考服务

MATH 523H COURSE NOTES ：

Let $A_{1}, \ldots, A_{n}$ be commuting bounded self-adjoint operators on $\mathscr{A}$, a separable Hilbert space.
(a) Let $\Omega_{1}, \ldots, \Omega_{n}$ be Borel sets on $\mathbb{R}$. Prove that $P_{\Omega_{1}}\left(A_{1}\right), P_{\Omega_{2}}\left(A_{2}\right), \ldots, P_{\Omega_{n}}\left(A_{n}\right)$ all commute.
(b) Let $f$ be a function on $\mathbb{R}^{n}$ which is a linear combination of characteristic functions of rectangles (that is, sets of the form $\Omega=\Omega_{1} \times \cdots \times \Omega_{n}$ ). Show that $f$ can be written
$$
f=\sum_{i=1}^{n} c_{i} \chi_{\Omega(t)} \quad \text { with } \Omega^{(t)} \cap \Omega^{(j)}=\varnothing \text { if } i \neq j
$$
(c) For $f$ of the above form, define





组合数学|Combinatorics代写 MATH 513

0
Posted on by

这是一份umass麻省大学 MATH 513作业代写的成功案例

组合数学|Combinatorics代写 MATH 513
问题 1.

$$
T_{n}=n U_{n} \quad \text { implying } \quad U_{n}=n^{n-2} \text {. }
$$
At generating function level, this combinatorial equality translates into
$$
U(z)=\int_{0}^{z} T(w) \frac{d w}{w},
$$

证明 .

which integrates to give (take $T$ as the independent variable)
$$
U(z)=T(z)-\frac{1}{2} T(z)^{2} .
$$
Since $U(z)$ is the EGF of acyclic connected graphs, the quantity
$$
A(z)=e^{U(z)}=e^{T(z)-T(z)^{2} / 2},
$$


英国论文代写Viking Essay为您提供作业代写代考服务

MATH 513 COURSE NOTES ：

Substitution (composition). The composition or substitution can be defined so that it corresponds a priori to composition of generating functions. It is formally defined as
$$
\mathcal{B} \circ \mathcal{C}=\sum_{k=0}^{\infty} \mathcal{B}{k} \times \operatorname{SET}{k}{\mathcal{C}},
$$
so that its EGF is
$$
\sum_{k=0}^{\infty} B_{k} \frac{(C(z))^{k}}{k !}=B(C(z))
$$





纽结理论|Knot Theory代写 MATH 497K

0
Posted on by

这是一份umass麻省大学 MATH 497K作业代写的成功案例

纽结理论|Knot Theory代写 MATH 497K
问题 1.

Deform spinning is another construction that takes $n$-knots to $n+1$ knots. The tersest description of deform spinning comes from once again thinking of a simple spin as a special case of a frame spin, i.e., as
$$
\left[\left(S^{n+1}, S^{n-1}\right)-S^{1} \times\left(B^{n}, B^{n-2}\right)\right] \bigcup_{a}\left[S^{1} \times\left(B_{K}^{n}, B_{K}^{n-2}\right)\right]
$$

证明 .

where $\cup_{a}$ indicates gluing along the common boundary in the obvious (untwisted) fashion. Suppose now that we have a 1-parameter family $f_{\psi}$ of deformations of $B_{K}^{n}$ rel $\partial B_{K}^{n}$ such that $f_{0}$ is the identity and $f_{2} \pi^{n}\left(B_{K}^{n-2}\right)=B_{K}^{n-2}$. The family $f_{\psi}$ should also depend piecewise linearly on the parameter $\psi$. Litherland then describes the deform spin of $K$ as
$$
\left[\left(S^{n+1}, S^{n-1}\right)-S^{1} \times\left(B^{n}, B^{n-2}\right)\right] \bigcup_{a}\left(S^{1} \times B_{K}^{n}, \bigcup_{\psi \in S^{1}} \psi \times f_{\psi}\left(B_{K}^{n-2}\right)\right)
$$


英国论文代写Viking Essay为您提供作业代写代考服务

MATH 497K COURSE NOTES ：

Litherland also shows that, thinking of the collection $f_{\psi}$ as a PL map
$$
f: B_{K}^{n} \times[0,2 \pi] \rightarrow B_{K}^{n} \times[0,2 \pi],
$$
the type of the deform spun knot is dependent only upon the pseudo-isotopy class of $f$ rel $\partial B_{K}^{r}$ as a map of pairs ( $f$ and $g$ are pseudo-isotopic rel $\partial B_{K}^{n}$ as maps of pairs if there is a PL homeomorphism
$$
H:\left(B_{K}^{n}, B_{K}^{n-2}\right) \times[0,2 \pi] \rightarrow\left(B_{K}^{n}, B_{K}^{n-2}\right) \times[0,2 \pi]
$$
such that $\left.H\right|{B{K}^{n} \times 0}$ and $\left.H\right|{a B{K}^{n} \times(0,2 \pi]}$ are the identity maps and $\left.\left.H\right|{B{E}^{n} \times 2 \pi}=f_{2 \pi} g_{2 \pi}^{-1}\right)$.