Category Archives: 物理代写

高级量子物理学|Advanced Quantum Physics PHYSM3416代写

0
Posted on by

Assignment-daixieTM为您提供布里斯托大学University of Bristol Advanced Quantum Physics PHYSM3416高级量子物理学代写代考和辅导服务！

Instructions:

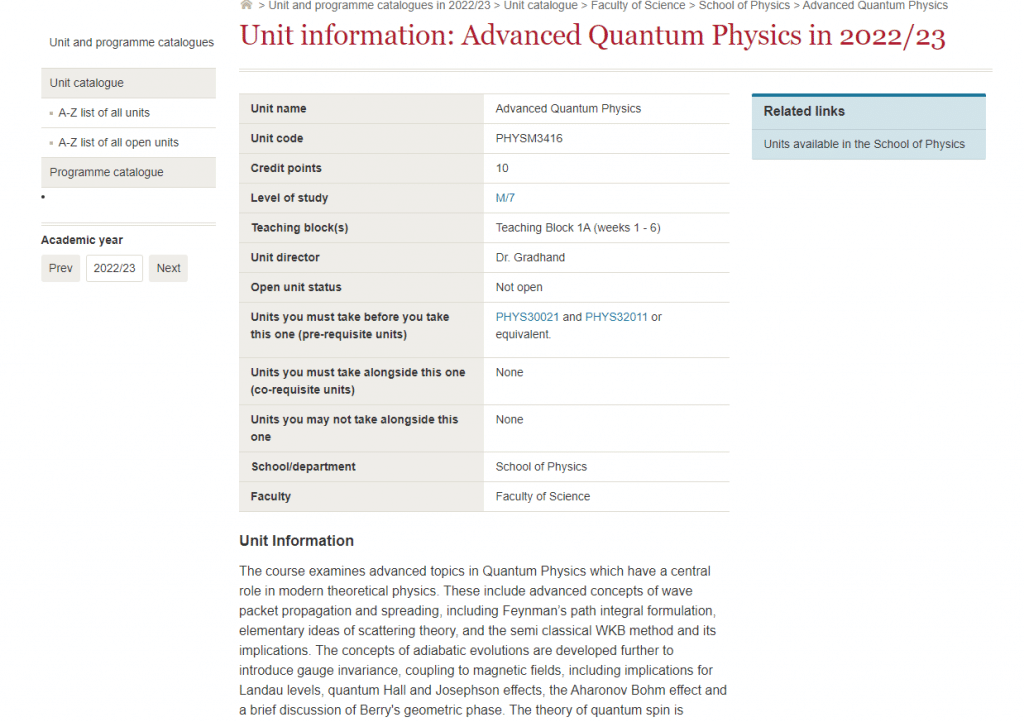
The Feynman path integral formulation is a powerful tool for understanding quantum mechanics, and it allows for the calculation of transition probabilities between states. Scattering theory is important for understanding the interaction of particles and can be used to predict the behavior of atoms, molecules, and nuclei.

The semi-classical WKB method is useful for approximating solutions to quantum mechanical problems, particularly when the potential is slowly varying. Adiabatic evolutions are important for understanding the behavior of systems that change slowly over time, such as quantum computers.

The addition of angular momenta is an important concept in quantum mechanics, and the Clebsch Gordan algebra provides a mathematical framework for understanding it. The Bloch sphere is a useful tool for representing spins, and it has applications in quantum computing and magnetic resonance imaging.

The course also covers magnetic fields and their effects on quantum systems, including Landau levels, the quantum Hall effect, and the Aharonov-Bohm effect. Finally, the discussion of Berry’s geometric phase provides a deeper understanding of the behavior of quantum systems in the presence of external forces.

Overall, this course seems like an excellent opportunity to explore advanced topics in quantum physics and develop a deeper understanding of the behavior of quantum systems.

高级量子物理学|Advanced Quantum Physics PHYSM3416代写

问题 1.

Here we discuss an angular momentum algebra using the one-dimensional harmonic oscillator. Throughout this question we will set $\hbar=1$ (to save ink). Consider a pair of creation and annihilation operators $\hat{a}^{\dagger}$ and $\hat{a}$ satisfying $\left[\hat{a}, \hat{a}^{\dagger}\right]=1$ and a number basis of states $$ |n\rangle=\frac{1}{\sqrt{n !}}\left(\hat{a}^{\dagger}\right)^n|0\rangle, \quad \hat{N}|n\rangle \equiv \hat{a}^{\dagger} \hat{a}|n\rangle=n|n\rangle . $$ (a) Now define, with $j$ a positive integer, the operators $$ J_z=-j+\hat{N}, \quad J_{+}=\hat{a}^{\dagger} \sqrt{2 j-\hat{N}}, \quad J_{-}=J_{+}^{\dagger}=\sqrt{2 j-\hat{N}} \hat{a} . $$ Verify that these operators define an angular momentum algebra. Note: You should not have to expand the square roots in your manipulations. These operators are defined only on states for which the argument of the square root is not negative.

证明 .

(a) To show that the operators $J_z, J_+, J_-$ define an angular momentum algebra, we need to verify that they satisfy the commutation relations \begin{align} [J_z, J_{\pm}] &= \pm J_{\pm}, \ [J_+, J_-] &= 2J_z. \end{align}

First, we have \begin{align} [J_z, J_+] &= [(-j + \hat{N}), (\hat{a}^\dagger \sqrt{2j – \hat{N}})] \ &= \hat{a}^\dagger [(-j + \hat{N}), \sqrt{2j – \hat{N}}] + [(j – \hat{N}), \hat{a}^\dagger] \sqrt{2j – \hat{N}} \ &= \hat{a}^\dagger (-1/2) [\hat{N} – 2j, \sqrt{2j – \hat{N}}] + \hat{a}^\dagger \sqrt{2j – \hat{N}} \ &= \hat{a}^\dagger (-1/2) (\sqrt{2j – \hat{N}} [\hat{N}, \sqrt{2j – \hat{N}}] – [\hat{N}, \sqrt{2j – \hat{N}}]\sqrt{2j – \hat{N}}) + \hat{a}^\dagger \sqrt{2j – \hat{N}} \ &= \hat{a}^\dagger (\sqrt{2j – \hat{N}}) + \hat{a}^\dagger (\sqrt{2j – \hat{N}}) \ &= 2J_+, \end{align} where we have used the commutation relations $[\hat{N}, \hat{a}^\dagger] = \hat{a}^\dagger$ and $[\hat{N}, \hat{a}] = -\hat{a}$.

Next, we have \begin{align} [J_z, J_-] &= [(-j + \hat{N}), (\sqrt{2j – \hat{N}} \hat{a})] \ &= \sqrt{2j – \hat{N}} [(-j + \hat{N}), \hat{a}] + [(-j + \hat{N}), \sqrt{2j – \hat{N}}] \hat{a} \ &= -\sqrt{2j – \hat{N}} \hat{a} + \sqrt{2j – \hat{N}} \hat{a} \ &= 0. \end{align}

Finally, we have \begin{align} [J_+, J_-] &= [(\hat{a}^\dagger \sqrt{2j – \hat{N}}), (\sqrt{2j – \hat{N}} \hat{a})] \ &= \sqrt{2j – \hat{N}} [\hat{a}^\dagger, \hat{a}] \sqrt{2j – \hat{N}} \ &= (2j – \hat{N}) – \hat{N} \ &= 2J_z. \end{align}

Therefore, the operators $J_z, J_+, J_-$ define an angular momentum algebra.

问题 2.

(b) Construct the corresponding $J^2$ operator. Does it satisfy the proper commutation relations with the $J_i$ operators?

证明 .

(b) The $J^2$ operator is defined as $J^2=J_x^2+J_y^2+J_z^2$, where $J_x$, $J_y$, and $J_z$ are the angular momentum operators. In terms of the creation and annihilation operators, we can write these operators as \begin{align} J_x &= \frac{1}{2}\left(\hat{a}^{\dagger}+\hat{a}\right), \ J_y &= \frac{1}{2i}\left(\hat{a}^{\dagger}-\hat{a}\right), \ J_z &= \frac{1}{2}\left(\hat{a}^{\dagger}\hat{a}-\frac{1}{2}\right), \end{align} where we have chosen $\hbar=1$. Then, we can calculate $J^2$ as \begin{align} J^2 &= J_x^2+J_y^2+J_z^2 \ &= \frac{1}{4}\left(\hat{a}^{\dagger}+\hat{a}\right)^2 – \frac{1}{4}\left(\hat{a}^{\dagger}-\hat{a}\right)^2 + \frac{1}{4}\left(\hat{a}^{\dagger}\hat{a}-\frac{1}{2}\right)^2 \ &= \frac{1}{2}\left(\hat{a}^{\dagger}\hat{a}+\frac{1}{4}\right). \end{align} Note that we have used the commutation relation $\left[\hat{a}, \hat{a}^{\dagger}\right]=1$ to simplify the expression. We can check that $J^2$ commutes with $J_x$, $J_y$, and $J_z$: \begin{align} \left[J^2, J_x\right] &= \left[J^2, J_y\right] = \left[J^2, J_z\right] = 0, \end{align} so $J^2$ and $J_i$ form a set of commuting observables.

问题 3.

(c) Find a subset of the harmonic oscillator basis states $|n\rangle$ with $n=0,1,2, \ldots$ that forms a multiplet of $\mathbf{J}$ with angular momentum $j$. Write explicitly your $|j, m\rangle$ states in terms of the oscillator basis states. Confirm that $J_z$ and $J_{+}$act as expected on these states.

证明 .

The angular momentum operators are given by $\mathbf{J}=\mathbf{r} \times \mathbf{p}$, where $\mathbf{r}$ is the position operator and $\mathbf{p}$ is the momentum operator. In one dimension, we have $\mathbf{r}=x$ and $\mathbf{p}=-i \partial / \partial x$. Therefore, the angular momentum operator can be written as

$\mathbf{J}=x p+i \hat{k}$

where $\hat{k}$ is a constant vector in the $z$ direction.

Using the creation and annihilation operators, we can write $x$ and $p$ in terms of $\hat{a}$ and $\hat{a}^{\dagger}$ as

$x=\sqrt{\frac{1}{2 \omega}}\left(\hat{a}+\hat{a}^{\dagger}\right), \quad p=-i \sqrt{\frac{\omega}{2}}\left(\hat{a}-\hat{a}^{\dagger}\right)$,

where $\omega$ is the frequency of the harmonic oscillator.

Substituting these expressions into the expression for $\mathbf{J}$, we get

$\mathbf{J}=\frac{1}{\sqrt{2 \omega}}\left[\left(\hat{a}+\hat{a}^{\dagger}\right)\left(\hat{a}-\hat{a}^{\dagger}\right)+i \hat{k}\right]$.

Let’s define the total number operator as $\hat{N}=\hat{a}^{\dagger} \hat{a}$, which counts the number of excitations in the oscillator. We can use $\hat{N}$ to label the states $|n\rangle$.

Now, we can write down the action of $\mathbf{J}^2$ and $J_z$ on the states $|n\rangle$: \begin{align} \mathbf{J}^2 |n\rangle &= j(j+1) |n\rangle, \ J_z |n\rangle &= m |n\rangle, \end{align} where $j$ and $m$ are the total angular momentum and the $z$ component of angular momentum, respectively. We want to find a subset of the states $|n\rangle$ that form a multiplet of $\mathbf{J}$ with angular momentum $j$.

To do this, we can define the raising and lowering operators

$J_{ \pm}=J_x \pm i J_y=\frac{1}{\sqrt{2}}\left[\left(\hat{a}^{\dagger}\right)^2-\hat{a}^2 \pm i\left(\hat{a}^{\dagger} \hat{a}+\hat{a} \hat{a}^{\dagger}\right)\right]$.

Using the commutation relation $[\hat{a},\hat{a}^\dagger]=1$, we can show that $[J_z,J_{\pm}]=\pm J_{\pm}$ and $[J_+,J_-]=2J_z$. Therefore, if we start with a state $|j,m\rangle$ and apply the raising operator $J_+$ repeatedly, we generate a set of states with angular momentum $j$ that are proportional to $|j,j\rangle$.

这是一份2023年的布里斯托大学University of Bristol University of Bristol Advanced Quantum Physics PHYSM3416高级量子物理学代写的成功案例

核与粒子物理学|Nuclear and Particle Physics PHYS22040代写

0
Posted on by

Assignment-daixieTM为您提供布里斯托大学University of Bristol Foundation Nuclear and Particle Physics PHYS22040核与粒子物理学代写代考和辅导服务！

Instructions:

The quantum world operates according to different laws and principles than the macroscopic world we observe in our daily lives. At the subatomic level, particles can exist in multiple states simultaneously and exhibit behavior that seems counterintuitive. However, the principles of quantum mechanics have been shown to accurately explain and predict the behavior of particles and their interactions.

In studying sub-atomic processes, rates and cross-sections are used to measure the likelihood of certain reactions and interactions occurring. This helps us to better understand the behavior of particles and how they interact with each other.

The semi-empirical mass formula is a tool used in nuclear physics to calculate the binding energy of atomic nuclei. It takes into account the contributions from the strong nuclear force, the electromagnetic force, and the asymmetry energy. The shell model, on the other hand, is a quantum mechanical model that describes how the protons and neutrons in a nucleus are arranged in energy levels or shells.

Using these ideas, we can better understand the stability of atomic nuclei, as well as the processes of nuclear fusion and fission. We can also explore how these processes occur in stars and supernovae, leading to the formation of new elements through nucleosynthesis.

核与粒子物理学|Nuclear and Particle Physics PHYS22040代写

问题 1.

The coupling of the Higgs boson to fermions is proportional to their masses. The partial width is then proportial to the coupling squared. Calculate Higgs boson branching fractions assuming (simplifying) that only decays to bottom and charm quarks as well as to taus and muons are possible.

证明 .

The partial width for Higgs decay to fermion pairs is proportional to the fermion mass squared:
$$
\Gamma_i \propto g_i^2 \propto m_i^2 .
$$
For quarks, there is a color factor of 3. So, for Higgs decay to charms (1.28 GeV), bottoms $(4.18 \mathrm{GeV})$, taus $(1.78 \mathrm{GeV})$ and muons $(0.11 \mathrm{GeV})$, the branching ratios can be calculated by:
$$
\begin{aligned}
& B r[\text { charm }]=\frac{\Gamma_c}{\Gamma_{\text {tot }}}=\frac{3 \times 1.28^2}{3 \times 1.28^2+3 \times 4.18^2+1.78^2+0.11^2}=8.1 \% \
& B r[\text { bottom }]=\frac{\Gamma_b}{\Gamma_{\text {tot }}}=\frac{3 \times 4.18^2}{3 \times 1.28^2+3 \times 4.18^2+1.78^2+0.11^2}=86.6 \% \
& B r[\text { tau }]=\frac{\Gamma_\tau}{\Gamma_{\text {tot }}}=\frac{1.78^2}{3 \times 1.28^2+3 \times 4.18^2+1.78^2+0.11^2}=5.2 \% \
& B r[\text { muon }]=\frac{\Gamma_\mu}{\Gamma_{\text {tot }}}=\frac{0.11^2}{3 \times 1.28^2+3 \times 4.18^2+1.78^2+0.11^2}=0.020 \%
\end{aligned}
$$

问题 2.

Charged pions, $\pi^{+}\left(\pi^{-}\right)$, can decay to electrons as well as to muons and the corresponding neutrinos. In this decay, the parity violation of the weak interaction is maximal. All arguements hold for $\pi^{+}$and $\pi^{-}$.
a)
Prepare a sketch of the pion decay at rest noting the momentum vectors as well as the spin of the involved particles. Using the sketch, discuss why you expect maximal parity violation of the weak interaction in pion decays. Following this discussion, which branching fraction is larger? Include your reasoning in the answer.

证明 .

  • a)
    The decay, here for $\pi^{-}$, follows:
    $$
    \pi^{-} \rightarrow \ell^{-} \bar{\nu}_{\ell}, \quad \ell=\mu, \mathrm{e}
    $$
    The $\mathrm{W}^{-}$boson couples exclusively to left-handed particles and right-handed antiparticles. Since we can assume the neutrino to be massless, its chirality corresponds to its helicity. The momentum- and spin-configuration is shown in Fig 1.

问题 3.

b)
Show the following relation for momentum and energy, assuming that neutrinos are massless:
$$
\begin{aligned}
& p_{\ell}=\frac{m_\pi^2-m_{\ell}^2}{2 m_\pi} \
& E_{\ell}=\frac{m_\pi^2+m_{\ell}^2}{2 m_\pi},
\end{aligned}
$$
with $m_\pi=139,57 \mathrm{MeV}$ the mass of the pion and $m_{\ell}=105,66(0.511) \mathrm{MeV}$ the mass of the muon (electron).

证明 .

b)
Using energy conservation, we find for pion at rest:
$$
E_\pi=m_\pi=E_\nu+E_{\ell}, \quad \text { mit: } p_\nu=p_{\ell}=p
$$
For the momentum we find:

$$
\begin{aligned}
& E_\pi=m_\pi=E_\nu+E_{\ell}, \quad \text { mit: } p_\nu=p_{\ell}=p \
& E_\pi=m_\pi=p+\sqrt{p^2+m_{\ell}^2} \
& \left(m_\pi-p\right)^2=p^2+m_{\ell}^2 \
& m_\pi^2-2 m_\pi p+p^2=p^2+m_{\ell}^2 \
& p=\frac{m_\pi^2-m_{\ell}^2}{2 m_\pi}
\end{aligned}
$$
And for the energy:
$$
\begin{aligned}
E & =\sqrt{p^2+m_{\ell}^2} \
& =\frac{1}{2 m_\pi} \sqrt{m_\pi^2-2 m_\pi^2 m_{\ell}^2+m_{\ell}^4+4 m_\pi^2 m_{\ell}^2} \
& =\frac{1}{2 m_\pi} \sqrt{\left(m_\pi^2+m_{\ell}^2\right)^2} \
E & =\frac{m_\pi^2+m_{\ell}^2}{2 m_\pi}
\end{aligned}
$$

这是一份2023年的布里斯托大学University of Bristol University of Bristol Nuclear and Particle Physics PHYS22040核与粒子物理学代写的成功案例

电磁学和电波|Classical Physics II: Electromagnetism and Waves  PHYS20020代写

0
Posted on by

Assignment-daixieTM为您提供布里斯托大学University of Bristol Foundation Classical Physics II: Electromagnetism and Waves  PHYS20020电磁学和电波代写代考和辅导服务！

Instructions:

Classical Physics is a branch of physics that deals with the study of macroscopic phenomena at speeds much slower than the speed of light. It was developed in the 17th to 19th centuries and forms the foundation of modern physics. The core concepts of classical physics include mechanics, thermodynamics, electromagnetism, and optics.

This unit builds on the foundations developed in level C/4 in the areas of electromagnetic fields and waves. Maxwell’s equations, which describe the behavior of electric and magnetic fields, are studied in vacuo and in simple solids. These equations form the basis of a discussion of fields, forces, and energy for general charge and current configurations.

The wave solutions of Maxwell’s equations are also studied in this unit. These solutions relate the electromagnetic and optical properties of materials, providing a deeper understanding of how light interacts with matter. General wave phenomena, such as interference and diffraction, are investigated, along with practical applications of these effects.

Overall, this unit provides a comprehensive understanding of electromagnetic fields and waves, which is essential for understanding many aspects of modern physics and technology.

电磁学和电波|Classical Physics II: Electromagnetism and Waves  PHYS20020代写

问题 1.

Use index notation to derive a formula for $\vec{\nabla} \times(s \vec{A})$, where $s$ is a scalar field $s(\vec{r})$ and $\vec{A}$ is a vector field $\vec{A}(\vec{r})$.

证明 .

$\begin{aligned} {[\vec{\nabla} \times(s \vec{A})]i } & =\varepsilon{i j k} \partial_j(s \vec{A})k \ & =\varepsilon{i j k} s \partial_j A_k+\varepsilon_{i j k} A_k \partial_j s \ & =s \vec{\nabla} \times \vec{A}+\vec{\nabla} s \times \vec{A} .\end{aligned}$

问题 2.

Which of the following vector fields could describe an electric field? Say yes or no for each, and give a very brief reason.
(i) $\vec{E}(\vec{r})=x \hat{e}_x-y \hat{e}_y$.
(ii) $\vec{E}(\vec{r})=y \hat{e}_x+x \hat{e}_y$.
(iii) $\vec{E}(\vec{r})=y \hat{e}_x-x \hat{e}_y$.

证明 .

The curl of an electrostatic field must be zero, but otherwise there is no restriction. So the answer follows as
(i) $\vec{\nabla} \times \vec{E}(\vec{r})=\left(\frac{\partial E_y}{\partial x}-\frac{\partial E_x}{\partial y}\right) \hat{e}_z+\ldots=\overrightarrow{0}$. YES, it describes an electric field.
(ii) $\vec{\nabla} \times \vec{E}(\vec{r})=(1-1) \hat{e}_z=0$. YES, it describes an electric field.
(iii) $\vec{\nabla} \times \vec{E}(\vec{r})=(-1-1) \hat{e}_z=-2 \hat{e}_z$. NO, it does not describe an electric field.

问题 3.

(a) A spherical shell of radius $R$, with an unspecified surface charge density, is centered at the origin of our coordinate system. The electric potential on the shell is known to be
$$
V(\theta, \phi)=V_0 \sin \theta \cos \phi,
$$
where $V_0$ is a constant, and we use the usual polar coordinates, related to the Cartesian coordinates by
$$
\begin{aligned}
& x=r \sin \theta \cos \phi, \
& y=r \sin \theta \sin \phi, \
& z=r \cos \theta .
\end{aligned}
$$
Find $V(r, \theta, \phi)$ everywhere, both inside and outside the sphere. Assume that the zero of $V$ is fixed by requiring $V$ to approach zero at spatial infinity. (Hint: this problem can be solved using traceless symmetric tensors, or if you prefer you can use standard spherical harmonics. A table of the low- $\ell$ Legendre polynomials and spherical harmonics is included with the formula sheets.)

证明 .

This problem can be solving using either traceless symmetric tensors or the more standard spherical harmonics. I will show the solution both ways, starting with the simplier derivation in terms of traceless symmetric tensors.
(a) We exploit the fact that the most general solution to Laplace’s equation can be written as a sum of terms of the form
$$
\left(r^{\ell} \text { or } \frac{1}{r^{\ell+1}}\right) C_{i_1 \ldots i_{\ell}}^{(\ell)} \hat{n}{i_1} \ldots \hat{n}{i_{\ell}},
$$
where $C_{i_1 \ldots i_{\ell}}^{(\ell)}$ is a traceless symmetric tensor. In this case we only need an $\ell=1$ term, since
$$
F_a(\theta, \phi) \equiv \sin \theta \cos \phi=\frac{x}{r}=\hat{x}_i \hat{n}_i
$$
For $\ell=1$ the radial function must be $r$ or $1 / r^2$. For $rR$ the term proportional to $r$ is excluded, because it does not approach zero as $r \rightarrow \infty$, so only the $1 / r^2$ option remains, and the solution is
$$
\begin{aligned}
V(\vec{r}) & =V_0\left(\frac{R}{r}\right)^2 F_a(\theta, \phi) \
& =V_0\left(\frac{R}{r}\right)^2 \hat{x}_i \hat{n}_i \text { or } V_0\left(\frac{R}{r}\right)^2 \sin \theta \cos \phi .
\end{aligned}
$$

这是一份2023年的布里斯托大学University of Bristol University of BristolClassical Physics II: Electromagnetism and Waves  PHYS20020电磁学和电波代写的成功案例

物理学基础代写|Foundation Physics PHYS10010代写

0
Posted on by

Assignment-daixieTM为您提供布里斯托大学University of Bristol Foundation Physics PHYS10010物理学基础代写代考和辅导服务！

Instructions:

You will be introduced to the fundamental theories and principles of physics, including classical mechanics, electromagnetism, and thermodynamics. You will also learn how to apply your understanding of these concepts to solve simple problems and conduct laboratory experiments to reinforce your knowledge.

Throughout the unit, you will develop your analytical and problem-solving skills, which are essential for success in any undergraduate physics program. You will also gain practical experience in a laboratory setting, where you will learn to use various tools and equipment to conduct experiments, collect and analyze data, and draw conclusions.

By the end of the unit, you should feel confident in your understanding of fundamental physics concepts and be prepared for the challenges of an undergraduate degree program in physics or a related field.

物理学基础代写|Foundation Physics PHYS10010代写

问题 1.

(a) Let $\psi(x)$ be an energy eigenstate. Explain why the expectation value $\langle[H, \Omega]\rangle$ of the commutator of $H$ with an arbitrary operator $\Omega$ vanishes on the state $\psi$.

证明 .

Let $\psi(x)$ be an energy eigenstate of the Hamiltonian $H$. Then, we have \begin{align} \langle [H,\Omega]\rangle &= \langle H\Omega – \Omega H \rangle \ &= \langle H\Omega \rangle – \langle \Omega H \rangle \ &= E_\psi \langle \Omega \rangle – \langle \Omega H \rangle \ &= E_\psi \langle \Omega \rangle – E_\psi \langle \Omega \rangle \ &= 0, \end{align} where we have used the fact that $\psi$ is an energy eigenstate with eigenvalue $E_\psi$, and that the expectation value of $H$ on $\psi$ is just $E_\psi$. Therefore, the commutator of $H$ with an arbitrary operator $\Omega$ vanishes on the state $\psi$.

问题 2.

(b) Choose $\Omega=x p$, and take $$ H=\frac{p^2}{2 m}+V(x) $$ Use the claim from part (a) to find a relation between the expectation value $\langle T\rangle$ of the kinetic energy and the expectation value of a combination of $x$ and the derivative $V^{\prime}(x)$ of the potential with respect to its argument. Both expectation values are taken on an energy eigenstate.

证明 .

From part (a), we have shown that for any operator $A$ and Hamiltonian $H$,

$\langle[A, H]\rangle=i \hbar\langle\partial A / \partial t\rangle$.

We can use this to find a relation between the expectation value of the kinetic energy and the expectation value of a combination of $x$ and $V^{\prime}(x)$.

Let’s first find the commutator $[x,H]$ and $[p,H]$: \begin{align*} [x,H] &= xp – px = i\hbar, \ [p,H] &= \frac{p^3}{2m} + pV(x) – V(x)p – \frac{p}{2m}p^2 \ &= \frac{p}{m}(V(x)-p^2/2m) + [p,V(x)] \ &= -\frac{\partial V(x)}{\partial x}. \end{align*} Therefore, we have \begin{align*} \langle T \rangle &= \langle \frac{p^2}{2m} \rangle \ &= \frac{1}{2m} \langle p^2 \rangle \ &= \frac{1}{2m} \langle [p,p^2] \rangle \ &= \frac{1}{2m} \langle p[p,p] + [p,p]p \rangle \ &= \frac{1}{2m} \langle -p\hbar + \hbar p \rangle \ &= \frac{\hbar^2}{2m} \langle -p+p \rangle \ &= \frac{\hbar^2}{2m} \langle [x,H] \rangle \ &= \frac{i\hbar^3}{2m} \langle \partial x/\partial t \rangle \ &= \frac{i\hbar^3}{2m} \frac{\partial}{\partial t} \langle x \rangle \ &= \frac{i\hbar^3}{2m} \frac{\partial}{\partial t} \left(\frac{\langle [x,H] \rangle}{i\hbar}\right) \ &= -\frac{\hbar^2}{2m} \frac{\partial}{\partial t} \langle \frac{\partial V(x)}{\partial x} \rangle \ &= -\frac{\hbar^2}{2m} \frac{\partial}{\partial t} \langle V^{\prime}(x) \rangle. \end{align*} Thus, we have shown that the expectation value of the kinetic energy is related to the time derivative of the expectation value of $V^{\prime}(x)$ on an energy eigenstate.

问题 3.

Throughout this problem we consider a hydrogen atom with fixed principal quantum number $n$, with $\ell=n-1$, and $m=n-1$. The value $n$ is arbitrary and possibly large.
(a) Write the wavefunction $\psi_{n, \ell, m}(r, \theta, \phi)$ in terms of the relevant spherical harmonic and a radial factor fully determined except for an overall unit-free normalization constant $N$.

证明 .

The wavefunction for a hydrogen atom with principal quantum number $n$, $\ell=n-1$, and $m=n-1$ can be expressed as:

$\psi_{n, \ell, m}(r, \theta, \phi)=R_{n, \ell}(r) Y_{\ell}^m(\theta, \phi)$

where $R_{n,\ell}(r)$ is the radial part of the wavefunction and $Y_{\ell}^{m}(\theta,\phi)$ is the spherical harmonic. Since $\ell=n-1$ and $m=n-1$, we have $\ell=m=n-1$. Therefore, the spherical harmonic reduces to:

$Y_{n-1}^{n-1}(\theta, \phi)=(-1)^{n-1} \sqrt{\frac{(2 n-1) ! !}{4 \pi(n-1) !}} P_{n-1}^{n-1}(\cos \theta) e^{i(n-1) \phi}$

where $P_{n-1}^{n-1}(\cos\theta)$ is the associated Legendre polynomial of degree $n-1$. The radial part of the wavefunction $R_{n,\ell}(r)$ can be obtained from the radial Schrödinger equation for hydrogen atom:

$\frac{d^2 R_{n, \ell}(r)}{d r^2}+\frac{2}{r} \frac{d R_{n, \ell}(r)}{d r}-\frac{\ell(\ell+1)}{r^2} R_{n, \ell}(r)+\frac{-\frac{1}{2}\left(\frac{1}{n}\right)^2}{-\frac{1}{2}} R_{n, \ell}(r)=0$

Simplifying the equation, we get:

$\frac{d^2 R_{n, \ell}(r)}{d r^2}+\frac{2}{r} \frac{d R_{n, \ell}(r)}{d r}-\frac{\ell(\ell+1)}{r^2} R_{n, \ell}(r)+\frac{1}{n^2} R_{n, \ell}(r)=0$

This equation has the standard form of a differential equation for the radial part of a spherically symmetric potential. The solution can be written in terms of the associated Laguerre polynomial $L_{n-\ell-1}^{2\ell+1}(2r/n)$ as:

$R_{n, \ell}(r)=\sqrt{\frac{(n-\ell-1) !}{2 n[(n+\ell) ! 3}}\left(\frac{2 r}{n}\right)^{\ell} e^{-r / n} L_{n-\ell-1}^{2 \ell+1}\left(\frac{2 r}{n}\right)$

Therefore, the wavefunction for a hydrogen atom with principal quantum number $n$, $\ell=n-1$, and $m=n-1$ is:

$\psi_{n, n-1, n-1}(r, \theta, \phi)=N \sqrt{\frac{(2 n-1) ! !}{4 \pi(n-1) !}}\left(\frac{2 r}{n}\right)^{n-1} e^{-r / n} L_1^{2 n-1}\left(\frac{2 r}{n}\right) e^{i(n-1) \phi}$

where $N$ is an overall unit-free normalization constant.

这是一份2023年的布里斯托大学University of Bristol University of Bristol Foundation Physics PHYS10010物理学基础代写的成功案例

物理学的基本数学代写|Essential Maths for Physics PHYS11400代写

0
Posted on by

Assignment-daixieTM为您提供布里斯托大学University of Bristol Essential Maths for Physics PHYS11400物理学的基本数学代写代考和辅导服务！

Instructions:

Mathematics plays a crucial role in understanding and describing physical phenomena. Here are some essential math topics for physics:

  1. Calculus: Calculus is the branch of mathematics that deals with the study of continuous change. It is essential for physics because it helps in understanding how things change over time. Calculus concepts like differentiation and integration are widely used in physics, for instance, in the description of motion and the calculation of rates of change.
  2. Linear Algebra: Linear algebra deals with the study of linear equations, matrices, and vector spaces. It is used to solve systems of equations, which frequently arise in physics problems. Linear algebra is also used to describe transformations and rotations, which are essential in understanding the behavior of objects in motion.
  3. Differential Equations: Differential equations are equations that involve derivatives or rates of change. They are widely used in physics to describe the behavior of systems that change over time. Examples include the motion of particles under the influence of forces, the behavior of circuits, and the decay of radioactive materials.
  4. Complex Analysis: Complex analysis deals with the study of complex numbers and their properties. It is used to analyze functions that are defined in the complex plane, such as those that describe the behavior of electromagnetic waves. Complex analysis is also essential for understanding quantum mechanics.
  5. Statistics and Probability: Statistics and probability are essential for making predictions and inferences in physics. They are used to analyze data and to make quantitative predictions about the behavior of physical systems. Examples include the probability of a particle being found in a certain location and the probability of a system undergoing a particular change.
  6. Fourier Analysis: Fourier analysis is used to describe periodic functions and their properties. It is used extensively in physics to analyze signals and to understand the behavior of waves, including sound waves, electromagnetic waves, and quantum waves.
  7. Group Theory: Group theory is the study of symmetry and structure. It is used in physics to describe the behavior of particles, including their interactions with each other. Group theory is also essential for understanding the behavior of crystals, which have a regular, repeating structure.

These are just some of the essential math topics for physics. A strong foundation in these areas is crucial for understanding the mathematical underpinnings of physical phenomena.

物理学的基本数学代写|Essential Maths for Physics PHYS11400代写

问题 1.

Reduce the following ordinary differential equation to a first-order vector differential equations, which you should write out completely, in vector format. $$ \left(\frac{d^3 y}{d x^3}\right)^3–\frac{d^2 y}{d x^2}-y^2=0 $$

证明 .

Let $y_1=y$, $y_2=\frac{d y}{d x}$, and $y_3=\frac{d^2 y}{d x^2}$. Then, we can write the given third-order differential equation as a system of three first-order differential equations:

\begin{align*} \frac{d y_1}{d x} &= y_2 \ \frac{d y_2}{d x} &= y_3 \ \frac{d y_3}{d x} &= \sqrt[3]{y_3^3+y_2+y_1^2} \end{align*}

Thus, we have reduced the given third-order differential equation to a first-order vector differential equation in vector format:

$\frac{d}{d x}\left(\begin{array}{l}y_1 \ y_2 \ y_3\end{array}\right)=\left(\begin{array}{c}y_2 \ y_3 \ \sqrt[3]{y_3^3+y_2+y_1^2}\end{array}\right)$

问题 2.

Divergence of acceleration in phase space. (a) Prove that particles of charge $q$ moving in a magnetic field $\mathbf{B}$ and hence subject to a force $q \mathbf{v} \times \mathbf{B}$, nevertheless have $\nabla_v \cdot \mathbf{a}=0$.

证明 .

(a) The acceleration $\mathbf{a}$ of a charged particle moving in a magnetic field is given by $\mathbf{a} = (q/\gamma)m \mathbf{v} \times \mathbf{B}$, where $\gamma$ is the relativistic factor and $m$ is the mass of the particle. Using the vector identity $\nabla \cdot (\mathbf{a} \times \mathbf{b}) = \mathbf{b} \cdot (\nabla \times \mathbf{a}) – \mathbf{a} \cdot (\nabla \times \mathbf{b})$, we can write:

\begin{align} \nabla_v \cdot \mathbf{a} &= \frac{\partial}{\partial v_x} \left(\frac{q}{\gamma} m B_y v_z\right) + \frac{\partial}{\partial v_y} \left(\frac{q}{\gamma} m B_z v_x\right) + \frac{\partial}{\partial v_z} \left(\frac{q}{\gamma} m B_x v_y\right) \ &= \frac{q}{\gamma} m B_y \frac{\partial}{\partial v_x}(v_z) + \frac{q}{\gamma} m B_z \frac{\partial}{\partial v_y}(v_x) + \frac{q}{\gamma} m B_x \frac{\partial}{\partial v_z}(v_y) \ &= 0, \end{align}

where we have used the fact that $\partial v_i/\partial v_j = \delta_{ij}$, the Kronecker delta.

Therefore, the divergence of acceleration for a charged particle in a magnetic field is zero.

问题 3.

(b) Consider a frictional force that slows particles down in accordance with $\mathbf{a}=-K \mathbf{v}$, where $K$ is a constant. What is the “velocity-divergence”, of this acceleration, $\nabla_v \cdot \mathbf{a}$ ? Does this cause the distribution function $f$ to increase or decrease as a function of time?

证明 .

For a frictional force that slows particles down according to $\mathbf{a} = -K \mathbf{v}$, the velocity-divergence is given by:

\begin{align} \nabla_v \cdot \mathbf{a} &= \frac{\partial}{\partial v_x}(-Kv_x) + \frac{\partial}{\partial v_y}(-Kv_y) + \frac{\partial}{\partial v_z}(-Kv_z) \ &= -3K. \end{align}

The velocity-divergence is negative, indicating that the distribution function $f$ will decrease as a function of time. This is because the frictional force removes kinetic energy from the particles, causing them to slow down and reduce their probability of occupying higher velocity states.

这是一份2023年的布里斯托大学University of Bristol University of Bristol Essential Maths for Physics PHYS11400物理学的基本数学代写的成功案例

振荡,波和场代写|Oscillations, Waves and Fields PHYS10005代写

0
Posted on by

Assignment-daixieTM为您提供布里斯托大学University of Bristol Oscillations, Waves and Fields PHYS10005振荡,波和场代写代考和辅导服务！

Instructions:

Physics that builds on the foundation of A level Physics A2 and Core Physics I (Mechanics and Matter). In this unit, you will learn about waves and oscillations, which are fundamental concepts in physics that can be applied to a variety of fields, such as acoustics, optics, and quantum mechanics. You will also be introduced to the concept of the wave function, which is a fundamental concept in quantum mechanics that describes the behavior of quantum particles.

In addition to waves and oscillations, the unit will cover the concept of fields and their mathematical treatment in terms of vectors and vector operators in three dimensions. Fields are an important concept in physics because they help us understand how forces are transmitted through space. By learning about fields, you will gain a deeper understanding of how the physical world works.

Finally, the unit will cover fundamental particles and fields. This includes topics such as the Standard Model of particle physics, which is a theoretical framework that describes the fundamental particles and forces in the universe. By learning about fundamental particles and fields, you will gain a deeper understanding of the building blocks of the universe and the forces that govern their behavior.

振荡,波和场代写|Oscillations, Waves and Fields PHYS10005代写

问题 1.

A vertical spring on which is hung a block of mass $m_1$ oscillates with angular frequency $\omega$. With an additional block of mass $m_2 \neq m_1$ added to the spring, the frequency is $\omega / 2$. What is the ratio $m_1 / m_2$ ?

证明 .

We know the general formula for the angular frequency of a mass-spring system of spring constant $k$ and total mass $M_{\text {tot }}$ is
$$
\omega=\sqrt{\frac{k}{M_{\text {tot }}}} .
$$
If we have $\omega_{\text {before }}=\sqrt{k / m_1}$ and $\omega_{\text {after }}=\sqrt{k /\left(m_1+m_2\right)}$ and
$$
\omega_{\text {after }}=\frac{1}{2} \omega_{\text {before }},
$$
then we have
$$
\sqrt{\frac{k}{m_1+m_2}}=\frac{1}{2} \sqrt{\frac{k}{m_1}},
$$
or $m_1+m_2=4 m_1$ which gives us $m_1 / m_2=1 / 3$

问题 2.

You stop your car to pick up a member of your car pool. After she gets in, does the angular frequency $\omega$ of the oscillation due to the car’s suspension, increase, decrease or stay the same?

证明 .

We can effectively take the suspension of the car to be a spring of some spring constant $k$. If $M_{\text {tot }}$ is the mass of the car then the angular frequency of the suspension is
$$
\omega_0=\sqrt{\frac{k}{M_{\mathrm{tot}}}} .
$$

If we add $m$ to the $M_{\text {total }}$ we find a new angular frequency
$$
\omega_f=\sqrt{\frac{k}{M_{\mathrm{tot}}+m}}<\sqrt{\frac{k}{M_{\mathrm{tot}}}}=\omega_0 .
$$
Therefore, the angular frequency due to the car’s suspension decreases.

问题 3.

Given an object suspended by a spring, which of these variables of the motion can you control by varying the initial conditions: period, amplitude, energy of the system, frequency, phase, maximum velocity, maximum acceleration

证明 .

For a mass-spring system, the angular frequency is $\omega=\sqrt{k / m}$ and the period is $T=2 \pi / \omega$. Both of these quantities are fixed by the setup of the system and are therefore independent of the initial conditions. Everything else in the system is determined by the initial conditions. In particular, the motion is given by
$$
x(t)=A_0 \cos (\omega t-\phi),
$$
where $A_0=\sqrt{x_0^2+v_0^2 / \omega^2}$ and $\tan \phi=v_0 / \omega x_0$. The total energy of the system is $E=\frac{1}{2} k A_0^2$. The maximum velocity and acceleration are, respectively, $A_0 \omega$ and $A_0 \omega^2$. Thus we see that the energy of the system, the phase, the maximum velocity, and the maximum acceleration are controlled by the initial conditions.

这是一份2023年的布里斯托大学University of Bristol Oscillations, Waves and Fields PHYS10005代写的成功案例

力学和质代写|Mechanics and Matter PHYS10006 University of Bristol Assignment

0
Posted on by

Assignment-daixieTM为您提供布里斯托大学University of Bristol Mechanics and Matter PHYS10006力学和质代写代考和辅导服务！

Instructions:

Mechanics is a branch of physics that studies the motion of objects and the forces that cause them. It is concerned with how objects move and interact with each other, and how to describe and predict these behaviors using mathematical models. Mechanics is one of the oldest and most fundamental branches of physics, and has many important applications in engineering, technology, and everyday life.

Matter is anything that has mass and takes up space. It includes all the substances that we can see and touch, as well as those that are invisible to us, such as gases and subatomic particles. Matter is made up of tiny particles called atoms, which are themselves composed of even smaller particles such as protons, neutrons, and electrons.

Mechanics and matter are closely related, since mechanics is concerned with the motion and behavior of objects, and all objects are made of matter. The behavior of matter can be described and predicted using the laws of mechanics, which tell us how objects move and interact with each other under various conditions. For example, mechanics can be used to describe the motion of a ball rolling down a hill, the behavior of a spring as it is compressed and released, or the movement of planets and stars in the universe.

In summary, mechanics and matter are two important concepts in physics that are closely related. Mechanics helps us understand how objects move and interact with each other, while matter is the substance that makes up these objects and is affected by these interactions. Together, these concepts help us to understand the behavior of the physical world around us.

力学和质代写|Mechanics and Matter PHYS10006 University of Bristol Assignment

问题 1.

In a crash test, a truck with mass $2500 \mathrm{~kg}$ traveling at $24 \mathrm{~m} / \mathrm{s}$ smashes head-on into a concrete wall without rebounding. The front end crumples so much that the truck is $0.72 \mathrm{~m}$ shorter than before. (b) About how long does the collision last? (That is, how long is the interval between first contact with the wall and coming to a stop?)

证明 .

We can use the conservation of energy to find the initial kinetic energy of the truck:

$\frac{1}{2} m v^2=\frac{1}{2}(2500 \mathrm{~kg})(24 \mathrm{~m} / \mathrm{s})^2=1.44 \times 10^6 \mathrm{~J}$

During the collision, this kinetic energy is converted into deformation work done on the truck, which we can calculate from the change in length:

$W=\frac{1}{2} k x^2=\frac{1}{2} \frac{F}{\Delta x} x^2$

where $k$ is the spring constant of the deformed truck, $x$ is the amount of deformation, and $F$ is the average force exerted by the wall on the truck. Since the truck doesn’t rebound, we can assume that all of the initial kinetic energy is converted into deformation work, so we can equate these two expressions and solve for $F$:

$1.44 \times 10^6 \mathrm{~J}=\frac{1}{2} \frac{F}{0.72 \mathrm{~m}}(0.72 \mathrm{~m})^2 \Rightarrow F=2.96 \times 10^6 \mathrm{~N}$

问题 2.

(c) What is the magnitude of the average force exerted by the wall on the truck during the collision?

证明 .

We can use the impulse-momentum theorem to find the time interval $\Delta t$ during which the force is exerted:

$F \Delta t=\Delta p=m v_f-m v_i=2500 \mathrm{~kg}(0 \mathrm{~m} / \mathrm{s}-24 \mathrm{~m} / \mathrm{s})=-6.0 \times 10^4 \mathrm{~kg} \mathrm{~m} / \mathrm{s}$

Since the truck comes to a stop, the change in momentum is negative. Solving for $\Delta t$ gives:

$\Delta t=\frac{-6.0 \times 10^4 \mathrm{~kg} \mathrm{~m} / \mathrm{s}}{2.96 \times 10^6 \mathrm{~N}}=0.020 \mathrm{~s}$

So the collision lasts about 0.020 seconds.

问题 3.

It is interesting to compare this force to the weight of the truck. Calculate the ratio of the force of the wall to the gravitational force $m g$ on the truck. This large ratio shows why a collision is so damaging.

证明 .

The weight of the truck is $mg = (2500 \mathrm{~kg})(9.81 \mathrm{~m/s^2}) = 24.5 \times 10^3 \mathrm{~N}$. The ratio of the force of the wall to the gravitational force on the truck is:

$\frac{2.96 \times 10^6 \mathrm{~N}}{24.5 \times 10^3 \mathrm{~N}}=120$

So the force exerted by the wall is 120 times greater than the weight of the truck. This large ratio shows why a collision can be so damaging.

这是一份2023年的布里斯托大学University of Bristol Mechanics and Matter PHYS10006代写的成功案例

牛顿力学代写|NEWTONIAN MECHANICS MATH122 University of Liverpool Assignment

0
Posted on by

Assignment-daixieTM为您提供利物浦大学University of Liverpool NEWTONIAN MECHANICS MATH122牛顿力学代写代考和辅导服务！

Instructions:

Newtonian mechanics, also known as classical mechanics, is a branch of physics that deals with the study of motion and its causes. It was founded by Sir Isaac Newton in the 17th century and is based on three laws known as Newton’s laws of motion.

Newton’s first law of motion states that an object at rest will remain at rest, and an object in motion will continue in motion with a constant velocity unless acted upon by an external force. This law is also known as the law of inertia.

Newton’s second law of motion states that the force acting on an object is equal to the mass of the object times its acceleration. Mathematically, F=ma, where F is the force, m is the mass, and a is the acceleration.

Newton’s third law of motion states that for every action, there is an equal and opposite reaction. This law implies that forces always occur in pairs.

In addition to these laws, Newtonian mechanics also includes the concepts of energy, work, and momentum. The laws of conservation of energy, work-energy theorem, and conservation of momentum are also important principles in this branch of physics.

Newtonian mechanics has been instrumental in the development of modern physics, and its principles are still widely used in many fields of science and engineering.

牛顿力学代写|NEWTONIAN MECHANICS MATH122 University of Liverpool Assignment

问题 1.

A rocket in zero gravitational field has a mass of $m_{r, i}=2.81 \times 10^7 \mathrm{~kg}$, which is the sum of the mass of the fuel $m_{f, i}=2.46 \times 10^7 \mathrm{~kg}$ and the dry mass of the rocket (empty of fuel) $m_{r, d} \equiv m_{r, i}-m_{f, i}=0.35 \times 10^7 \mathrm{~kg}$. The fuel is ejected at a speed $u=3000$ $\mathrm{m} / \mathrm{s}$ relative to the rocket. The total burn time is $510 \mathrm{~s}$ and the fuel is burned at a constant rate. (a) What is the final speed $v_f$ of the rocket in meters/second after all the fuel is burned assuming it starts from rest?

证明 .

The total mass of the rocket decreases during the burn due to the ejection of fuel. The change in velocity of the rocket is given by the rocket equation:

$\Delta v=v_{f}-v_{i}=u \ln \left(\frac{m_{r, i}}{m_{r, f}}\right)$

where $v_i = 0$ is the initial velocity of the rocket, $v_f$ is the final velocity of the rocket, $m_{r,i}$ is the initial mass of the rocket, $m_{r,f}$ is the final mass of the rocket, and $u$ is the relative velocity of the ejected fuel. At the end of the burn, all the fuel has been ejected, so the final mass of the rocket is the dry mass $m_{r,d}$:

$m_{r, f}=m_{r, d}=0.35 \times 10^7 \mathrm{~kg}$

Thus,

$\Delta v=u \ln \left(\frac{m_{r, i}}{m_{r, d}}\right)=3000 \mathrm{~m} / \mathrm{s} \ln \left(\frac{2.81 \times 10^7 \mathrm{~kg}}{0.35 \times 10^7 \mathrm{~kg}}\right) \approx 7974.4 \mathrm{~m} / \mathrm{s}$

Therefore, the final speed of the rocket is $v_f = \Delta v = 7974.4$ m/s.

问题 2.

(b) Now suppose that the same rocket burns the fuel in two stages, expelling the fuel in each stage at the same relative speed $u=3000 \mathrm{~m} / \mathrm{s}$. In stage one, the available fuel to burn is $m_{f, 1, i}=2.03 \times 10^7 \mathrm{~kg}$ with burn time $150 \mathrm{~s}$. The total mass of the rocket after all the fuel in stage 1 is burned is $m_{r, 1, d}=m_{r, i}-m_{f, 1, i}=0.78 \times 10^7$ kg. What is the change in speed after stage one is complete?

证明 .

In stage one, the initial mass of the rocket is $m_{r,1,i}=m_{r,i}=2.81 \times 10^7 \mathrm{~kg}$ and the initial mass of fuel is $m_{f,1,i}=2.03 \times 10^7 \mathrm{~kg}$. The mass of the rocket after all the fuel in stage 1 is burned is $m_{r,1,f}=m_{r,1,i}-m_{f,1,i}=0.78 \times 10^7$ kg. By the principle of conservation of momentum, the change in velocity of the rocket after stage one is complete is given by:

$\Delta v_1=u\ln \left(\frac{m_{r,1,i}}{m_{r,1,f}}\right)$

where $u=3000 \mathrm{~m}/\mathrm{s}$ is the relative speed of the ejected fuel and $m_{r,1,i}$ and $m_{r,1,f}$ are the initial and final masses of the rocket in stage one, respectively. Substituting the values:

$\Delta v_1=3000 \mathrm{~m}/\mathrm{s} \ln \left(\frac{2.81 \times 10^7 \mathrm{~kg}}{0.78 \times 10^7 \mathrm{~kg}}\right) \approx 3066 \mathrm{~m}/\mathrm{s}$

Therefore, the change in speed after stage one is complete is approximately $3066 \mathrm{~m}/\mathrm{s}$.

问题 3.

(c) Next, the empty fuel tank and accessories from stage one are disconnected from the rest of the rocket. These disconnected parts had a mass $m=1.4 \times 10^6 \mathrm{~kg}$, hence the remaining dry mass of the rocket is $m_{r, 2, d}=2.1 \times 10^6 \mathrm{~kg}$. All the remaining fuel with mass $m_{f, 2, i}=4.3 \times 10^6 \mathrm{~kg}$ is burned during stage 2 with burn time of $360 \mathrm{~s}$. What is the change in speed in meters/second after stage two is complete?

证明 .

To calculate the change in speed after stage two, we can use the rocket equation, which relates the change in velocity of a rocket to the mass ratio of the rocket before and after the burn, and the effective exhaust velocity of the propellant.

The mass ratio of the rocket before and after stage two is:

$$\frac{m_{i}}{m_{f, 2}}=\frac{m_{r, i}+m_{f, i}+m}{m_{r, 2, d}}=\frac{2.81 \times 10^7 \mathrm{~kg}+2.46 \times 10^7 \mathrm{~kg}+1.4 \times 10^6 \mathrm{~kg}}{2.1 \times 10^6 \mathrm{~kg}}=22.95$$

where $m_{i}$ is the initial mass of the rocket (including all the fuel and the dry mass) and $m_{f,2}$ is the final mass of the rocket after stage two (including the dry mass and all the fuel burned in stage two).

The effective exhaust velocity $v_{e}$ is given by:

$$v_{e}=u \ln\frac{m_{i}}{m_{f,2}}=3000 \mathrm{~m/s} \ln\frac{2.81 \times 10^7 \mathrm{~kg}}{2.1 \times 10^6 \mathrm{~kg}+4.3 \times 10^6 \mathrm{~kg}}=2445.5 \mathrm{~m/s}$$

where $u$ is the speed of the ejected fuel relative to the rocket.

Now we can use the rocket equation:

$$\Delta v=v_{e} \ln\frac{m_{i}}{m_{f,2}}=2445.5 \mathrm{~m/s} \ln\frac{2.81 \times 10^7 \mathrm{~kg}}{2.1 \times 10^6 \mathrm{~kg}+4.3 \times 10^6 \mathrm{~kg}}=2434.4 \mathrm{~m/s}$$

Therefore, the change in speed after stage two is $\Delta v = 2434.4$ $\mathrm{m/s}$.

这是一份2023年的利物浦大学University of Liverpool NEWTONIAN MECHANICS MATH122代写的成功案例

计算物理学代写|INTRODUCTION TO COMPUTATIONAL PHYSICS PHYS105 University of Liverpool Assignment

0
Posted on by

Assignment-daixieTM为您提供利物浦大学University of Liverpool INTRODUCTION TO COMPUTATIONAL PHYSICS PHYS105计算物理学代写代考和辅导服务！

Instructions:

Computational physics is a subfield of physics that involves the use of computers to solve problems in physics that are too complex to be solved analytically. The use of computational techniques has become increasingly important in physics research and has led to many breakthroughs in our understanding of the physical world.

To get started with computational physics, it’s important to have a strong foundation in mathematics and programming. In terms of programming languages, Python is a popular choice for computational physics because of its ease of use and large user community.

There are many resources available online to help you learn computational physics. Some good places to start include:

  • The Open Source Physics Project: This project provides a collection of open-source software tools and simulations for teaching and researching physics. They have a range of resources and materials available for learning computational physics, including tutorials and sample code.
  • Coursera: Coursera offers a range of online courses in computational physics, including courses on Python programming and numerical methods for physics.
  • GitHub: GitHub is a platform for collaborative software development, and there are many open-source repositories available on GitHub that contain code for solving physics problems. You can use these repositories to learn from existing code and contribute to the development of new code.
  • Textbooks: There are many textbooks available on computational physics, including “Computational Physics” by Mark Newman and “An Introduction to Computational Physics” by Tao Pang.

I hope this information is helpful! Let me know if you have any other questions.

计算物理学代写|INTRODUCTION TO COMPUTATIONAL PHYSICS PHYS105 University of Liverpool Assignment

问题 1.

(a) Write a procedure that solves quadratic equations using the quadratic formula: It should take as arguments three numbers a, b, and c. It should print error messages if a is zero, or if the roots are complex. Otherwise it should print the two roots.

证明 . 

# a.) code for roots function
def roots(a, b, c):
    #form of equation is a*x**2 + b*x + c = 0
    #quadratic formula is   x = (-b + sqrt(b**2 - 4 * a * c))/(2 * a)
    #                   or  x = (-b - sqrt(b**2 - 4 * a * c))/(2 * a)
    #roots are complex when the discriminant (b**2 - 4*a*c) is negative
    discriminant = b**2 - (4 * a * c)
    if discriminant < 0:
        return "Roots are complex"
    return "x = "+str((-b + math.sqrt(discriminant)) / (2 * a))+" or x = "+\
        str((-b - math.sqrt(discriminant)) / (2 * a))

# Test Cases (assertions are just to automate)
##print '\nTesting roots'
##
##print roots(1, 2, 1) #(x+1)^2: double root at x = -1
##assert roots(1, 2, 1) == 'x = -1.0 or x = -1.0'
##
##print roots(1, -2, -3) #(x+1)(x-3): roots at x = 3 or x = -1
##assert roots(1, -2, -3) == 'x = 3.0 or x = -1.0'
##
##print roots(2, 2, 2) #2x^2 + 2x + 2: complex roots
##assert roots(2, 2, 2) == 'Roots are complex'

问题 2.

(b) Modify your procedure to handle the case of complex roots.

证明 . 

# b.) same code, modified to handle complex roots
def roots(a, b, c):
    discriminant = b**2 - (4 * a * c)
    if discriminant < 0:
        discriminant = discriminant + 0j
    return "x = "+str((-b + discriminant**0.5) / (2 * a))+ " or x = " +\
        str((-b - discriminant**0.5) / (2 * a)) # math.sqrt does not work

# Test Cases (assertions are just to automate)
##print '\nTesting roots'
##
##print roots(1, 2, 1) #(x+1)^2: double root at x = -1
##assert roots(1, 2, 1) == 'x = -1.0 or x = -1.0'
##
##print roots(1, -2, -3) #(x+1)(x-3): roots at x = 3 or x = -1
##assert roots(1, -2, -3) == 'x = 3.0 or x = -1.0'
##
##print roots(2, 2, 2) #2x^2 + 2x + 2: complex roots
##assert roots(2, 2, 2) == 'x = (-0.5+0.866025403784j) or x = (-0.5-0.866025403784j)'

###########################################
## 2.) procedure for evaluating polynomials
###########################################
def eval_poly(x, coeffs):
    total=0 #will keep a running total of the sum
    coeffs.reverse() # to put the low order coeffs first
    for i in range(len(coeffs)):
        total+=coeffs[i]*(x**i) #add the curent term to the total
    return total

# Test cases
##print '/nTesting Polynomial'
##
##print eval_poly(1,[1,2,3])
##assert eval_poly(1,[1,2,3]) == 6
##
##print eval_poly(2,[1,2,3,4])
##assert eval_poly(2,[1,2,3,4]) == 26

问题 3.

Write a procedure that evaluates polynomials. It should take two arguments. The first is a number $x$. The second is a list of of coefficients ordered from highest to lowest:
$$
a_n, a_{n-1}, \ldots, a_2, a_1, a_0
$$
Your procedure should return the value of the polynomial evaluated at $x$ :
$$
a_n x^n+a_{n-1} x^{n-1}+\ldots+a_2 x+a_1 x+a_0
$$

证明 . 

) procedure for evaluating polynomials
###########################################
def eval_poly(x, coeffs):
    total=0 #will keep a running total of the sum
    coeffs.reverse() # to put the low order coeffs first
    for i in range(len(coeffs)):
        total+=coeffs[i]*(x**i) #add the curent term to the total
    return total

# Test cases
##print '/nTesting Polynomial'
##
##print eval_poly(1,[1,2,3])
##assert eval_poly(1,[1,2,3]) == 6
##
##print eval_poly(2,[1,2,3,4])
##assert eval_poly(2,[1,2,3,4]) == 26

###########################################
## 3.) procedure to make change
###########################################
def make_change(cost, paid):
    change=paid-cost #calculate the change due
    bills={20:0,10:0,5:0,2:0,1:0} #dictionary that maps each bill to the amount of it
    bill_list=bills.keys() #bill_list is a list of the available bills
    bill_list.sort() #sort it in ascending order
    bill_list.reverse() #now reverse it to be in descending order
                        #this is to make sure you get the smallest number of bills
    for bill in bill_list: 
        while change >=bill: 
            bills[bill]+=1 #increment the amount of that bill
            change-=bill #decrease the change by that bill
            
    print "Change is:"
    for bill in bill_list:
        if bills[bill] == 1:
            print bills[bill], bill, "dollar bill"
        elif bills[bill] > 1:
            print bills[bill], bill, "dollar bills"

    #return bills #return the dictionary with amounts of each bill needed

make_change(1, 6)
make_change(4, 109)

这是一份2023年的利物浦大学University of Liverpool INTRODUCTION TO COMPUTATIONAL PHYSICS PHYS105代写的成功案例

天体物理学代写|ASTRONOMY 1 ASTRO1001 University of Glasgow AssignmentONLINE TUTOR

0
Posted on by

Assignment-daixieTM为您提供格拉斯哥大学University of Glasgow ASTRONOMY 1 ASTRO1001天体物理学代写代考和辅导服务！

Instructions:

Astronomy is the scientific study of celestial objects and phenomena beyond the Earth’s atmosphere, including planets, stars, galaxies, and other celestial bodies. It is a fascinating field that has captured the attention and imagination of people for thousands of years.

There are several subfields within astronomy, including solar system physics, positional astronomy, and dynamical astronomy.

Solar system physics is the study of the physical properties of the planets, moons, asteroids, and comets in our solar system. This includes their composition, structure, atmospheres, and orbits. Solar system physics also investigates the formation and evolution of the solar system, as well as the interactions between the various bodies.

Positional astronomy is concerned with measuring the positions, distances, and motions of celestial objects. This involves the use of telescopes, cameras, and other instruments to gather data on the positions of stars, galaxies, and other objects. Positional astronomy also includes the study of celestial coordinate systems, which are used to locate objects in the sky.

Dynamical astronomy is the study of the motions of celestial objects, including their orbits and gravitational interactions. This field includes the study of celestial mechanics, which deals with the mathematical description of the motions of objects in space. Dynamical astronomy also investigates the formation and evolution of galaxies, and the interactions between galaxies and other celestial bodies.

In conclusion, astronomy is a vast and complex field that encompasses many different areas of study. Solar system physics, positional astronomy, and dynamical astronomy are just a few of the many subfields within this fascinating discipline. The study of astronomy has helped us to understand the universe around us and has inspired us to ask even more questions about the nature of the cosmos.

天体物理学代写|ASTRONOMY 1 ASTRO1001 University of Glasgow Assignment

问题 1.

a. A globular cluster has $10^6$ stars each of apparent magnitude +8 . What is the combined apparent magnitude of the entire cluster?

证明 .

$\begin{gathered}+8=-2.5 \log \left(F / F_0\right) \ F=6.3 \times 10^{-4} F_0 \ F_{\text {cluster }}=10^6 \times 6.3 \times 10^{-4} F_0=630 F_0 \ m_{\text {cluster }}=-2.5 \log (630)=-7\end{gathered}$

问题 2.

b. Find the distance modulus to the Andromeda galaxy (M31). Take the distance to Andromeda to be $750 \mathrm{kpc}$.

证明 .

$\mathrm{DM}=5 \log \left(\frac{d}{10 \mathrm{pc}}\right)=5 \log (75,000)=24.4$

问题 3.

c. An eclipsing binary consists of two stars of different radii and effective temperatures. Star 1 has radius $R_1$ and $T_1$, and Star 2 has $R_2=0.5 R_1$ and $T_2=2 T_1$. Find the change in bolometric magnitude of the binary, $\Delta m_{\text {bol }}$, when the smaller star is behind the larger star. (Consider only bolometric magnitudes so you don’t have to worry about color differences.)

证明 .

$$
\begin{gathered}
\mathcal{F}{1 \& 2}=4 \pi \sigma\left(T_1^4 R_1^2+T_2^4 R_2^2\right) \ \mathcal{F}{\text {eclipse }}=4 \pi \sigma T_1^4 R_1^2 \
\Delta m=-2.5 \log \left(\frac{\mathcal{F}{1 \& 2}}{\mathcal{F}{\text {eclipse }}}\right) \
\Delta m=-2.5 \log \left(1+\frac{T_2^4 R_2^2}{T_1^4 R_1^2}\right) \
\Delta m=-2.5 \log \left(1+\frac{16}{4}\right)=-1.75
\end{gathered}
$$
So, the binary is 1.75 magnitudes brighter out of eclipse than when star 2 is behind star 1 .

这是一份2023年的格拉斯哥大学University of Glasgow天体物理学ASTRO1001代写的成功案例